The question aims to find the standard form of an algebraic equation. The question is based on the concepts of algebraic equations, particularly linear equations with two variables. Linear equations are algebraic equations with variables only having an exponent of one. These equations represent a linear straight line as shown in Figure 1. The equation of the line is given as:
\[ Ax + By = C \]
Here A, B, and C are constants, and x and y are two variables. If we solve this equation for variable y, then A/B will represent the slope of the equation, and C/B will give us the y-intercept of the line represented by this equation.
Expert Answer
The given algebraic linear equation is:
\[ y = 2x\ -\ 9 \]
Figure 1 below shows the graph of the equation for $0 \leq x \leq 5$.
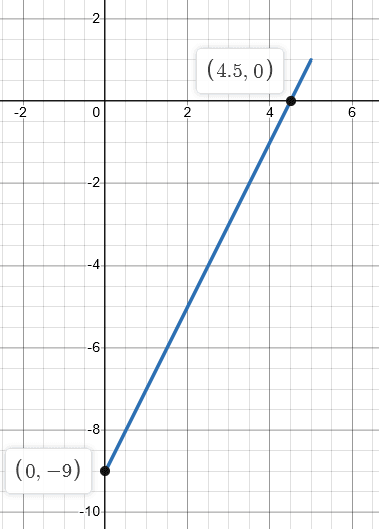
Figure 1
Figure 1 shows the graph of the given equation, which has a slope of 2, and the y-intercept is -9, as shown in the Figure above.
The standard form of the equation is given as:
\[ Ax + By = C \]
To make the given linear equation in standard form, we can perform the following operations.
\[ y = 2x\ -\ 9 \]
Step 1: Subtract y from both sides.
\[ y\ -\ y = 2x\ -\ 9\ -\ y \]
\[ 0 = 2x\ -\ 9\ -\ y \]
Step 2: Add 9 on both sides.
\[ 0 + 9 = 2x\ -\ 9\ -\ y + 9 \]
\[ 9 = 2x\ -\ y \]
Rearranging the equation to represent in standard form.
\[ 2x\ -\ y = 9 \]
When this equation is used to plot the graph, we will get the same line shown above in Figure 1, as these two equations are exactly the same.
Numerical Result
The standard form of the given equation y = 2x – 9 is calculated to be:
\[ 2x\ -\ y = 9 \]
Example
How do you write the algebraic equation y = x – 6 in standard form?
\[ y = x\ -\ 6 \]
Figure 2 below shows the graph of the equation for $0 \leq x \leq 5$.

Figure 2
The given equation has a slope of 1, as can be observed from the graph, and the y-intercept is -6.
The standard form of the equation is given as:
\[ Ax + By = C \]
To make the given linear equation in standard form, we can perform the following operations.
\[ y = x\ -\ 6 \]
Step 1: Subtract y from both sides.
\[ y\ -\ y = x\ -\ 6\ -\ y \]
\[ 0 = x\ -\ 6\ -\ y \]
Step 2: Add 6 on both sides.
\[ 0 + 6 = 2x\ -\ 6\ -\ y + 6 \]
\[ 6 = x\ -\ y \]
Rearranging the equation to represent in standard form.
\[ x\ -\ y = 6 \]
When this equation is used to plot the graph, we will get the same line shown above in Figure 2, as these two equations are exactly the same.
Images/Mathematical drawings are created with Geogebra.
