Synthetic Division – Explanation & Examples
A polynomial is an algebraic expression made up of two or more terms subtracted, added, or multiplied. A polynomial can contain coefficients, variables, exponents, constants, and operators such as addition and subtraction.
It is also important to note that, a polynomial can’t have fractional or negative exponents. Examples of polynomials are; 3y2 + 2x + 5, x3 + 2 x 2 − 9 x – 4, 10 x 3 + 5 x + y, 4x2 – 5x + 7) etc. Like number, polynomials can undergo addition, subtraction, multiplication, and division.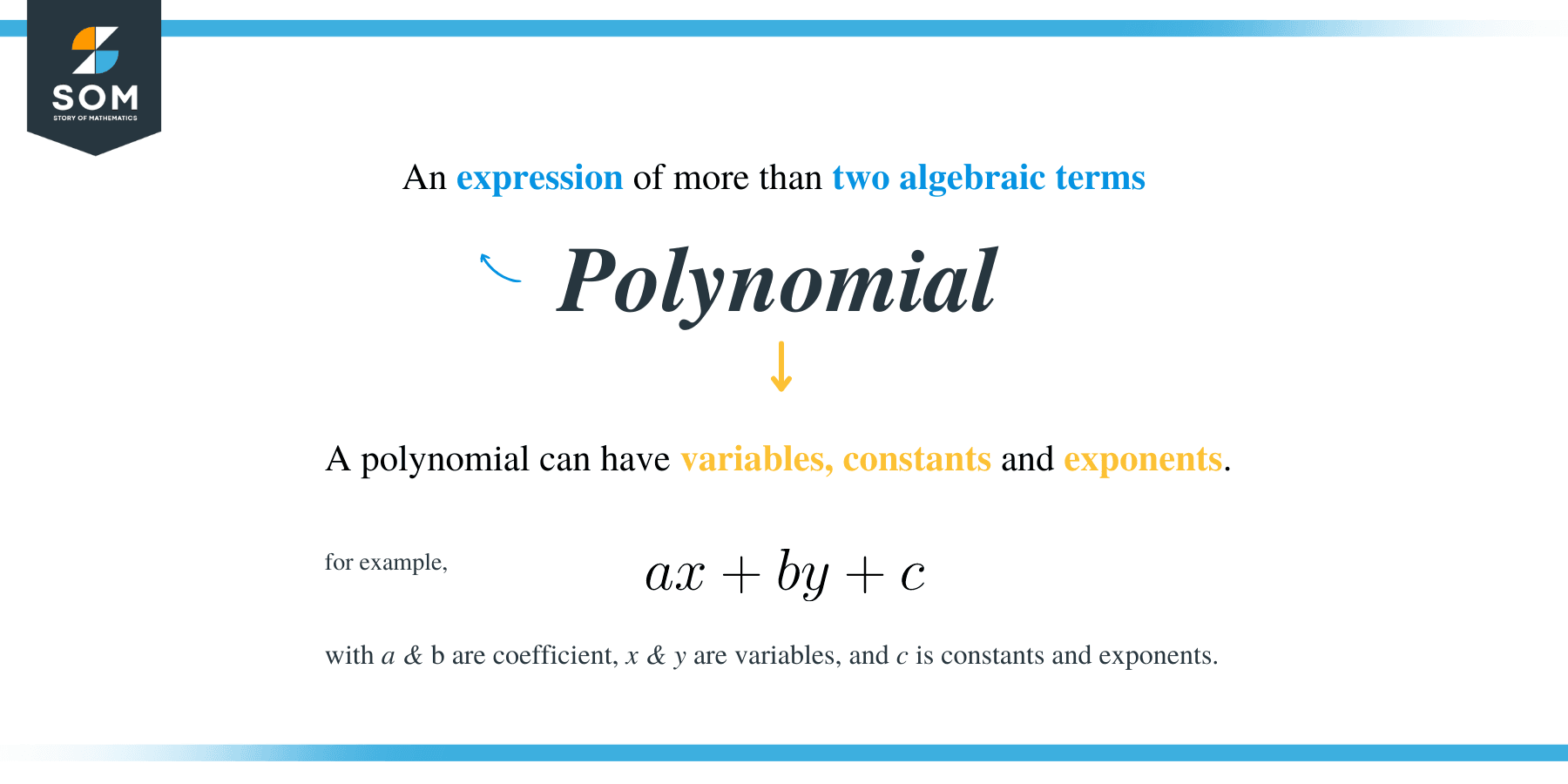
We saw addition, subtraction, multiplication, and long division of polynomials previously. Let’s have a look at synthetic division now.
There are two methods in mathematics for dividing polynomials.
These are the long division and the synthetic method. As the name suggests, the long division method is the most cumbersome and intimidating process to master. On the other hand, the synthetic method is a “fun” way of dividing polynomials.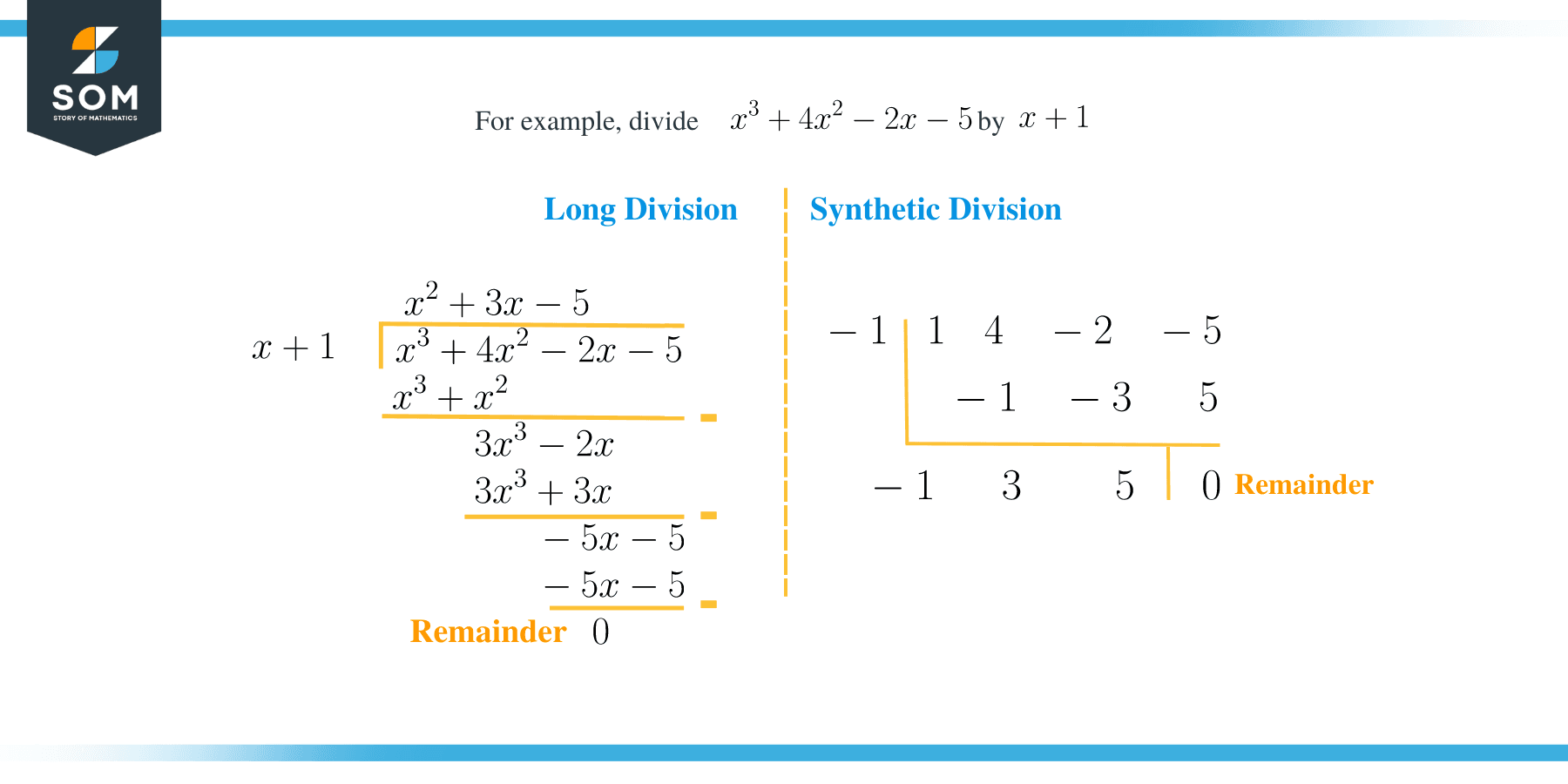
I must say that synthetic division is a shortcut way to divide polynomials because it entails fewer steps to arrive at the answer than the polynomial long division method. This article will discuss the synthetic division method and how to do the method with a couple of examples.
What is Synthetic Division?
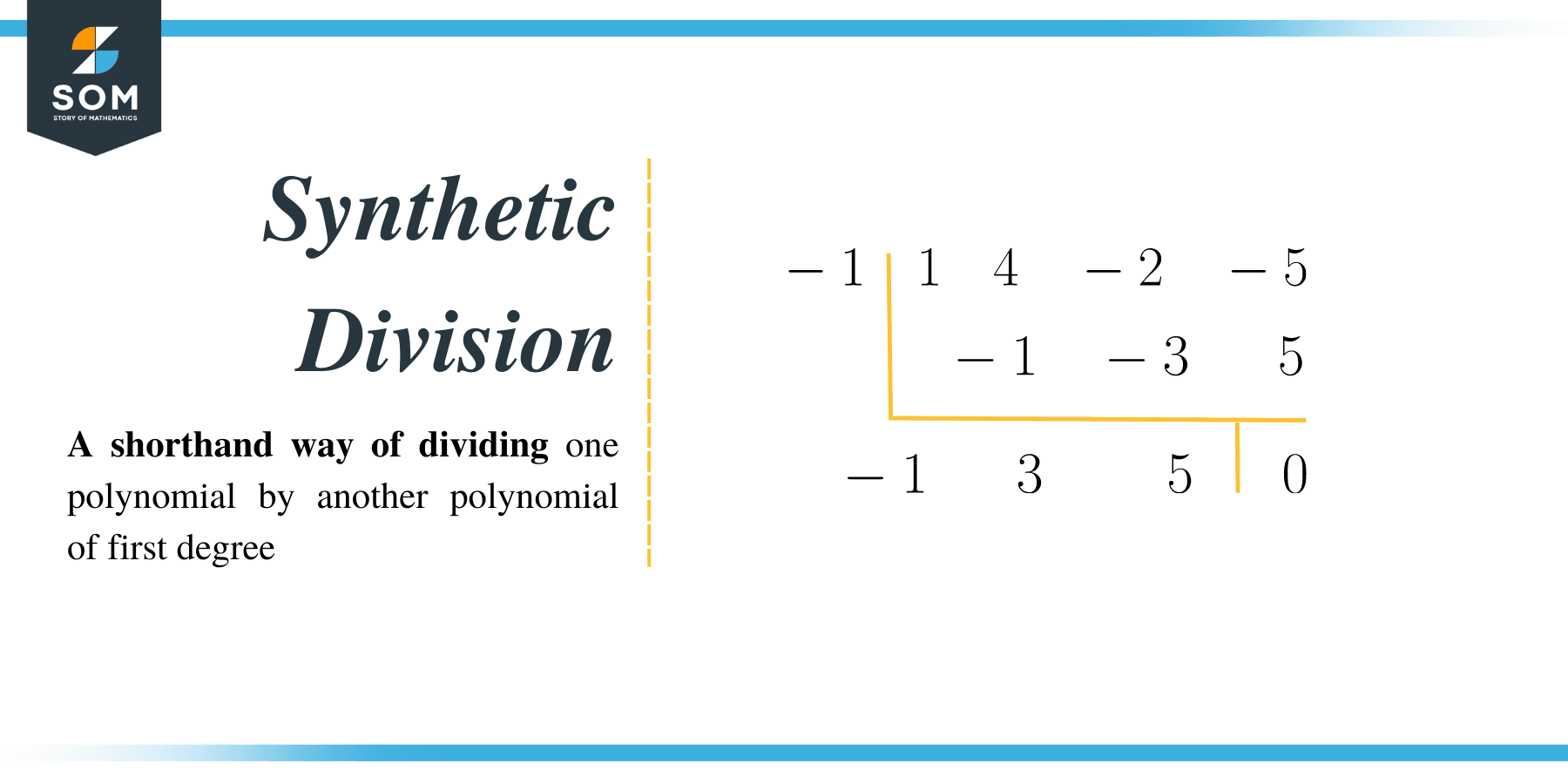Synthetic division can be defined as a shorthand way of dividing one polynomial by another polynomial of first degree. The synthetic method involves finding zeroes of the polynomials.
How to do Synthetic Division?
To divide a polynomial using synthetic division, you should divide it with a linear expression whose leading coefficient must be 1.
This type of division by a linear denominator is commonly known as division by Ruffini’s rule or the “paper-and-pencil computation.”
For the synthetic division method to be possible, the following requirements must be meet:
- The divisor should be a linear factor. This means that the divisor should be an expression of degree 1.
- The leading coefficient of the divisor should also be 1. If the divisor’s coefficient is other than 1, the synthetic division process will get messed up. Therefore, you will be forced to manipulate the divisor to convert the leading coefficient to 1. For example, 4x – 1 and 4x + 9 would be x – ¼ and x + 9/4 respectively.
To perform polynomial synthetic division, here are the steps:
- Set the divisor to zero to find the number to put in the division box.
- Express the dividend in standard form. This is the same as writing the dividend in descending order. If the dividend is missing some terms, fill them using zero. For example, 3x4 + 2 x3 + 3x2 + 5 = 3x4 + 2 x3 + 3x2 + 0x +5
- Now, bring down the leading coefficient in the dividend.
- Place the product of the number you brought down and the number in the division box in the preceding column.
- Write the result at the bottom of the row by adding the product from step 4 and the preceding number.
- Repeat procedure 5 until the remainder is zero or a numerical value.
- Write your final answer as the numbers in the bottom column. When there is a remainder in the division box, express it as a fraction with its denominator.
NOTE: The variable in the answer is one power less than the original dividend
You can master the above steps by using the following mantra: “Bring down, Multiply and add, multiply and add, Multiply and add, ….”
Example 1
Divide x3 + 5x2 -2x – 24 by x – 2
Solution
Change the sign of constant in the divisor x -2 from -2 to 2 and drop it down.
_____________________
x – 2 | x ³ + 5x² – 2x – 24
2 | 1 5 -2 -24
Also, bring down the leading coefficient. This means that 1 be the first number of the quotient.
2 | 1 5 -2 -24
________________________
1
Multiply 2 by 1 and add 5 to the product to get 7. Now bring 7 down.
2 | 1 5 -2 -24
2
________________________
1 7
Multiply 2 by 7 and add – 2 to the product to get 12. Bring 12 down
2 | 1 5 -2 -24
2 14
__________________________
1 7 12
Finally, multiply 2 by 12 and add -24 to the result to get 0.
2 | 1 5 -2 -24
2 14 24
__________________________
1 7 12 0
Hence;
x3 + 5x2 -2x – 24/ x – 2 = x² + 7x + 12
Example 2
Divide x2 + 11x + 30 by x + 5
Solution
Change the sign of constant in the divisor x + 5 from 5 to -5 and bring it down.
_____________________
x + 5 | x2 + 11x + 30
-5 | 1 11 30
Bring down the coefficient of the first term in the dividend. This will be our first quotient
2 | 1 11 30
________________________
1
Multiply -5 by 1 and add 11 to the product to get 6. Bring 6 down;
-5 | 1 11 30
-5
________________________
1 6
Multiply -5 by 6 and add 30 to the result to get 0.
-5 | 1 11 30
-5 -30
________________________
1 6 0
Therefore, the quotient is x + 6
Example 3
Divide 2x3 + 5x2 + 9 by x + 3
Solution
Reverse the sign of constant in the divisor x + 3 from 3 to -3 and bring it down.
_____________________
x + 3 | 2x3 + 5x2 + 0x + 9
-3| 2 5 0 9
Bring down the coefficient of the first term in the dividend. This will be our first quotient.
-3 | 2 5 0 9
________________________
2
Multiply -3 by 2 and add 5 to the product to get -1. Bring -1 down;
-3 | 2 5 0 9
-6
________________________
2 -1
Multiply -3 by -1 and add 0 to the result to get 3. Bring 3 down.
-3 | 2 5 0 9
-6 3
________________________
2 -1 3
Multiply -3 by 3 and add -9 to the result to get 0.
-3 | 2 5 0 9
-6 3 -9
________________________
2 -1 3 0
Therefore, 2x2– x + 3 is the correct answer.
Example 4
Use synthetic division to divide 3x3 + 10x2 − 6x −20 by x+2.
Solution
Reverse the sign of x + 2 from 2 to -2 and bring it down.
_____________________
x + 2 |4x3 + 10x2 − 6x − 20
-2| 4 10 6 20
Bring down the coefficient of the first term in dividend.
-2 | 4 10 6 20
________________________
4
Multiply -2 by 4 and add 10 to get 2. Bring 2 down;
-2 | 4 10 6 20
-8
________________________
4 2
Multiply -2 by 2 and add -6 to the result to get 10. Bring -10 down.
-2 | 4 10 -6 20
-8 -4
________________________
4 2 10
Multiply -2 by 10 and add 20 to the result to get 0.
-2 | 4 10 -6 20
-8 -4 -20
________________________
4 2 -10 0
Therefore, 4x2 + 2x −10 is the answer.
Example 5
Divide -9x4 +10x3 + 7x2 − 6 by x−1.
Solution
-9x4 +10x3 + 7x2 − 6 / x−1 =
1 | -9 10 7 0 -6
-8 1 8 8
________________________
-9 8 8 2
Therefore, the answer is -9x3 +8x2+8x + 2/x -1
