JUMP TO TOPIC
Subtracting Exponents – Explanation & Examples
 Exponents are powers or indices. An exponential expression consists of two parts, namely the base, denoted as b and the exponent, denoted as n. The general form of an exponential expression is b n.
Exponents are powers or indices. An exponential expression consists of two parts, namely the base, denoted as b and the exponent, denoted as n. The general form of an exponential expression is b n.
How to Subtract Exponents?
The operation of subtracting exponents is quite easy if you have a good understanding of exponents. In this article, you will learn the rules and how to apply them when you need to subtract with exponents.
But before we can embark on subtracting with exponents, let us remind ourselves some of the basic terms about exponents.
What is an exponent?
Well, an exponent or power denotes the number of times a number is repeatedly multiplied by itself. For example, when we encounter a number written as, 53, it simply implies that 5 is multiplied by itself three times. In other words, 53 = 5 x 5 x 5 = 125
The same format of writing exponents applies with variables. Variables are represented by letters and symbols. For instance, when x is multiplied repeated by itself 3 times, then we write this as; x3. Variables are usually accompanied by coefficients. A coefficient is therefore an integer that is multiplied by variable.
For instance, in 2x3, the coefficient is the number 2 and x is the variable. When a variable has no number before it, the coefficient is always 1. This is also true when a number has no exponent. The coefficient of 1 is normally negligible, and therefore cannot be written with a variable.
Subtraction of exponents really does not involve any a rule. If a number is raised to a power. You simply compute the result and then perform the normal subtraction. If both the exponents and the bases are the same, you can subtract them like any other like terms in algebra. For example, 3y – 2xy = x y.
Subtracting exponents with the same base
Let’s explain this concept with the help of a few examples.
Example 1
- 23– 22 = 8 – 4 = 4
- 53 – 52 = 75 – 25 = 50
- Subtract x 3 y 3 from 10 x 3 y 3
In this case the coefficients of exponents are 10 and 1
The variables are like terms and hence can be subtracted
Subtract the coefficients = 10 – 1
= 9
Thus, 10x 3y 3– x 3y 3 = 9 (xy)3
You can notice that, the subtraction of exponents with like terms is done by finding the difference of their coefficients.
- Subtract 8x2 – 4x2
In this case, the variables 4x2 and 8x2 are like terms and their coefficients are 4 and 8 respectively.
= 8x2 – 4x2
= (8-4) x2.
= 4 x2
- Work out (-7x) – (-3x)
Here, -7x and -3x are like terms
= -7x – (-3x)
= -7x + 3x,
= -4x.
- 15x – 4x – 12y – 3y
Subtract like terms
15x – 4x = 11x
12y – 3y = 9y
Thus, the answer is 11x – 9y.
- Subtract (4x + 3y + z) – (2x + 3y – z).
These variables are like terms
(2x + 3y – z) – (4x + 3y + z)
Open the parenthesis;
= 2x + 3y – z – 4x – 3y – z,
Rearrange the like terms, and perform the subtraction
= 2x – 4x + 3y – 3y – z – z
= -2x + 0 – 2z,
= -2x – 2z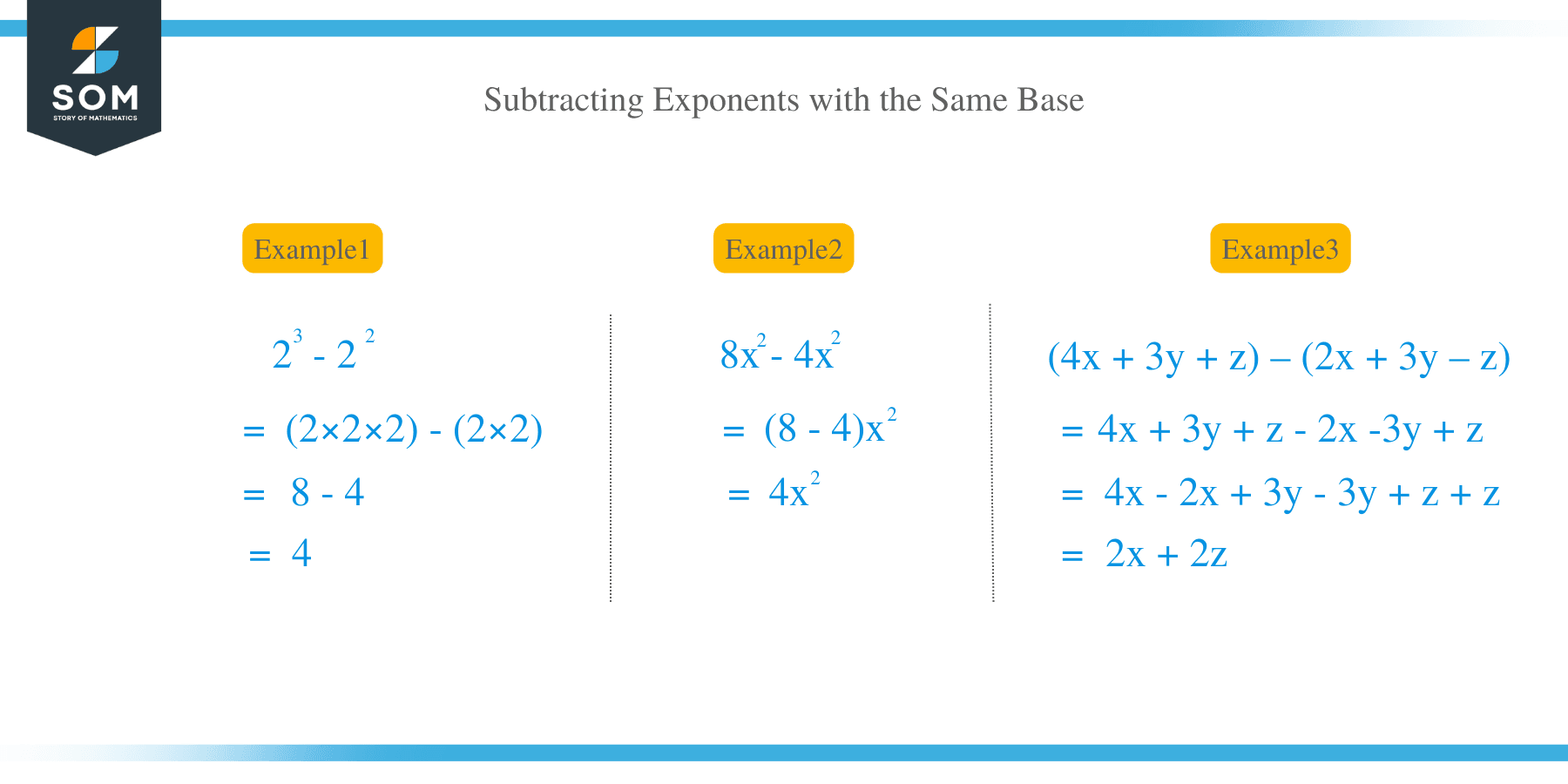
Subtracting exponents with different base
Exponents with different bases are computed separated and the results subtracted. On the other hand, variable with unlike bases can not be subtracted at all. For, example subtraction of a and b can not be performed and the result is just a -b.
To subtract a positive exponents m and negative exponents n, we just connect both the terms by changing the subtraction sign to a positive sign and write the result in the form of m + n.
Therefore, subtraction of a positive and a negative unlike exponents m and -n = m + n.
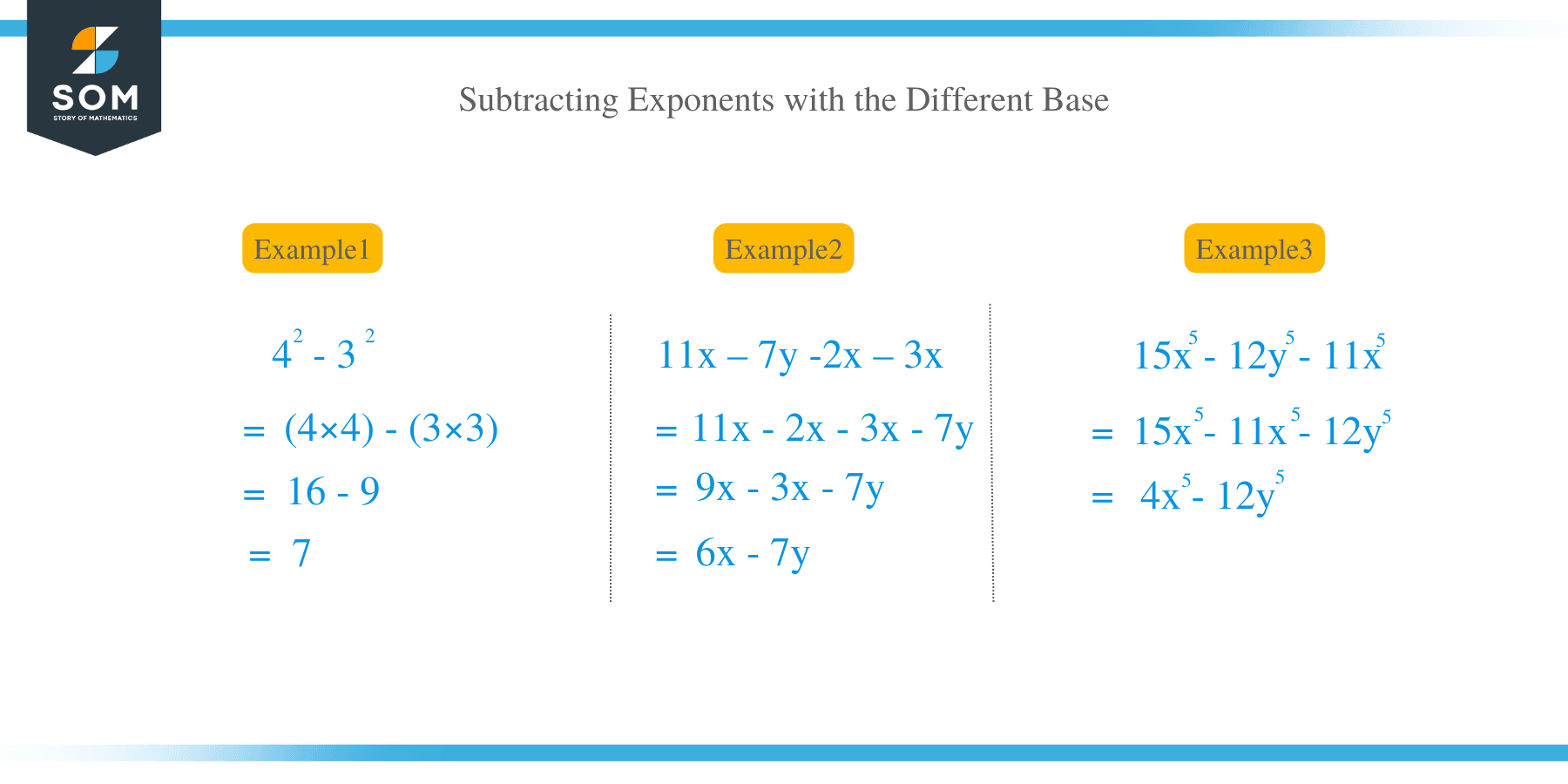
Example 2
- 42 – 32 = 16 – 9 =7
- Subtract: 11x – 7y -2x – 3x.
= 11x – 2x – 3x – 7y.
= 6x – 7y - Evaluate 3x2 – 7y2
In this case, the two exponents 3x 2 and 7y2 are unlike terms and so it will remain as it is.
Here 3x and 7y both are unlike terms so it will remain as it is.
Therefore, the answer is 3x2 – 7y2 - Evaluate 15x – 12y – 11x
= 15x5 – 11x5 – 12y5
= 4x5 – 12y5