Solving Two-Step Equations – Techniques & Examples
What is a Two-step Equation?
It is probably undebatable that a two-step equation is as easy as ABC. As the name suggests, a two-step equation is an algebraic equation that requires only two steps to be completely solved.
The equation is already solved when the value of the variable is found. In this article, we will take you step by step in solving two-step equations to make you familiar and proficient with the process.
Generally, when solving an equation, we apply the Law of Equations, which states that whatever is to be performed on the right-hand side (RHS) of an equation should also be done to the left-hand side (LHS) of the equation so that the equation can remain balanced.
A two-step equation has been solved if a variable, usually represented by an alphabetical letter, is isolated on either the equation’s left or right side. The number is located on the opposite side.
How to Solve Two-step Equations?
Solving a two-step equation involves working backward concerning the order of operations (PEMDAS). In this case, multiplication and division are preceded by addition and subtraction.
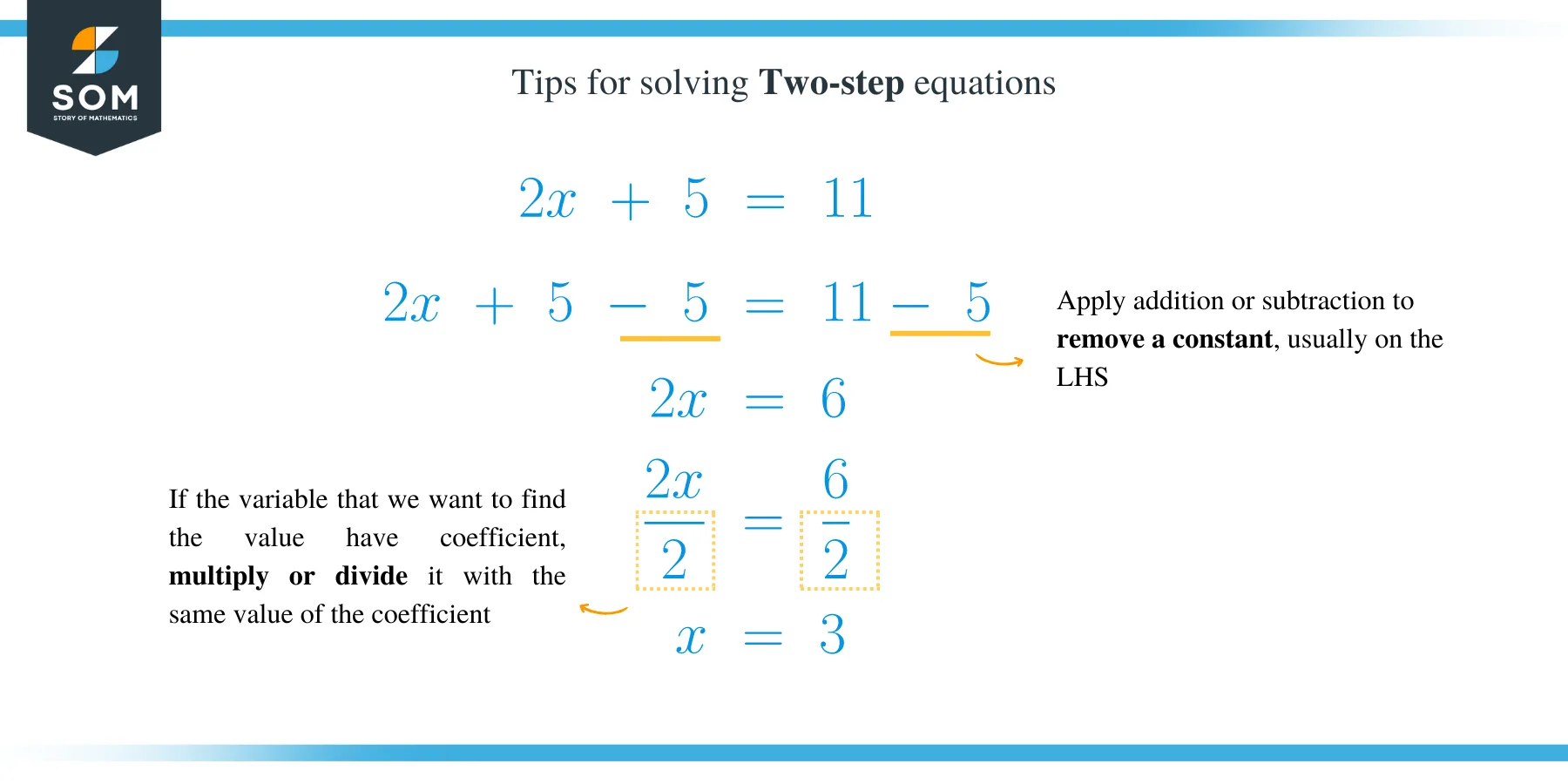
Tips for Solving Two-step equations include:
- Always apply addition or subtraction to remove a constant.
- Apply multiplication or division to remove any coefficient from a variable.
Example 1
Solve the two-step equation y:
3y – 2 = 13
Solution
Add 2 to both sides of the equation and divide by 3.
3y – 2 + 2 = 13 + 2
3y = 15
3y/3 = 15/3
y = 5
Example 2
Solve the two-step equation for z.
2z +15 = −3z
Solution
Subtract 2z from both sides of the equation and divide by -5.
2z – 2z + 15 = -3z – 2z
15 = -5z
15/-5 = -5z/-5
z = 3
Example 3
Solve the two-step equation for x
(x/5) -6 = -8
Solution
Add both 6 to both sides of the equation and multiply by 5.
(x/5) – 6 + 6 = – 8 + 6
(x/5)5 = – 2 x 5
x = -10
Example 4
Solve the two-step equation for k.
(k + 5)/2 = 8
Solution
Multiply 2 on both sides of the equation then, subtract 5 from both sides as well.
2 x (k + 5)/2 = 8 x 2
k + 5-5 = 16 -5
k = 11
Example 5
Solve the two-step equation for y.
5y/4 + 2y/3 = 5
Solution
Multiply each term of the equation by the LCD.
The LCD = 12
(5y/4)12 + (2y/3)12 = 5 x 12
15y + 8y = 60
23y = 60
23y/23 = 60/23
y = 60/23
Example 6
Solve the equation for x in the following two-step equation.
4.25 – 0.25x = 3.75
Solution
Subtract 4.25 from both sides and divide by – 0.25
4.25 – 4.25- 0.25x = 3.75 – 4.25
– 0.25x = – 0.5
-0.25x/-0.25 = – 0.5/- 0.25
X = 2
Example 7
Solve for x in the two-step equation 5x − 6 = 9
Solution
Add 6 to both sides.
5x – 6 + 6 = 9 + 6
5x = 15
Divide both sides by.
5 x /5 = 15/5
x = 3
Example 8
Solve for x in the equation -2x – 3 = 4x – 15.
Solution
Adding +3 to the left and right side of the equation will give;
(-2x – 3) +3 = (4x – 15) +3 = -2x = 4x – 12
Subtract -4x from both sides of the equation.
-2x – 4x = (4x – 12) – 4x = -6x = -12
Divide both sides of the equation by -6.
-6x ÷ -6 = -12 ÷ -6
x = 2
Example 9
Solve for x in the two-step equation:4x + 7 – 6 = 5 – 4x + 4
Solution
First, simplify both sides of the equation by combining like terms.
4x + 1 = 9 – 4x.
Add 4x and subtract 1 from both sides of the equation.
8x = 8.
Divide both sides of the equation by 8.
8x /8 = 8/8
x = 1
Example 10
Solve for x in the following two step equation:
11 = 3 – 7x.
Solution
In this case, we can still isolate the variable x to the right side of the equation.
Subtract 3 from both sides of the equation.
=> 11 – 3 = 3 – 3 – 7x
8 = – 7x
Divide both sides of the equation by -7 to isolate for x.
=> 8/-7 = -7/7x
x = -1.14
