Solving Multi-Step Equations – Methods & Examples
To understand how to solve multi-step equations, one must have a strong foundation for solving one-step and two-step equations. And for this reason, let’s take a brief review of what one-step and two-step equations entail.
One step equation is an equation that requires only one step to be solved. You only perform a single operation in order to solve or isolate a variable. Examples of one step equations include: 5 + x = 12, x – 3 = 10, 4 + x = -10 etc.
- For instance, to solve 5 + x = 12,
You only need to subtract 5 from both sides of the equation:
5 + x = 12 => 5 – 5 + x = 12 – 5
=> x = 7
- 3x = 12
To solve this equation, divide both sides of the equation by 3.
x = 4
You can note that for a one-step equation to be completely solved, you only need a single step: add/subtract or multiply/divide.
A two-step equation, on the other hand, requires two operations to perform to solve or isolate a variable. In this case, the operations to solve a two-step are addition or subtraction and multiplication or division. Examples of two-step equations are:
- (x/5) – 6 = -8
Solution
Add both 6 to both sides of the equation and multiply by 5.
(x/5) – 6 + 6 = – 8 + 6
(x/5)5 = – 2 x 5
x = -10
- 3y – 2 = 13
Solution
Add 2 to both sides of the equation and divide by 3.
3y – 2 + 2 = 13 + 2
3y = 15
3y/3 = 15/3
y = 5
- 3x + 4 = 16.
Solution
To solve this equation, subtract 4 from both sides of the equation,
3x + 4 – 4 = 16 – 4.
This gives you the one-step equation 3x = 12. Divide both sides of the equation by 3,
3x/3 = 12/3
x = 4
What is a Multi-step Equation?
The term “multi” means many or more than two. Therefore, a multi-step equation can be defined as an algebraic expression that requires several operations such as addition, subtraction, division, and exponentiation to be solved. Multi-step equations are solved by applying similar techniques used in solving one-step and two-step equations.
Like we saw in one-step and two-step equations, the main objective of solving multi-step equations is to isolate the unknown variable on either the RHS or LHS of the equation while keeping a constant term on the opposite side. The strategy of obtaining a variable with a coefficient of one entails several processes.
The Law of equations is the most important rule you should remember while solving any linear equation. This implies that, whatever you do to one side of the equation, you MUST do to the opposite of the equation.
For instance, if you add or subtract a number on one side of the equation, you must also add or subtract on the equation’s opposite side.
How to Solve Multi-step Equations?
A variable in an equation can be isolated on any side, depending on your preference. However, keeping a variable on the left side of the equation makes more sense because an equation is always read from left to right.
When solving algebraic expressions, you should keep in mind that a variable doesn’t need to be x. Algebraic equations make use of any available alphabetical letter.
In summary, to solve multi-step equations, the following procedures are to be followed:
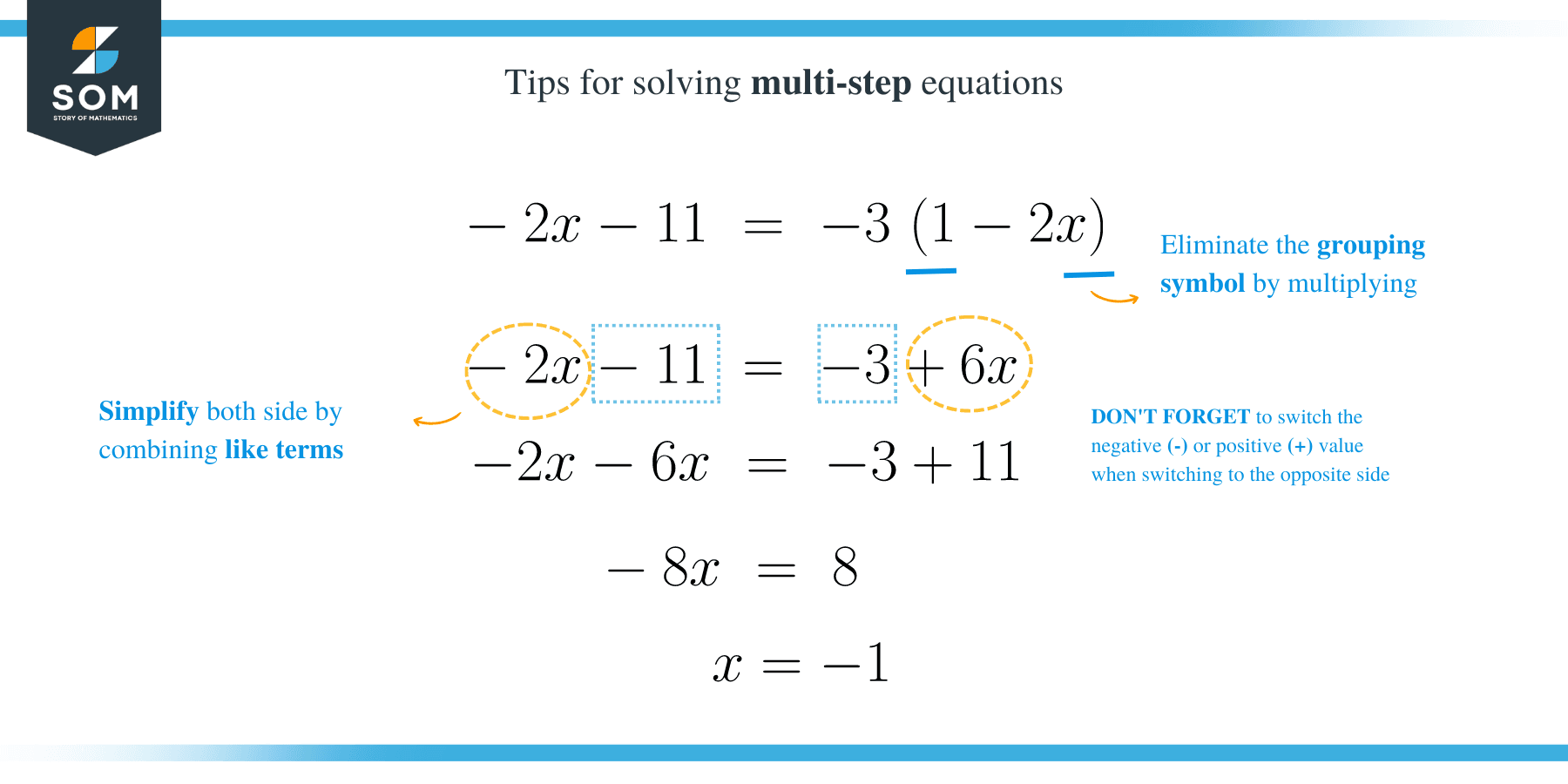
- Eliminate any grouping symbols such as parentheses, braces, and brackets by employing the distributive property of multiplication over addition.
- Simplify both sides of the equation by combining like terms.
- Isolate a variable on any side of the equation depending on your preference.
- A variable is isolated, performing the two opposite operations, such as addition and subtraction. Addition and subtraction are the opposite operations of multiplication and division.
Examples of How to Solve Multi-Step Equations
Example 1
Solve the multi-step equation below.
12x + 3 = 4x + 15
Solution
This is a typical multi-step equation where variables are on both sides. This equation has no grouping symbol and like terms to combine on opposite sides. Now, to solve this equation, first decide where to keep the variable. Since 12x on the left side is greater than 4x on the right side, therefore we keep our variable to the LHS of the equation.
This implies that, we subtract by 4x from both sides of the equation
12x – 4x + 3 = 4x – 4x + 15
6x + 3 = 15
Also subtract both sides by 3.
6x + 3 – 3 = 15 – 3
6x = 12
The last step now is to isolate x by dividing both sides by 6.
6x/6 = 12/6
x = 2
And there, we are done!
Example 2
Solve for x in the multi-step equation below.
-3x – 32 = -2(5 – 4x)
Solution
- The first step is to remove the parenthesis by use of the Distributive Property of Multiplication.
-3x – 32 = -2(5 – 4x) = -3x – 32 = – 10 + 8x
- In this example, we have decided keep the variable on the left side.
- adding both sides by 3x gives; -3x + 3x – 32 = – 10 + 8x + 3x =>
– 10 + 11x = -32
- Add both sides of the equation by 10 to clear -10.
– 10 + 10 + 11x = -32 + 10
11x = -22
- Isolate the variable x by dividing both sides of the equation by 11.
11x/11 = -22/11
x = -2
Example 3
Solve the multi-steps equation 2(y −5) = 4y + 30.
Solution
- Remove the parentheses by distributing the number outside.
= 2y -10 = 4y + 30
- By keeping the variable to the right side, subtract 2y from both sides of the equation.
2y – 2y – 10 = 4y – 2y + 23
-10 = 2y + 30
- Next, subtract both of the sides of the equation by 30.
-10 – 30 = 2y + 30 – 30
– 40 = 2y
- Now divide both sides by the coefficient of 2y to get the value of y.
-40/2 = 2y/2
y = -20
Example 4
Solve the multi-steps equation below.
8x -12x -9 = 10x – 4x + 31
Solution
- Simplify the equation by combining like terms on both sides.
– 4x – 9 = 6x +31
- Subtract on both sides of the equation by 6x to keep the variable x to the equation’s left side.
– 4x -6x – 9= 6x -6x + 31
-10x – 9 = 31
- Add 9 to both sides of the equation.
– 10x -9 + 9 = 31 +9
-10x = 40
- Finally, divide both sides by -10 to get the solution.
-10x/-10 = 40/-10
x = – 4
Example 5
Solve for x in the multi-step equation 10x – 6x + 17 = 27 – 9
Solution
Combine the like terms on both sides of the equation
4x + 17 = 18
Subtract 17 from both sides.
4x + 17 – 17 = 18 -17
4x = 1
Isolate x by dividing both sides by 4.
4x/4 = 1/4
x = 1/4
Example 6
Solve for x in the multi-step equation below.
-3x – 4(4x – 8) = 3(- 8x – 1)
Solution
The first step is to remove the parentheses by multiplying numbers outside the parentheses by terms within the parentheses.
-3x -16x + 32 = -24x – 3
Perform a bit of housecleaning by collecting like terms on both sides of the equation.
-19x + 32 = -24x – 3
Let’s keep our variable to the left by adding 24x to both sides of the equation.
-19 + 24x + 32 = -24x + 24x – 3
5x + 32 = 3
Now move all constants to right side by subtracting by 32.
5x + 32 -32 = -3 -32
5x = -35
The last step is to divide both sides of the equation by 5 to isolate x.
5x/5 = – 35/5
x = -7
Example 7
Solve for t in the multi steps equation below.
4(2t – 10) – 10 = 11 – 8(t/2 – 6)
Solution
Apply the distributive property of multiplication to eliminate the parentheses.
8t -40 – 10 = 11 -4t – 48
Combine the like terms on both sides of the equation.
8t -50 = -37 – 4t
Let’s keep the variable on the left side by adding 4t to both sides of the equation.
8t + 4t – 50 = -37 – 4t + 4t
12t – 50 = -37
Now add 50 to both sides of the equation.
12t – 50 + 50 = – 37 + 50
12t = 13
Divide both sides by 12 to isolate t.
12t/12 = 13/12
t = 13/12
Example 8
Solve for w in the following multi steps equation.
-12w -5 -9 + 4w = 8w – 13w + 15 – 8
Solution
Combine the like term and constants of both sides of the equation.
-8w – 14= -5w + 7
To keep the variable on the left side, we add 5w on both sides.
-8w + 5w – 14 = -5w + 5w + 7
-3w – 14 = 7
Now add 14 to both sides of the equation.
– 3w – 14 + 14 = 7 + 14
-3w = 21
The final step is to divide both sides of the equation by -3
-3w/-3 = 21/3
w = 7.
