Solving Equations – Techniques & Examples

Understanding how to solve equations is one of the most fundamental skills each student studying algebra can master. The solutions for most of the algebraic expressions are sought by applying this skill. Therefore, students need to become more proficient in how to go about the operation.

This article will learn how to solve an equation by performing the four basic mathematical operations: addition, subtraction, multiplication, and division.

An equation is generally composed of two expressions separated by a sign that indicates their relationship. Expressions in an equation can be related by equals to sign (=), less than (<), greater than (>), or a combination of these signs.

How to Solve Equations?

Solving an algebraic equation is generally the procedure of manipulating an equation. The variable is left on one side, and everything else is on the other side of the equation.

In simple words, to solve an equation is to isolate by making its coefficient equal to 1. Whatever you do to one side of an equation, do the same to the opposite side of the equation.

Solve equations by adding

Let’s see a few examples below to understand this concept.

Example 1

Solve: –7 – x = 9

Solution

–7 – x = 9

Add 7 to both sides of the equation.
7 – x + 7 = 9 + 7
– x = 16

Multiply both sides by –1
x = –16

Example 2

Solve 4 = x – 3

Solution

Here, the variable is on the RHS of the equation. Add 3 to both sides of the equation

4+ 3 = x – 3 + 3

7 = x

Check for the solution by substituting the answer into the original equation.

4 = x – 3

4 = 7 – 3

Therefore x = 7 is the correct answer.

Solving equations by subtracting

Let’s see a few examples below to understand this concept.

Example 3

Solve for x in x + 10 = 16

Solution

x + 10 = 16

Subtract 7 from both sides of the equation.

x + 10 – 10 = 16 – 10

x = 6

Example 4

Solve the linear equation 15 = 26 – y

Solution

15 = 26 – y

Subtract 26 from both sides of the equation
15 -26 = 26 – 26 -y
– 11 = -y

Multiply both sides by –1

y = 11

Solving equations with variables on both sides by adding

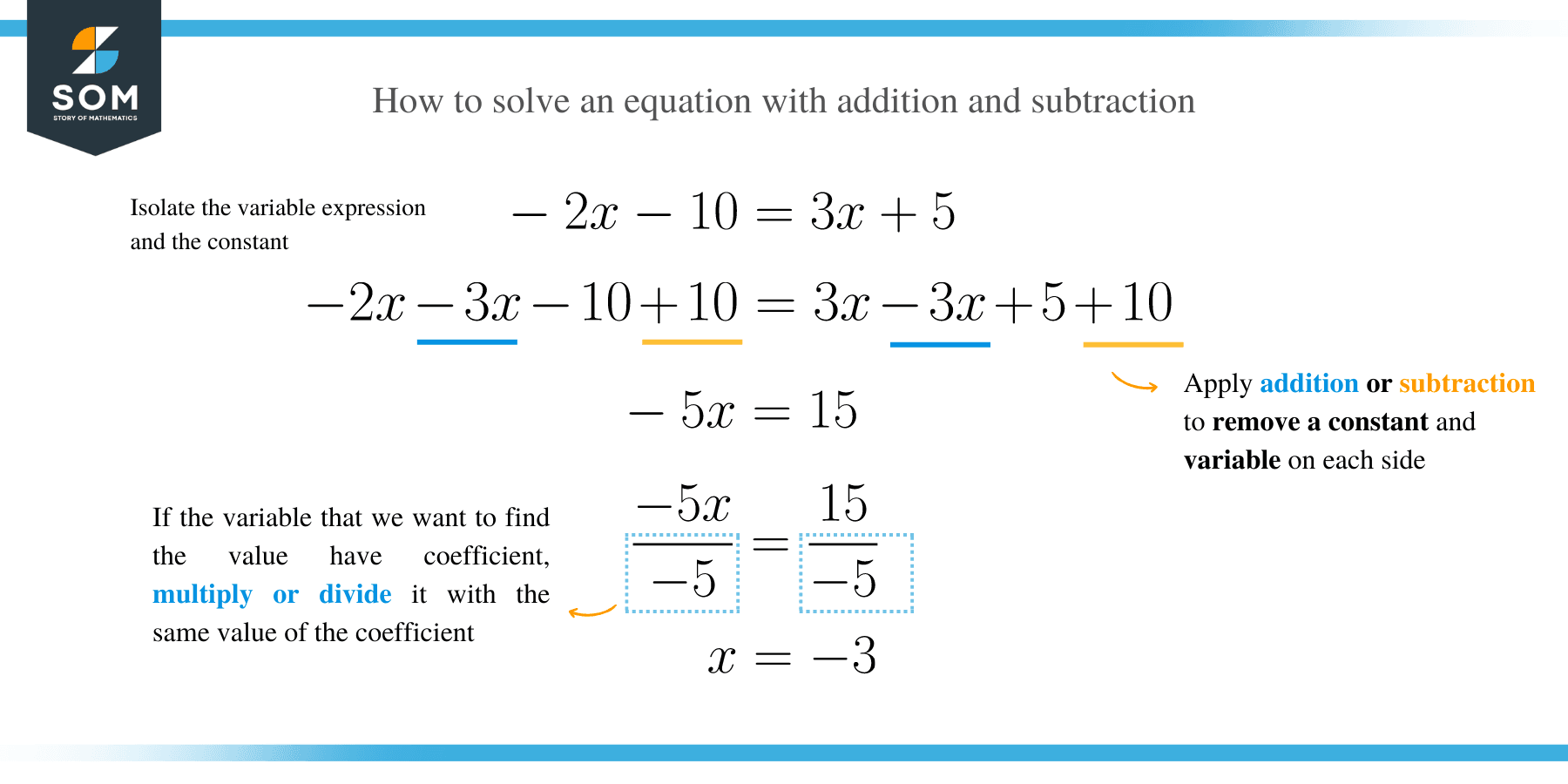
Let’s see a few examples below to understand this concept.

Example 4

Consider an equation 4x –12 = -x + 8.

Since an equation has two sides, you need to perform the same operation on both sides.

Add the variable x to both sides of the equation

⟹ 4x –12 + x = -x + 8 + x.

Simplify

Simplify the equation by collecting the like terms on both sides of the equation.

5x – 12 = 8.

The equation now has only one variable on one side.

Add the constant 12 to both sides of the equation.

The constant attached to the variable is added on both sides.

⟹ 5x – 12 +12 = 8 + 12

Simplify

Simplify the equation by combining the like terms. And 12.

⟹ 5x = 20

Now, divide by the coefficient.

Dividing both sides by the coefficient is simply dividing all through by the number attached to the variable.

The solution is of this equation is, therefore,

x = 4.

Verify your solution

Check whether the solution is correct by plugging the answer into the original equation.

4x –12 = -x + 8

⟹ 4(4) –12 = -4 + 8

4 = 4

Hence, the solution is correct.

Example 5

Solve -12x -5 -9 + 4x = 8x – 13x + 15 – 8

Solution

Simplify by combining the like terms

-8x-14= -5x +7

Add 5x on both sides.

-8x + 5x -14 = -5x +5x + 7

-3w -14=7

Now add 14 to both sides of the equation.

– 3x – 14 + 14 = 7 + 14

-3x = 21

Divide both sides of the equation by -3

-3x/-3 = 21/3

x = 7.

Solving equations with variables on both sides by subtracting

Let’s see a few examples below to understand this concept.

Example 6

Solve the equation 12x + 3 = 4x + 15

Solution

Subtract 4x from each side of the equation.

12x-4x + 3 = 4x – 4x + 15

6x + 3= 15

Subtract the constant 3 from both side.

6x + 3 -3 = 15 – 3

6x = 12

Divide through by 6;

6x/6 = 12/6

x=2

Example 7

Solve the equation 2x − 10 = 4x + 30.

Solution

Subtract 2x from both sides of the equation.

2x -2x -10 = 4x – 2x + 23

-10 = 2x + 30

Subtract both of the sides of the equation by the constant 30.

-10 – 30 = 2x + 30 – 30

– 40 = 2x

Now divide through by 2

-40/2 = 2x/2

-20 = x

Solving linear equations with multiplication

Linear equations are solved by multiplication if division is used in writing the equation. Once you notice a variable being divided, you can use multiplication to solve the equations.

Example 7

Solve x/4 = 8

Solution

Multiply both sides of the equation by the denominator of the fraction,

4(x/4) = 8 x 4

x = 32

Example 8

Solve -x/5 = 9

Solution

Multiply both sides by 5.

5(-x/5) = 9 x 5

-x = 45

Multiply both sides by -1 to make the coefficient of the variable positive.

x = – 45

Solving linear equations with division

To solve linear equations by division, both sides of the equation are divided by the variable’s coefficient. Let’s take a look at the examples below.

Example 9

Solve 2x = 4

Solution

To solve this equation, divide both sides by the coefficient of the variable.

2x/2 = 4/2

x = 2

Example 10

Solve the equation −2x = −8

Solution

Divide both sides of the equation by 2.

−2x/2 = −8/2

−x = − 4

On multiplying both sides by -1, we get;

x = 4

How to solve algebraic equations using the distributive property?

Solving equations using the distributive property entails multiplying a number with the expression within the parentheses. The like terms are then combined and then the variable isolated.

Example 11

Solve 2x – 2(3x – 2) = 2(x –2) + 20

Solution

2x – 2(3x – 2) = 2(x –2) + 20

Use the distributive property to remove brackets
2x – 6x + 4 = 2x – 4 + 20
– 4x + 4 = 2x + 16

Add or subtract on both sides

–4x + 4 – 4 –2x = 2x + 16 – 4 –2x
–6x = 12
x = –2

Check the answer by plugging the solution into the equation.

2x – 2(3x – 2) = 2(x –2) + 20

(2 * –2) – 2((3 * –2) –2) = 2(–2 –2) + 20
12 = 12

Example 12

Solve for x in the equation -3x – 32 = -2(5 – 4x)

Solution

Apply the distributive property to remove the parentheses.

–3x – 32 = – 10 + 8x

Adding both sides of the equation by 3x gives,

-3x + 3x – 32 = – 10 + 8x + 3x

= – 10 + 11x = -32

Add both sides of the equation by 10.

– 10 + 10 + 11x = -32 + 10

11x = -2

Divide the entire equation by 11.

11x/11 = -22/11

x= -2

How to Solve Equations with Fractions?

Do not get panic when you see fractions in an algebraic equation. If you know all the rules for adding, subtracting, multiplying, and dividing, it’s a piece of cake for you.

To solve equations with fractions, you need to transform them into an equation without fractions.

This method is also called the “clearing of fractions.”

In solving equations with fractions, the following steps are followed:

• Determine the lowest common multiple of the denominators (LCD) of all fractions in an equation and multiply by all fractions in the equation.
• Isolate the variable.
• Simplify both sides of an equation by applying simple algebraic operations.
• Apply division or multiplication property to make the coefficient of a variable equal to 1.

Example 13

Solve (3x + 4)/5 = (2x – 3)/3

Solution

The LCD of 5 and 3 is 15, therefore multiply both
(3x + 4)/5 = (2x – 3)/3

{(3x + 4)/5}15 = {(2x – 3)/3}15

9x +12 = 10x -15

Isolate the variable;

9x -10x = -15-12

-x = -25

x =25

Example 14

Solve for x 3/2x + 6/4 = 10/3

Solution

The LCD of 2x, 4, and 3 is 12x

Multiply each fraction in the equation by the LCD.

(3/2x)12x + (6/4)12x = (10/3)12x

=> 18 +18x = 40x

Isolate the variable

22x = 18

x = 18/22

Simplify

x = 9/11

Example 15

Solve for x (2 + 2x)/4 = (1 + 2x)/8

Solution

LCD = 8

Multiply each fraction by the LCD,

=> 4 +4x = 1 +2x

Isolate x;

2x = -3

x = -1.5