Any bank where we deposit money will pay us interest on that amount. Simple interest is one of many forms of interest that banks charge. Before discussing simple interest, we must first understand what loans are. 

What Are Loans?

A loan is a sum of money someone borrows from a bank or financial institution to cover expenses. Home loans, auto loans, student loans, and personal loans are a few instances of loans. A loan amount must be promptly repaid to the authorities with an additional sum, often the interest you paid on the loan. 

The Formula for Simple Interest

We can use the following formula given below to find the simple interest:

Simple Interest = P x R x T

Here, P is the Principal, R is the Rate of Interest in percentage per year, and T is the time calculated in the number of years. We can also write R as the fraction R/100.

Let us look at what the terms above actually mean.

• Principal: The principal is the sum that was first invested or borrowed from the bank or any other financial institution. P stands for the principal. 
• Rate of Interest: The rate at which someone receives their principal amount for a specific period is called the rate. This rate can be 5%, 10%, etc. The interest rate is represented by R.
• Time: The amount of time someone receives the principal is called time. T stands for time.
• Amount: When a person obtains a loan from a bank, they are required to pay back the principal borrowed and the interest amount; this is known as the amount returned. The amount is equal to the sum of the principal amount and simple interest.

Explaining Compound Interest

Compound interest builds up over a set period on both the principal and interest. The principal also includes the interest accrued on a principal over time. Furthermore, the accumulated principal value calculates interest for the subsequent time. Compound interest is the new method of calculating interest used in all international financial and commercial transactions.

Compound interest is calculated regularly, such as annually, semi-annually, or even monthly. This is similar to how reinvested interest on an investment accelerates the growth of the principal over time.

We can explain compound interest with an example. For example, if you borrow \$150 overtime at a rate of 10%, the compound interest per year would look something like \$15, \$33, \$52, and so on.

The Formula for Compound Interest

The total amount, over a specific timespan, is computed using the original principal, the interest rate, and the compound interest. The formula for calculating the amount is given below for an initial principal amount of P, an annual interest rate of r, a period of t in years, and a frequency of n times the interest is compounded annually:

Compound Amount = P$\displaystyle\mathsf{\left(1 + \frac{r}{n}\right)^{nt}}$

To find only the compound interest, we can subtract the principal amount from the compound amount, as shown below:

Compound Interest = P$\displaystyle\mathsf{\left(1 + \dfrac{r}{n}\right)^{nt}}$ – P

Difference Between Simple and Compound Interest

There are two techniques to figure out the interest on a loan amount: simple interest and compound interest.

Compound interest is more challenging to compute than the simple interest due to several fundamental distinctions between the two.

Table 1 explains the critical differences between simple and compound interest:

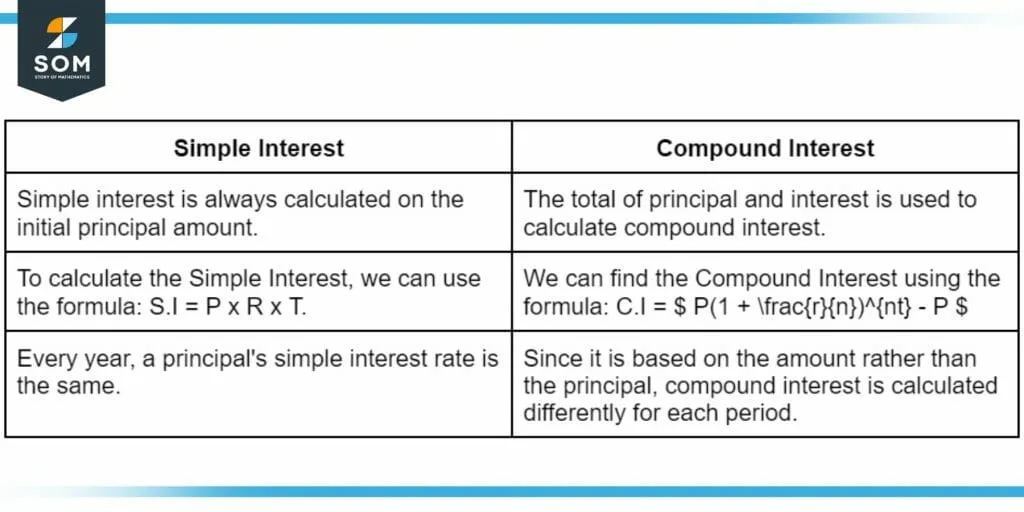
Table 1 – Explaining differences between simple and compound interests.

Applications of Simple Interest

Nowadays, most banks utilize compound Interest on loans since it increases the amount of money they get in interest from their clients. Still, this approach is more complicated and challenging to explain to clients.

However, when banks use simple interest systems, calculations are made simple. Simple interest is quite helpful when a consumer needs a loan for a short time, such as one month, two months, or six months.

Simple interest loans are short-term loans where the interest is calculated daily or weekly rather than annually.

Examples of Simple Interest

To clarify the concept of simple interest, we can look at the following examples:

Example 1

A college student takes a loan of \$1500 from the bank at an interest rate of 10%. Using the simple interest formula to find the interest the student needs to pay after one year. Also, see the interest for three, four, and eight years.

Solution

We can easily find the simple interest in the question given above. We use the formula S.I = P x R x T. We plug in the values in the equation as S.I = 1500 x 10% x 1, and we get the simple interest for one year \$75. 

We keep applying the formula for each time interval, as shown in the table below:

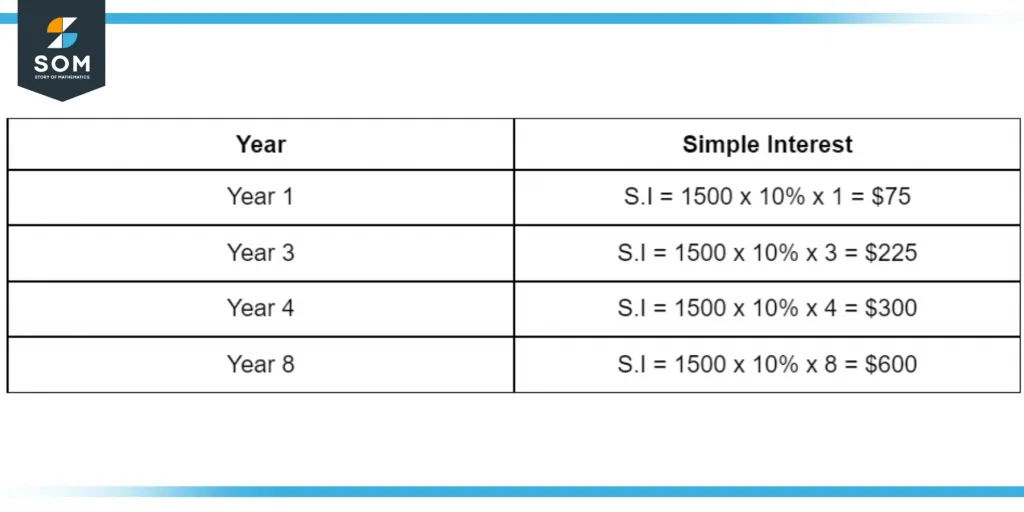
Table 2 – Explaining how simple interest is calculated.

Example 2

Consider a person who borrows \$2000 from a bank at an interest rate of 6%. Using the simple interest formula, compute the amount of interest he needs to pay the bank after two years, five years, seven years, and ten years.

Solution

To find the amount of interest the person has to pay to the bank, we use the simple interest formula S.I = P x R x T. The principal amount is \$2000, the interest rate is 5%, and the time is two years, five years, seven years, and ten years. 

We plug in the values and get the results as shown in the table below:

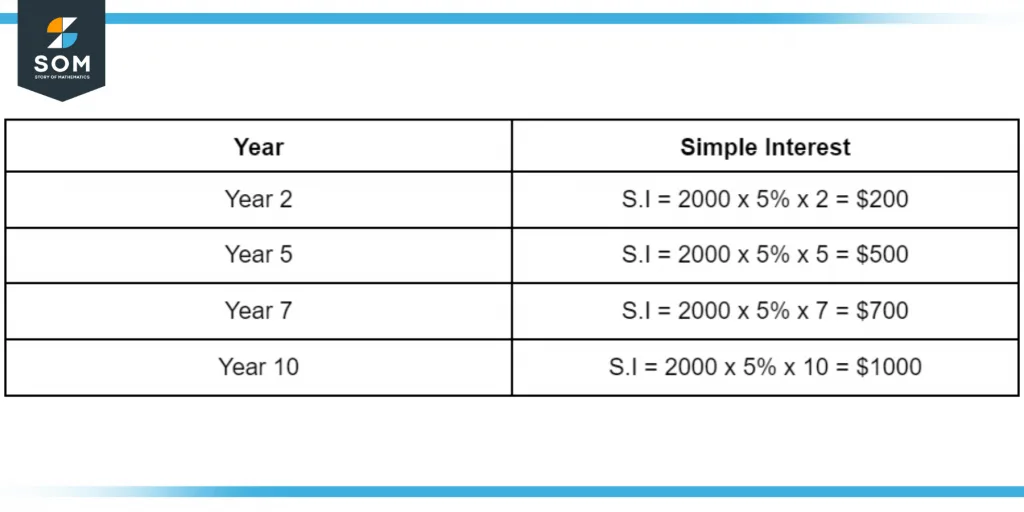
Table 3 – Example of how compound and simple interest is calculated.

All Images/Tables are made using GeoGebra.

Similar Definition < Glossary Index > Simplest Form Algebra Definition