- Home
- >
- Similar Triangles – Explanation & Examples
JUMP TO TOPIC
Similar Triangles – Explanation & Examples
 Now that we are done with the congruent triangles, we can move on to another concept called similar triangles.
Now that we are done with the congruent triangles, we can move on to another concept called similar triangles.
In this article, we will learn about similar triangles, features of similar triangles, how to use postulates and theorems to identify similar triangles, and lastly, how to solve similar triangle problems.
What are Similar Triangles?
The concept of similar triangles and congruent triangles are two different terms that are closely related. Similar triangles are two or more triangles with the same shape, equal pair of corresponding angles, and the same ratio of the corresponding sides.
Illustration of similar triangles:
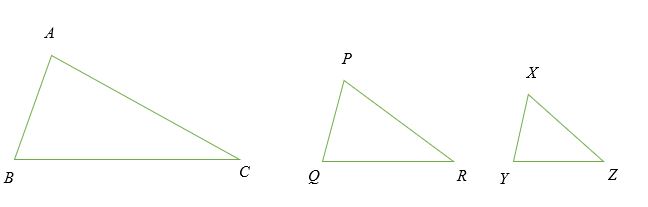
Consider the three triangles below. If:
- The ratio of their corresponding sides is equal.
AB/PQ = AC/PR= BC= QR, AB/XY= AC/XZ= BC/YZ
- ∠ A= ∠ P=∠X, ∠B = ∠Q= ∠Y, ∠C= ∠R =∠Z
Therefore, ΔABC ~ΔPQR~ΔXYZ
Comparison between similar triangles and congruent triangles
| Features | Congruent triangles | Similar Triangles |
| Shape and size | same size and shape | Same shape but different size |
| Symbol | ≅ | ~ |
| Corresponding side lengths | The ratio of corresponding sides is congruent triangles is always equal to a constant number 1. | The ratio of all the corresponding sides in similar triangles is consistent. |
| Corresponding angles | All corresponding angles are equal. | Each pair of corresponding angles are equal. |
How to identify similar triangles?
We can prove similarities in triangles by applying similar triangle theorems. These are postulates or the rules used to check for similar triangles.
There are three rules for checking similar triangles: AA rule, SAS rule, or SSS rule.
Angle-Angle (AA) rule:
With the AA rule, two triangles are said to be similar if two angles in one particular triangle are equal to two angles of another triangle.
Side-Angle-Side (SAS) rule:
The SAS rule states that two triangles are similar if the ratio of their corresponding two sides is equal and also, the angle formed by the two sides is equal.
Side-Side-Side (SSS) rule:
Two triangles are similar if all the corresponding three sides of the given triangles are in the same proportion.
How to Solve Similar Triangles?
There are two types of similar triangle problems; these are problems that require you to prove whether a given set of triangles are similar and those that require you to calculate the missing angles and side lengths of similar triangles.
Let’s take a look at the following examples:
Example 1
Check whether the following triangles are similar
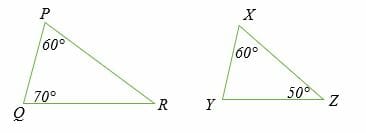
Solution
Sum of interior angles in a triangle = 180°
Therefore, by considering Δ PQR
∠P + ∠Q + ∠R = 180°
60° + 70° + ∠R = 180°
130° + ∠R = 180°
Subtract both sides by 130°.
∠ R= 50°
Consider Δ XYZ
∠X + ∠Y + ∠Z = 180°
∠60° + ∠Y + ∠50°= 180°
∠ 110° + ∠Y = 180 °
Subtract both sides by 110°
∠ Y = 70°
Hence;
- By Angle-Angle (AA) rule, ΔPQR~ΔXYZ.
- ∠Q = ∠ Y = 70° and ∠Z = ∠ R= 50°
Example 2
Find the value of x in the following triangles if, ΔWXY~ΔPOR.
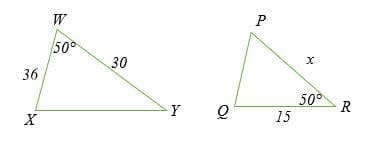
Solution
Given that the two triangles are similar, then;
WY/QR = WX/PR
30/15 = 36/x
Cross multiply
30x = 15 * 36
Divide both side by 30.
x = (15 * 36)/30
x = 18
Therefore, PR = 18
Let’s check if the proportions of the corresponding two sides of the triangles are equal.
WY/QR = WX/PR
30/15 = 36/18
2 = 2 (RHS = LHS)
Example 3
Check whether the two triangles shown below are similar and calculate the value k.
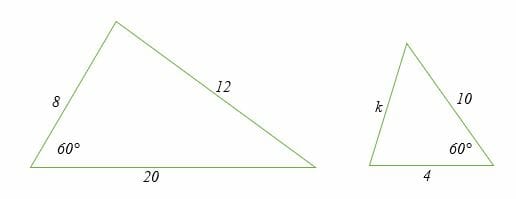
Solution
By Side-Angle-Side (SAS) rule, the two triangles are similar.
Proof:
8/ 4 = 20/10 (LHS = RHS)
2 = 2
Now calculate the value of k
12/k = 8/4
12/k = 2
Multiply both sides by k.
12 = 2k
Divide both sides by 2
12/2 = 2k/2
k = 6.
Example 4
Determine the value of x in the following diagram.
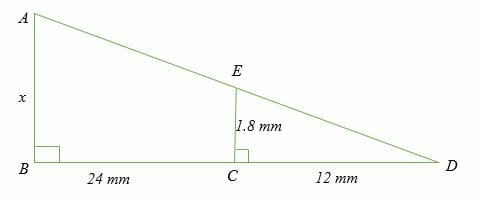
Solution
Let triangle ABD and ECD be similar triangles.
Apply the Side-Angle-Side (SAS) rule, where A = 90 degrees.
AE/EC= BD/CD
x/1.8 = (24 + 12)/12
x/1.8 = 36/12
Cross multiply
12x = 36 * 1.8
Divide both sides by 12.
x = (36 * 1.8)/12
= 5.4
Therefore, the value of x is 5.4 mm.
