Perimeters of Polygons – Explanation & Examples

Have you ever walked around the edge of a school compound or a park? After completion of a whole round, the distance traveled by you will be exactly equal to the perimeter of the compound or the park.

Therefore, the perimeter of a polygon is defined as the total distance around the outside of a polygon.  The perimeter of a polygon is measured in meters, kilometers, yards, etc.

This article will show how to calculate the perimeter of polygons such as triangle, rectangle, square, parallelogram, rhombus, and trapezoid.  We will also discuss the formulas for calculating the perimeter of different polygons with examples.

How to Find the Perimeter of a Polygon?

By definition, the perimeter of a polygon is calculated by taking the sum of all side lengths of a particular polygon. You can also find the perimeter of all polygons, whether they are regular or irregular polygons.

For a regular polygon, the perimeter is equal to the product of one side length and the number of sides of the polygon.

Perimeter of a regular polygon = (length of one side) × number of sides

For example, the perimeter of a regular pentagon whose side length 8 cm, is given by;

Perimeter of a regular pentagon = 8 x 5 = 40 cm.

For an irregular polygon, the perimeter is calculating by adding together the individual side lengths.

For example, the perimeter of an irregular pentagon whose side lengths are; 5 cm, 4 cm, 6 cm, 10 cm, and 9 cm.

Perimeter = (5 + 4 + 6 + 10 + 9) cm

= 34 cm.

What is the formula for finding the perimeter of different polygons?

Different types of regular polygons have their own formulas for calculating perimeter. Let’s take a look.

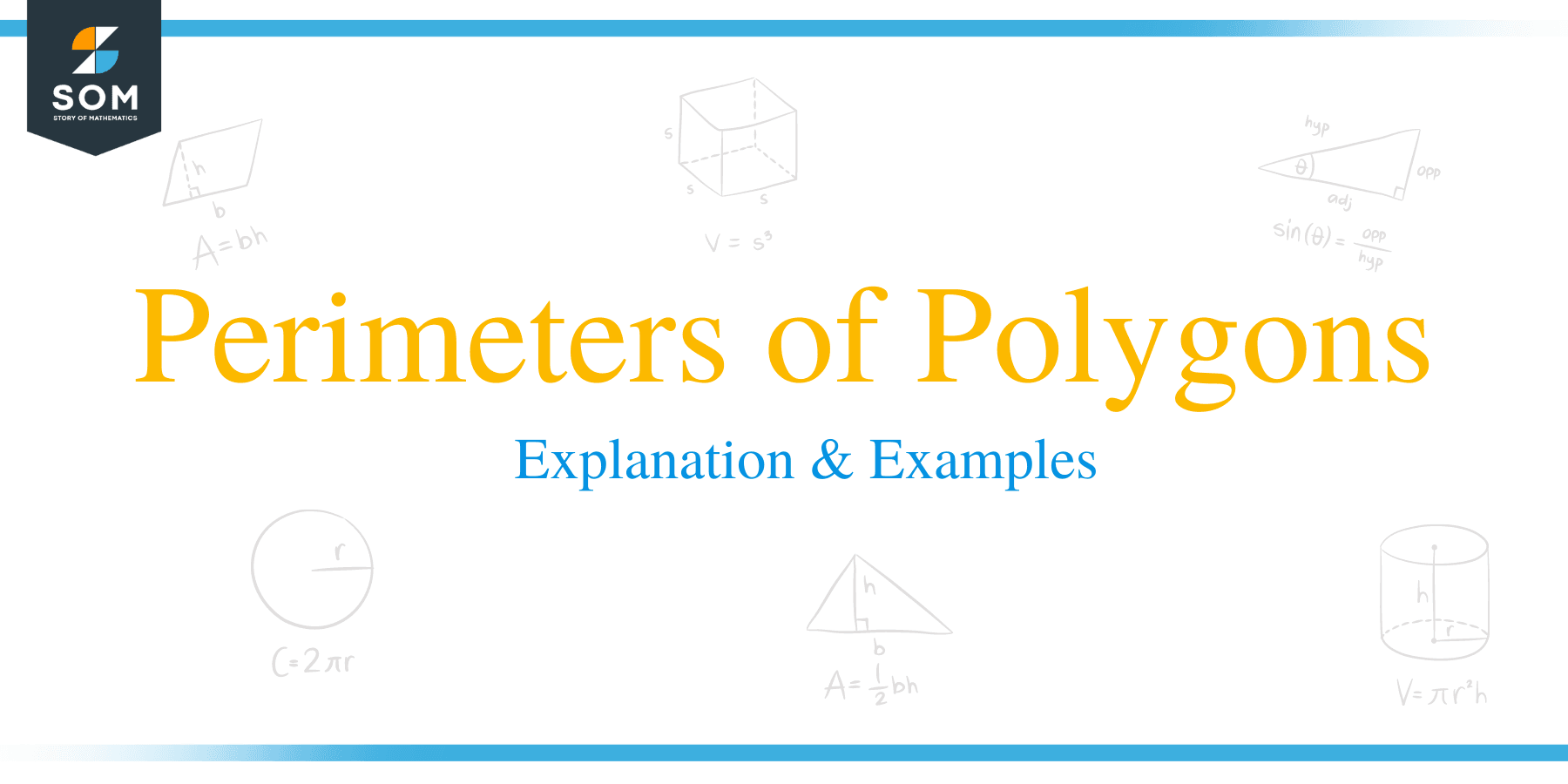
Perimeter of triangles

The perimeter of a triangle is given by;

P = a + b + c

For an equilateral triangle, the perimeter = 3a

Where a, b, and c are the three side lengths of a triangle.

Example 1

Find the perimeter of a triangle whose sides are 20 cm, 15 cm, and 18 cm.

Solution

Perimeter of a triangle = a + b + c

= (20 + 15 + 18) cm

= 53 cm

Example 2

Calculate the perimeter of an equilateral triangle whose side length is 12 cm.

Solution

Perimeter of an equilateral triangle = 3a

= (3 x 12) cm

= 36 cm

Example 3

Determine the value of x for a triangle whose side lengths are, (x + 20) cm, (4x – 5) cm, (2x + 15) cm and the perimeter is 100 cm.

Solution

Perimeter = a + b + c

(x + 20) + (4x – 5) + (2x + 15) = 100 cm

Simplify.

x + 20 + 4x – 5 + 2x + 15 = 100

Collect the like terms.

7x + 30 = 100

Subtract 30 on both sides.

7x = 70

Divide both sides by 7 to get,

x = 10.

Therefore, the value of x = 10 cm.

So, the three side lengths are;

⇒ (x + 20) = (10 + 20) = 30 cm

⇒ (4x – 5) = 4(10) – 5 = 35 cm

⇒ (2x + 15) = 2(10) + 15 = 35 cm.

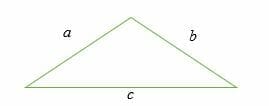
The perimeter of a square and a rhombus

The perimeter of a square is given by,

P = a + a + a + a

P= 4a

Where a = the length of the side length of a square.

Since a square and a rhombus have 4 equal sides, then the perimeter of a rhombus is equal to a square’s perimeter.

Example 4

Calculate the perimeter of a square that has a length of 10 ft.

Solution

P= 4a

= (4 x 10) ft

= 40 ft.

Example 5

Find the perimeter of a rhombus with a side length of 4 inches.

Solution

Perimeter of a rhombus = 4a

= (4 x 4) inches.

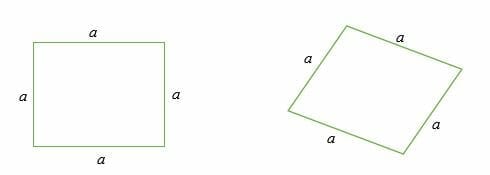
The perimeter of a rectangle and parallelogram

The perimeter of a rectangle and parallelogram are equal. The formula for calculating the perimeter of a rectangle is given by,

Perimeter of a rectangle = Length + Length + width + width

Perimeter of a rectangle = 2 (L + W)

where,

L = length of a rectangle or parallelogram and

W = width of the rectangle or parallelogram.

 Example 6

What is the perimeter of a rectangle with a length of 100 mm and a width of 80 mm?

Solution

Perimeter of a rectangle = 2 (L + W).

= 2 (100 + 80) mm

= 2 x 180 mm

P = 360 mm

Example 7

Find the perimeter of a parallelogram whose length is 12 yards and whose width is 5 yards.

Solution

Perimeter of a parallelogram = 2(L + W).

= 2 (12 + 5) yards.

= 2 x 17 yards

P = 34 yards

Example 8

The width of a rectangle 5 m less than the length. Find the length and width of the rectangle if its perimeter is 34 m.

Solution

The width is 5 m less than the length.

Let, the length = x.

Width = x – 5

But, the perimeter = 2 (L + W)

34 = 2 (x – 5 + x)

34 = 2 (2x – 5)

34 = 4x -10

Add 10 on both sides.

44 = 4x

Divide both sides by 4.

x = 11

Therefore, the length of the rectangle is 11m, and the width is 6 m.

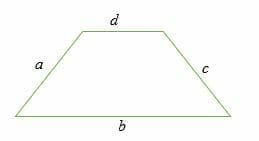
The perimeter of a trapezoid

The perimeter of a trapezoid is given by,

P = a + b + c + d

where a, b, c, and d are the lengths of each side.

Example 9

Calculate the perimeter of the trapezoid shown below.

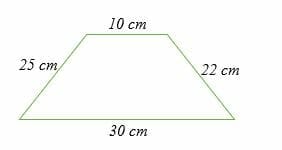
Solution

Perimeter of a trapezoid = a + b + c + d

= (25 + 30 + 22 + 10)

= 87 cm.

Perimeter of irregular polygons

As stated earlier, the perimeter of an irregular polygon is equal to the sum of all side lengths.

Example 10

Calculate the perimeter of the diagram shown below if the dimensions are in mm.

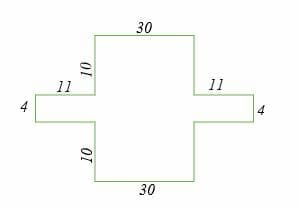
Solution

Perimeter = (4 + 11 + 10 + 30 + 10 + 11 + 4 + 11 + 10 + 30+ 10 + 11) mm

P = 152 mm