Percentage Conversion – Conversion Method & Examples

Fractions and percent are the two terms used interchangeably to compare and describe the size of quantities. Converting fractions to percent and vice versa may seem challenging to some students but is just a simple two-step process. Before we can the conversion process, let take a quick recap of what a fraction and a percent are.

A percentage is a means of indicating a portion out of 100. It is a ratio whose denominator is 100. Percent is represented by the percentage sign (%). The term percent originates from a Latin word –per centum which means by a hundred.

Example of percent include: 4%, 10%, 100% etc.

A fraction is defined as a portion of a whole quantity. A fraction simply represents the number of parts of a certain number divide a whole number.  A simple fraction is composed of two parts namely the numerator, which is the number at the top, and the denominator being the number at the bottom. The slash line usually separates the numerator and the denominator. Examples of fractions include: 2/5, 1/3, 4/9 etc.

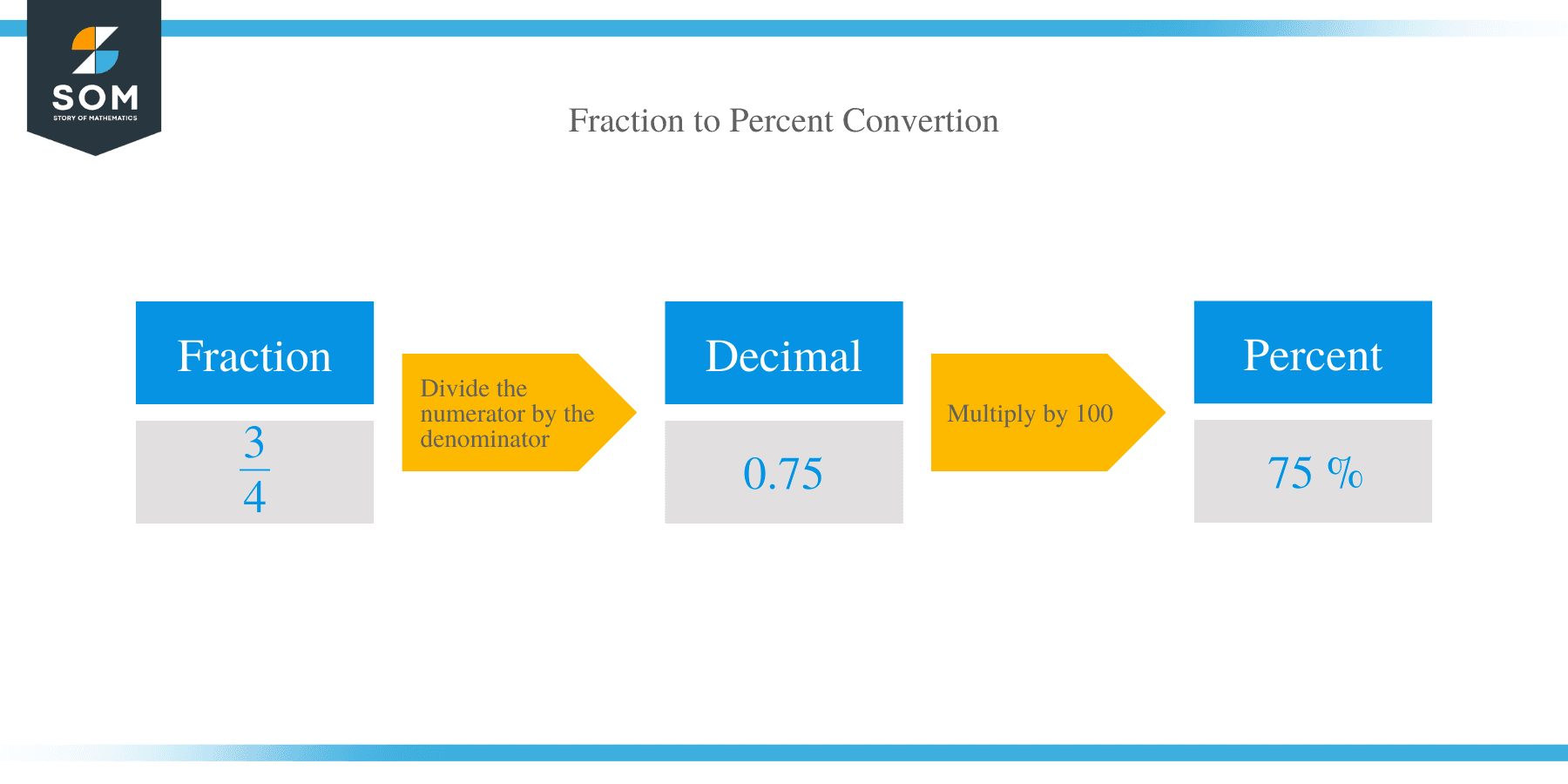
How to Convert a Fraction to Percent?

To convert fraction to a percent, you only need to follow the two simple steps below:

• First, convert the given fraction into a decimal. Use long division method to divide the numerator by the denominator
• Multiply the resultant decimal by 100.
• It should be noted the result is written followed by the percentage sign (%) behind.

In order to understand the process well, let’s solve a few examples by applying the above steps.

Example 1

Convert 3/4 to a percent.

Explanation

• Begin by converting the fraction 3/4 into decimal.
• Divide the numerator by the denominator: 3 ÷ 4 = 0.75
• Multiply the decimal by 100 and write the result with the percentage sign: 0.75 × 100 = 75%.
• Therefore, 3/4 = 75%

Example 2

Convert 3/16 to per cent.

Explanation

• Convert 3/16 into decimal by dividing the numerator by the denominator
• 3 ÷ 16 = 0.1875
• Now multiply the decimal number by 100 and write the result followed by percentage symbol (%): 0.1875 × 100 = 18.75%
• Therefore, 3/16 = 75 %

Other examples of conversion of fractions to percent are:

1. 3/25 = (0.12 × 100) % = 12 %
2. 4/5 = (0.8 × 100) % = 80 %

• 3/4 = (0.75 × 100) % = 75 %

1. 2/3 = (2/3 × 100) % = 0.67 %
2. 1 = (1 × 100) % = 100 %

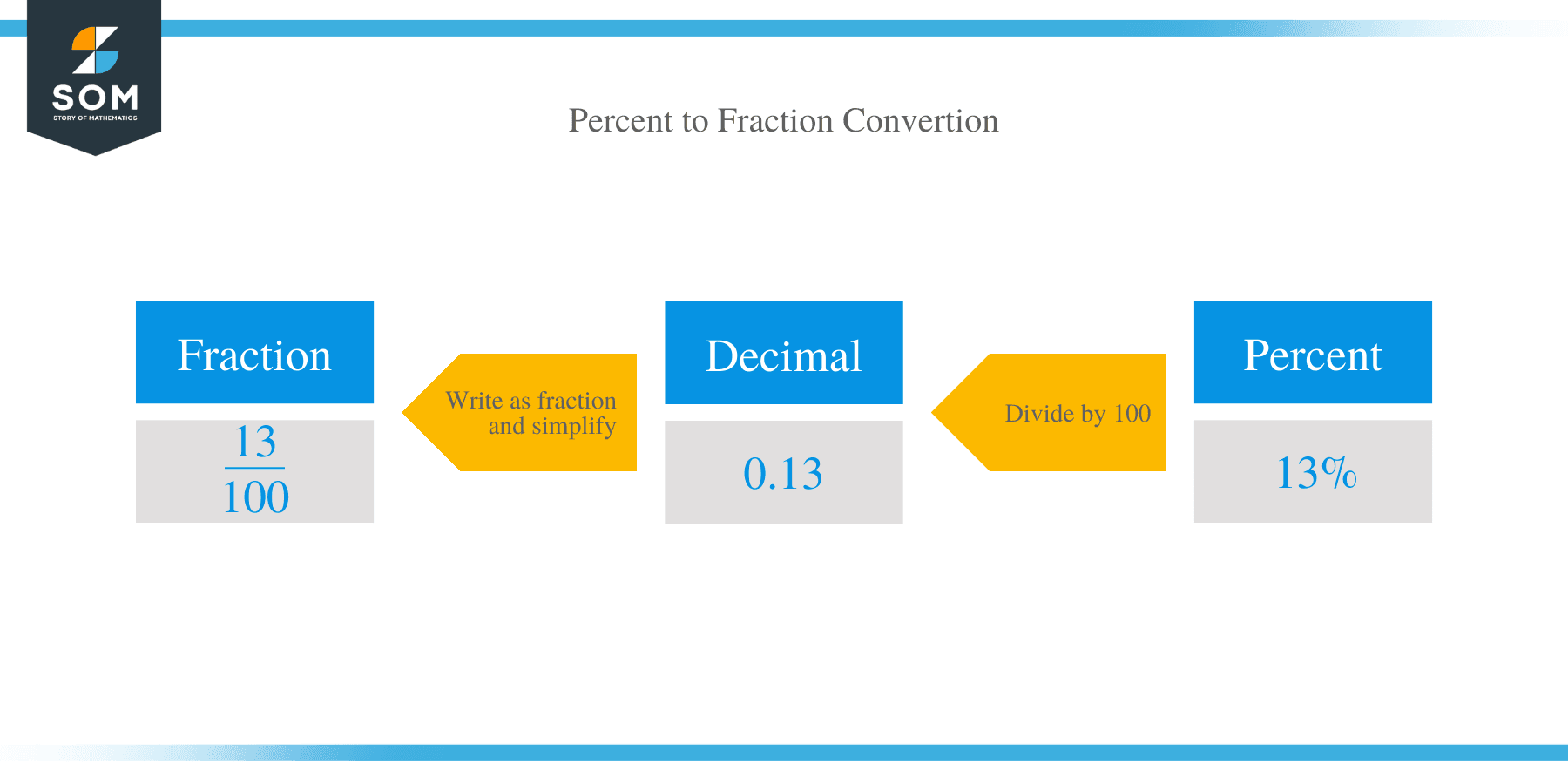
How to Convert Percent to fraction?

Conversion of percent to fraction is another straight forward operation. You only need to follow the steps outlined below in this article:

• Remove the percentage sign (%) from the given number.
• Write the number as the numerator and 100 as the denominator
• Simplify the fraction, if possible, in its lowest terms.

Let’s also solves some examples by using the steps:

Example 3

Convert 13% to fraction.

Explanation

• Remove the percentage sign (%) and divide the number by 100.
• 13% = 13÷100 = 13/100
• This cannot be simplified and so, the answer is 13/100

Example 4

Convert 2.5% to a fraction.

Explanation

• Remove the percentage sign (%).
• In this case, the given percentage is a decimal number. We just use the same steps but now count number of decimal places and multiply by 100 in powers of 10.
• 5 has 1 decimal place, therefore the power of 10 will be 10 ¹.
• Take the number as a whole number and divide by the product of 100 and 10 ¹.

= 25/ (100 x 10¹) = 25/1000

• The fraction can be simplified into the lowest terms; 25/1000 = 1/ 40.
• Therefore, 2.5% = 1/40

Example 5

Convert 0.005% to fraction.

Explanation

• Remove the percentage sign (%)
• Count the number of decimal places in the number. In this case, 0.005 has 3 decimal places.
• Write the number as a whole number and divide by the product of 100 and 10³.

= 5/ (100 x 10 ³)

= 5/100000

• Reduce the fraction: 5/100000 = 1/20000
• Therefore, 0.005% = 1/20000

Other examples of conversion of percent to fraction:

1. 62 % = 62/100 = 31/50
2. 30 % = 30/100 = 3/10

• 0.5 % = 5/10 % = 5/10 × 1/100 = 1/200

30. 30.2% = 322/10 % = 322/10 × 1/100 = 322/1000 = 151/500
31. 0.4 % = 4/10 % = 4/10 × 1/100 = 4/1000 = 1/250
32. 3²/₅% = 17/5 % = 17/5 × 1/100 = 17/500

• 2/5 % = 2/5 × 1/100 = 1/250
• 16²/₃ % = 50/3 % = 50/3 × 1/100 = 50/300 = 1/6