Percentage Change – Explanation & Examples

Percentage in mathematics is a number or ratio which can be represented as a fraction of 100. The term percent originates from a Latin word ‘per centum’ which means per 100. The symbol (%) is used to denote percentage. For example, we can express 50 percent as 50%.

Percentage change, percentage increase and decrease and percentage difference are the most common terms we encounter in our daily life. Calculating percent change is useful in various daily applications such as finance, sales, tax and inflation rate, physics and other fields of mathematics.

In this article, you will learn how to calculate percent change, percentage difference and percentage decrease and increase.

How to Calculate Percent Change?

Percent change can be defined as the difference between the old and new value of a quantity expressed in percentages. Calculating the percent change between two given quantities is quite an easy process. When the initial or old value and final or new values of a quantity are known, percent change formula is applied determine the percent change.

The formula is given by;

Percentage change = [(New Value − Old Value)/ Old Value] × 100%

If the value of the percent change is positive, then it referred to as percentage increase and when the value is negative, it is referred to as percentage decrease.

Percentage difference

The percentage difference of two numbers is the absolute value of the difference between the two quantities, divided by the average of those two quantities, multiplied by 100%. The formula for percentage difference is:

Percentage difference = [(difference between the two values)/ (the average mean of the values)] x 100%

Percentage difference = [(second value -first value)/{(second value + first value)/2}] x 100%

Example 1

The price of a kilo of rice went from $10 to $12.5, what is the percentage change?

Explanation

• The old value of sugar = $ 10
• New value = $12.5
• Now apply the percent change formula;
• Percentage change = [(New Value − Old Value)/ Old Value] ×100%

= [(12.5 -10)/10] x 100%

= (2.5/10) x 100%

= 25%

In this case, the percent change is positive and therefore, it is an increase.

Example 2

The weight of a boy this year is 48 kg. If his weight was 50 kg the previous year, what is the percentage change in weight of the boy?

Explanation

• The new weight = 48
• Old weight of the boy = 50
• Applying the percent formula, substitute the values
• Percentage change = [(New Value − Old Value)/ Old Value] ×100%

= [(48 -50)/50] x 100%

= -2/50 x 100

= – 4%; which is a percentage decrease

Example 3

Mary is 8 years old while Peter is 12 years. Find the percentage difference of their ages?

Explanation

• Apply the formula for percentage difference;
• Percentage difference = [(difference between the two values)/ (the average mean of the values)] x 100%
• [(12- 8)/ {(12+8)/2}] x 100

= 4/10 x 100

= 40%

The percentage difference is therefore, 40%

Percentage increase and decrease

Some values we encounter on a regular basis change over a given period of time. When the value of a quantity reduces, it is termed as depreciation and when the value rises, it called appreciation. We use percentage decrease or increase to compare the quantity of a value of a period of time.

The formula for percentage increase is given by;

Percentage increase = [(Increased value – Original value)/Original value] x 100%

Similarly, the formula for percentage decrease is given as;

Percentage decrease = = [(Decreased value – Original value)/Original value] x 100%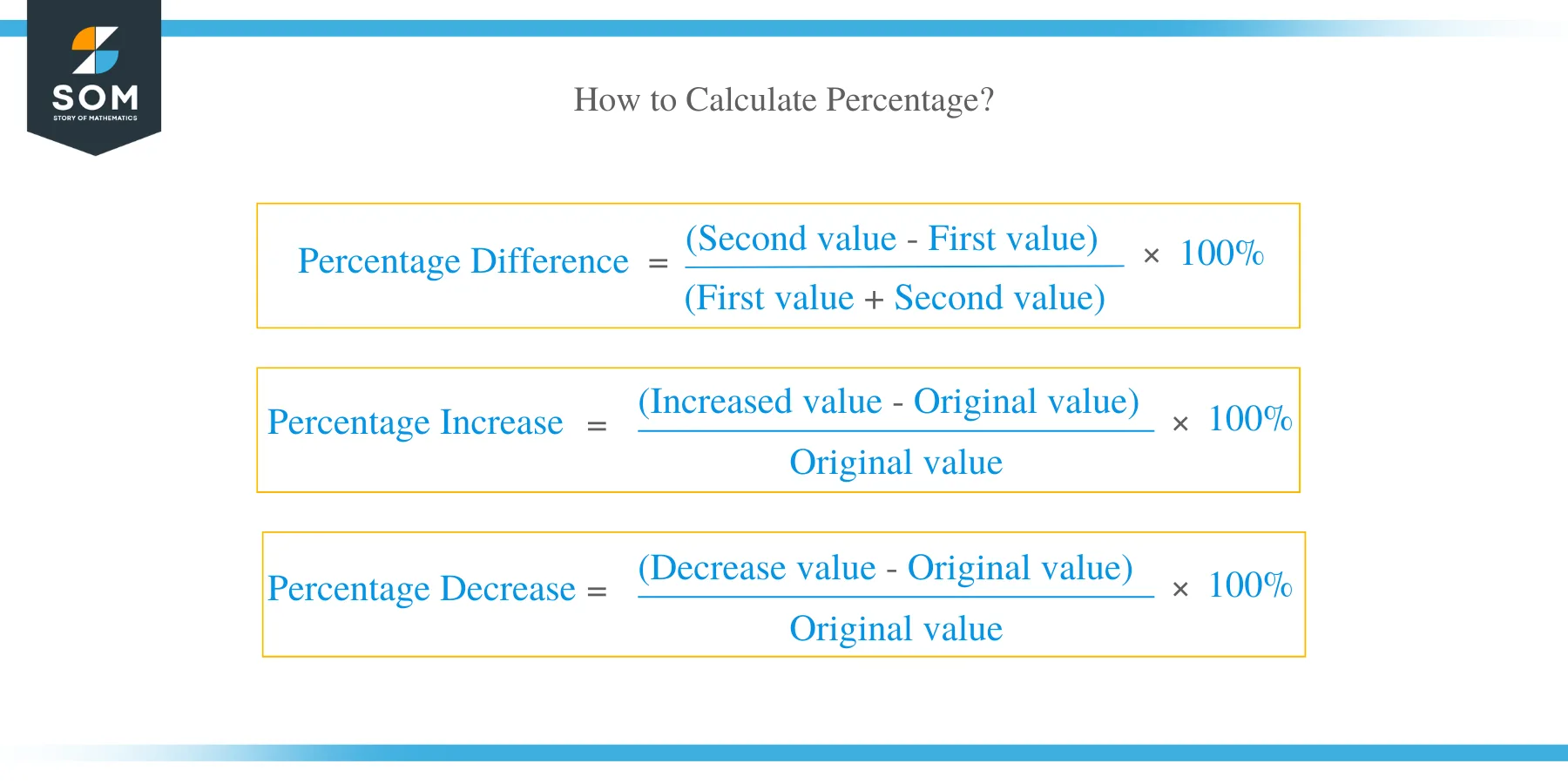

Example 4

The population of a certain town increased from 20000 to 21250 over a given period of time. Find the population increase in as a percentage

Explanation

• Original population = 20000
• The increased population = 21250
• Percentage increase = [(Increased value – Original value)/Original value] x 100%
• Percentage increase = [(21250 – 20000)/ 20000] x 100%

= 1250/20000 × 100 %

= 125000/20000 %

= 25/4 %

= 6.25%

Thus, population increase is 6.25%

Example 5

Instead of using a correct number 42, the number 24 was used during calculation. Find the error in calculation as a percentage.

Explanation

• Original number =42
• New number =24
• Apply the percentage decrease formula

Percentage decrease = [(Decreased value – Original value)/Original value] x 100%

= [(42- 24)/42] x 100%

= 18/42 x 100

= 42.86%

Therefore, the percentage error in calculation is 42.86%