Numbers in Scientific Notation – Explanation & Examples

The distance from the Earth to the nearest star is given as 40,208,000,000,000 kilometres, and the distance between the nucleus and electron of a hydrogen atom is 0.00000000005291772 meters. Whenever you see such a huge number or very small number, what normally comes in your mind? Performing calculations using such numbers is very tiresome and boring, because the number can’t even fit on a piece of paper.

Scientists perform calculations involving large or very small numbers by using scientific notations. What are then scientific notations? Why and how do we use it. How is scientific notation done? Well, this article is going to discuss and answer these questions in detail.

What is Scientific Notation?

To start with, scientific notation is a form of expressing very small or large numbers in a simpler form. It is sometimes referred to as standard index form.

The general representation of scientific notation is:  a x 10^b   where 1 ≤ a < 10 and b can be any integer. The number b is known as the order of magnitude while the number a is referred to as the mantissa or significand. The number a is the coefficient of the scientific notation and is normally greater than or equal to 1 and less than 10.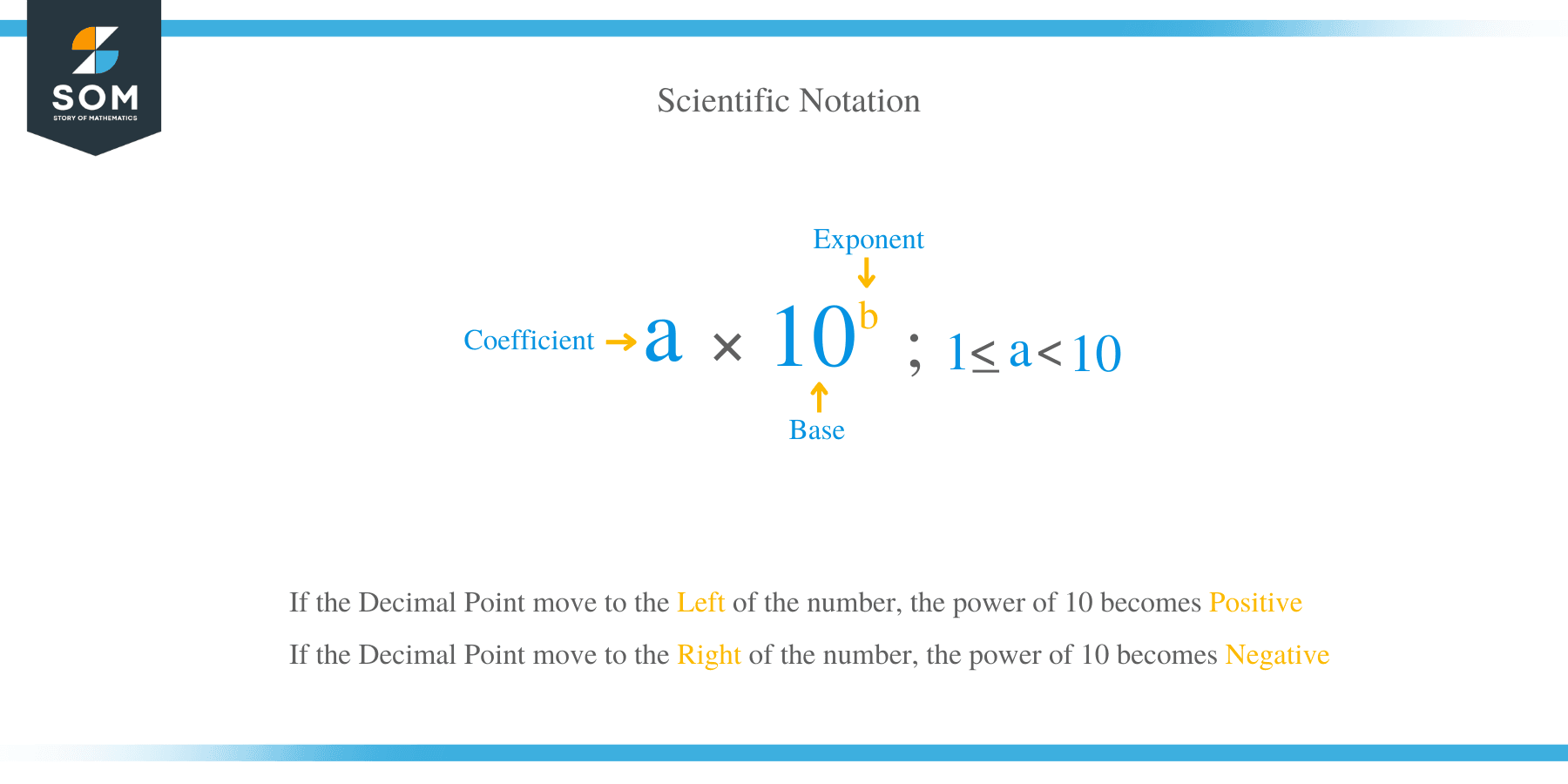

How to do Scientific Notation?

To write a number in scientific notation, the following steps are followed:

• If the given number is greater or equals to 10, the decimal point is moved to the left of the number and so, the power of 10 becomes positive. For example, 6000 in scientific notation is 6 × 10³
• When the scientific notation of any large numbers is expressed, then we use positive exponents for base 10. For example:
  20000 = 2 x 10⁵, where 5 is the positive exponent
• If the given number is less than 1, the decimal point is moved to the right, and so the power of 10 becomes negative. For example, 0.006 in scientific notation is 6 × 0.001 = 6 × 10^-3
• When expressing small numbers in scientific notation, we use negative exponents for the base of 10. For example, 0.00002 = 2 x 10^-5, where -5 is the negative exponent.

Example 1

Convert 0.000000046 into scientific notation.

Explanation

• The number is less than 1, therefore the decimal point is moved to the right up to 8 places
• The decimal point is moved 8 steps to form 4.6 because, the number 4.6 is greater than 1 and less than 10.
• The power of base 10 will be a negative exponent since we moved to the right.
• Therefore, the scientific notation of 0.00000046 ⇒6 × 10^-7

Example 2

Convert 4000000000 in scientific notation.

Explanation

• 4000000000 is more 10, therefore, move the decimal point to the left 9 places.
• All the zeros are removed and the number multiplied by 10 raised to 9
• Now the number becomes 4 x 10⁹
• In this case a positive exponent is used because the decimal point I moved to the left.
• Hence, 4 × 10⁹ is the scientific notation of the number.

Example 3

Convert 4.306 × 10⁷ to standard notation.

Explanation

• Already 4.306 × 10⁷is in scientific notation, so to change it to standard notation;
• Multiply 4.306 by 10000000
• 306 × 10⁷= 14.306 × 10000000 = 43,060,000