Negative Numbers – Explanation & Examples

The study of negative numbers might seem a little boring to some people.

These individuals have questions like why to study negative numbers?

How are negative numbers connected to their daily life? 

Well, in this article, we will learn what negative numbers are, their operations and how the numbers are connected real life.

The history of negative numbers is dated back a thousand years a go when mathematicians from the Indian subcontinent started using them. Europeans later showed interest in negative numbers but were very reluctant to embrace them.

Egyptians were also dismissive of negative and at some point, they regarded negative number as being ridiculous. This because the mathematics they were using at that time was only based on geometrical concepts like circumference and area.  Europeans later started catching up with negative numbers when the scholars began translating Arabic texts retrieved from North Africa.

From this brief history, we have learned that, yet these generations of brilliant and intelligent individuals initially proved difficult to accept the concept of negative numbers.

They finally embraced the idea after discovering the significance of negative numbers.

What is a Negative Number?

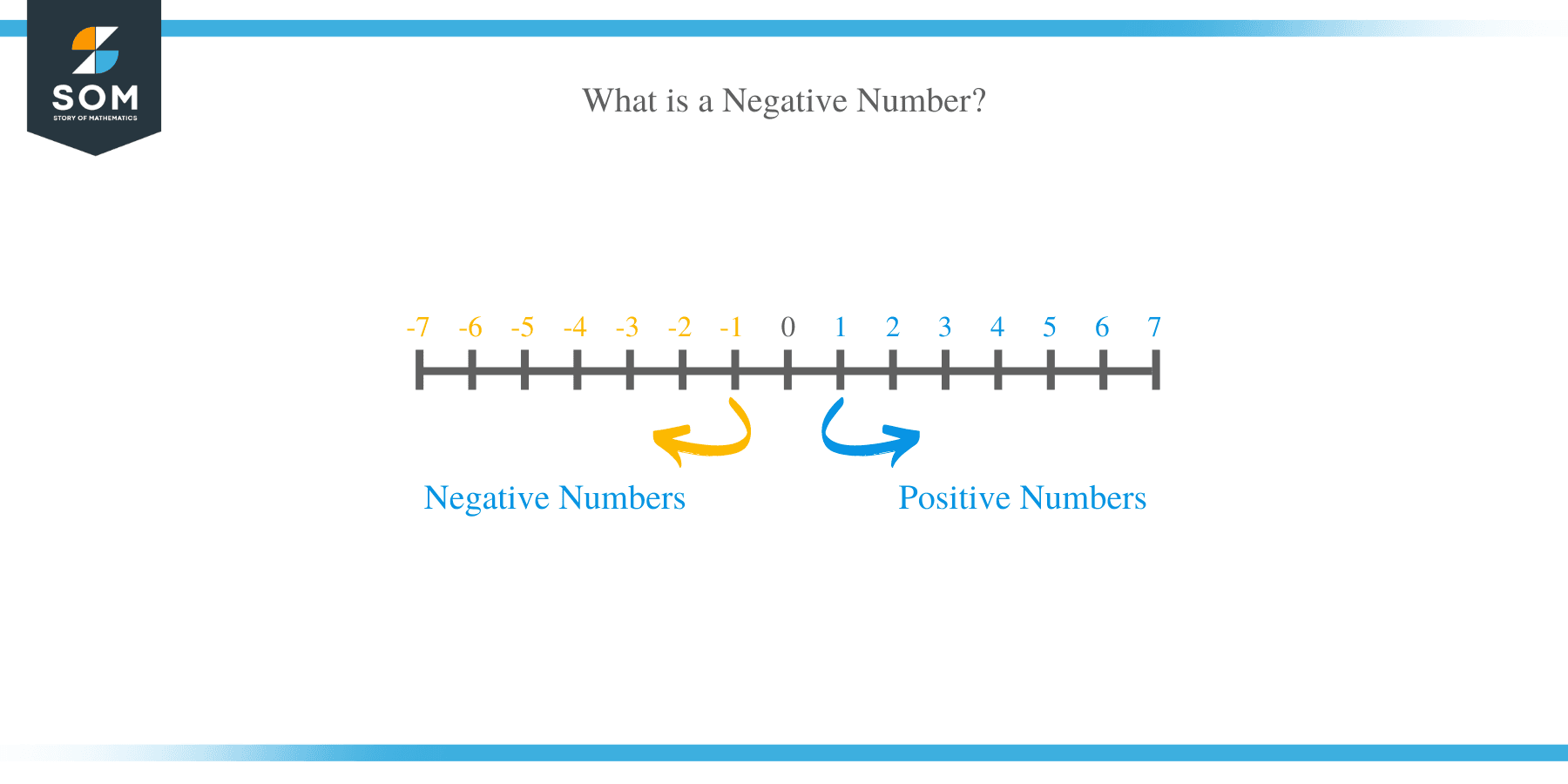
A negative number is a number that its value is less than zero. Negative numbers are symbolized by a minus or a dash (-) sign in front of a number.

They are represented on the number line to the left of origin. Negative numbers can be either be whole numbers, fractions or decimals. For instance, – 2, – 3, – 4, – 5, -2/3, -5/7, -3/4, -0.5, -0.7. etc. are examples of negative numbers.  In this case, these numbers are pronounced as negative two, negative three, negative four and so on.

Negative number have several different interpretations. And these are:

• A negative number is a number that is less than zero
• Numbers that are to the left of zero on the number line
• A number that is the opposite of a positive number
• A negative number represents loss or absence of something.
• A quantity that has a direction

What is a Negative Integer?

A negative integer is a whole number that has value less than zero. Negative integers are normally whole numbers, for example, -3, -5, -8, -10 etc.

Operations with Negative integers

Negative integers have rules for performing different calculations. These are:

• Adding a negative and a positive integer

When adding a negative and a positive integer together, subtract the integers and write the sign of the greater absolute value. In other words, when small negative integer is added to a larger positive integer, the integers are subtracted and given a positive sign. For example,

8 + (- 2) = 6. Similarly, when a small positive and a big negative integer are added, the sum is always negative. For example, – 5 + 3 = – 2.

• Adding negative integers

When adding negative integers, the numbers are added and sum assumes the sign of the original integers. For example, – 5 + (-1) = – 6.

• Subtracting signed integers

Subtracting a positive integer from a negative integer is equivalent to adding the negative of that integer. For example, -10 – 15 = -10 + (-15) = -25.

Subtracting a negative integer from another negative integer is equal to adding the positive of that integer. For example, 13 – (-14) = 13 + 14 = 27.

• Multiplication and division of negative integers

When a negative integer is multiplied by another negative integer, the product is positive. Example, -4 x -4 = 16. Similarly, dividing a negative integer by another negative integer results in a positive quotient.

Multiplication of a positive integer by another negative integer results in a negative product. Example, -2 x 5 = -10. And the division a positive integer by a negative result in a negative quotient.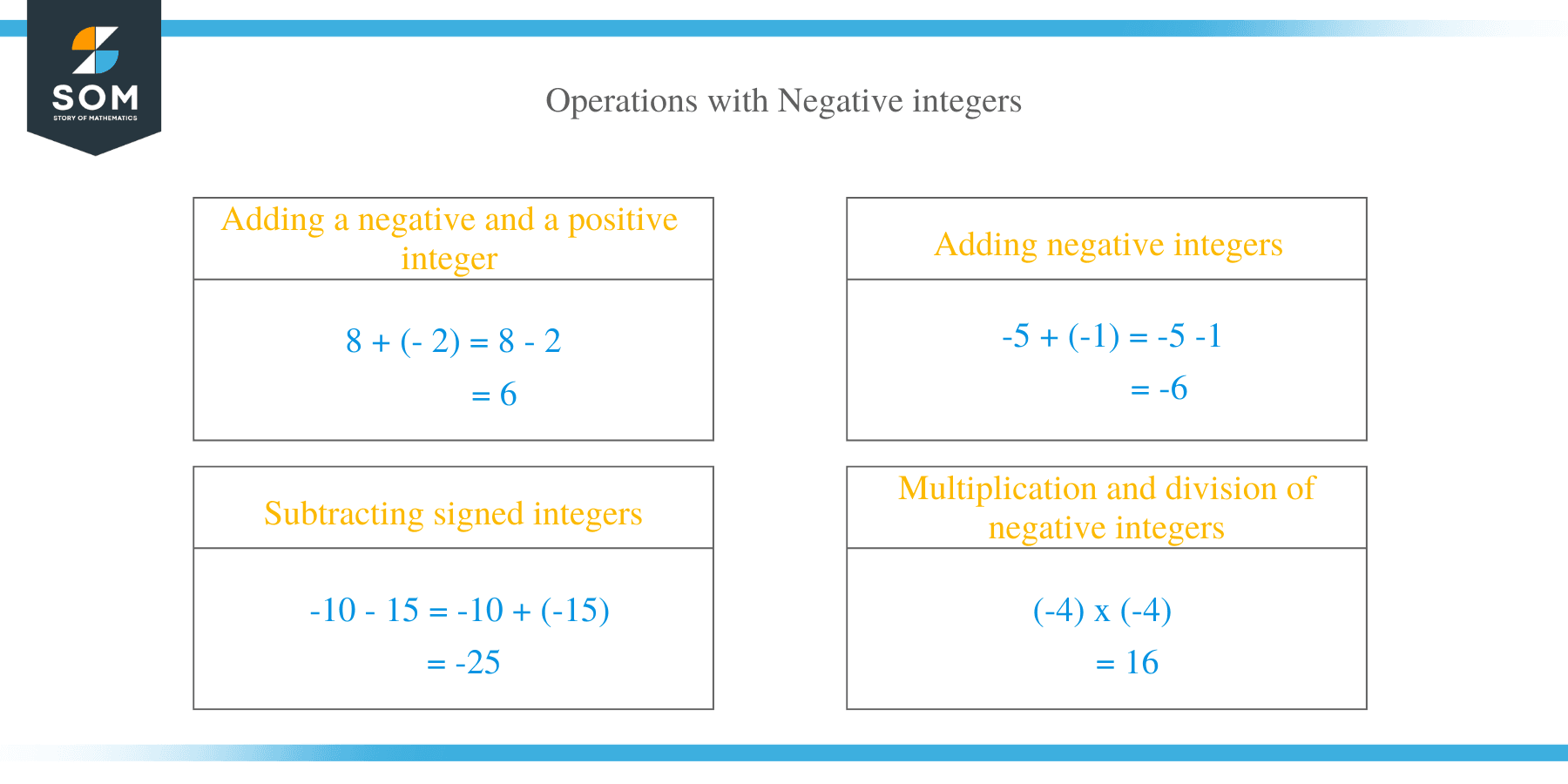

Application of Negative Integers in Real Life

Irrespective of their value, negative integers are widely applied in different fields of life. The following real-life applications of negative numbers will motivate you to see the advantage of studying them.

• The Sector of Banking and Finance.

Banks and financial institutions entail debit, credits and money. For this reason, there is need to have numbers which differentiate between a credit and debit transaction. Profits and losses are also defined by positive and negative number respectively. Another field where negative numbers are used is the stock market. Positive and negative numbers are used depict the ups and downs of share price.

Deposits are normally represented by a positive sign whereas withdrawals are denoted by a negative sign.

• Science, engineering and medicine

Negative numbers are used in weather forecasting to show the temperature of a region.  Negative integers are used to show the temperature on Fahrenheit and Celsius scales.

In engineering for example, instruments such as boilers and steam engines use pressure gauges and thermometers calibrated from negative to positive integers.

Instruments for measuring blood pressure, body weight and drug testing all operate on the concept negative or positive scale.

• Other real-life applications of negative integers

The goal differences in sports such as football, hockey and basketball are denoted by negative integers.

Lifts, speedometers and Alco-blows all use negative and positive values.