Multiplying Polynomials – Explanation & Examples

Many students will find the lesson of multiplication of polynomials a bit challenging and boring. This article will help you to understand how different types of polynomials are multiplied.

Before jumping into multiplying polynomials, let’s recall what monomials, binomials, and polynomials are.

A monomial is an expression with one term. Examples of monomial expression are 3x, 5y, 6z, 2x, etc. Monomial expressions are multiplied the same way integers are multiplied.

A binomial is an algebraic expression with two terms separated by either the addition sign (+) or subtraction sign (-). Examples of binomial expressions are 2x + 3, 3x – 1, 2x+5y, 6x−3y, etc. Binomial expressions are multiplied using the FOIL method. F-O-I- L is the short form of ‘first, outer, inner and last.’ The general formula of foil method is; (a + b) × (m + n) = am + an + bm + bn.

Let’s take a look at the example below.

Example 1

Multiply (x – 3) (2x – 9)

Solution

• Multiply the first terms together;

= (x) * (2x) = 2x ²

• Multiply the outermost terms of each binomial;

= (x) *(–9) = –9x

• Multiply the inner terms of the binomials;

= (–3) * (2x) = –6x

• Multiply the last terms of each binomial;

= (–3) * (–9) = 27

• Sum up the products following the foil order and collect the like terms;

= 2x ² – 9x -6x + 27

= 2x ² – 15x +27

On the other hand, a polynomial is an algebraic expression consisting of one or more terms involving constants and variables with coefficients and exponents.

The terms in a polynomial are linked by addition, subtraction, or multiplication, but not division.

It is also important to note that, a polynomial can’t have fractional or negative exponents. Examples of polynomials are; 3y² + 2x + 5, x³ + 2 x ² − 9 x – 4, 10 x ³ + 5 x + y, 4x² – 5x + 7) etc.

How to Multiply Polynomials?

To multiply polynomials, we use the distributive property whereby the first term in one polynomial is multiplied by each term in the other polynomial.

The resulting polynomial is then simplified by adding or subtracting identical terms. You should note that the resulting polynomial has a higher degree than the original polynomials.

NOTE: To multiply variables, you multiply their coefficients and then add the exponents.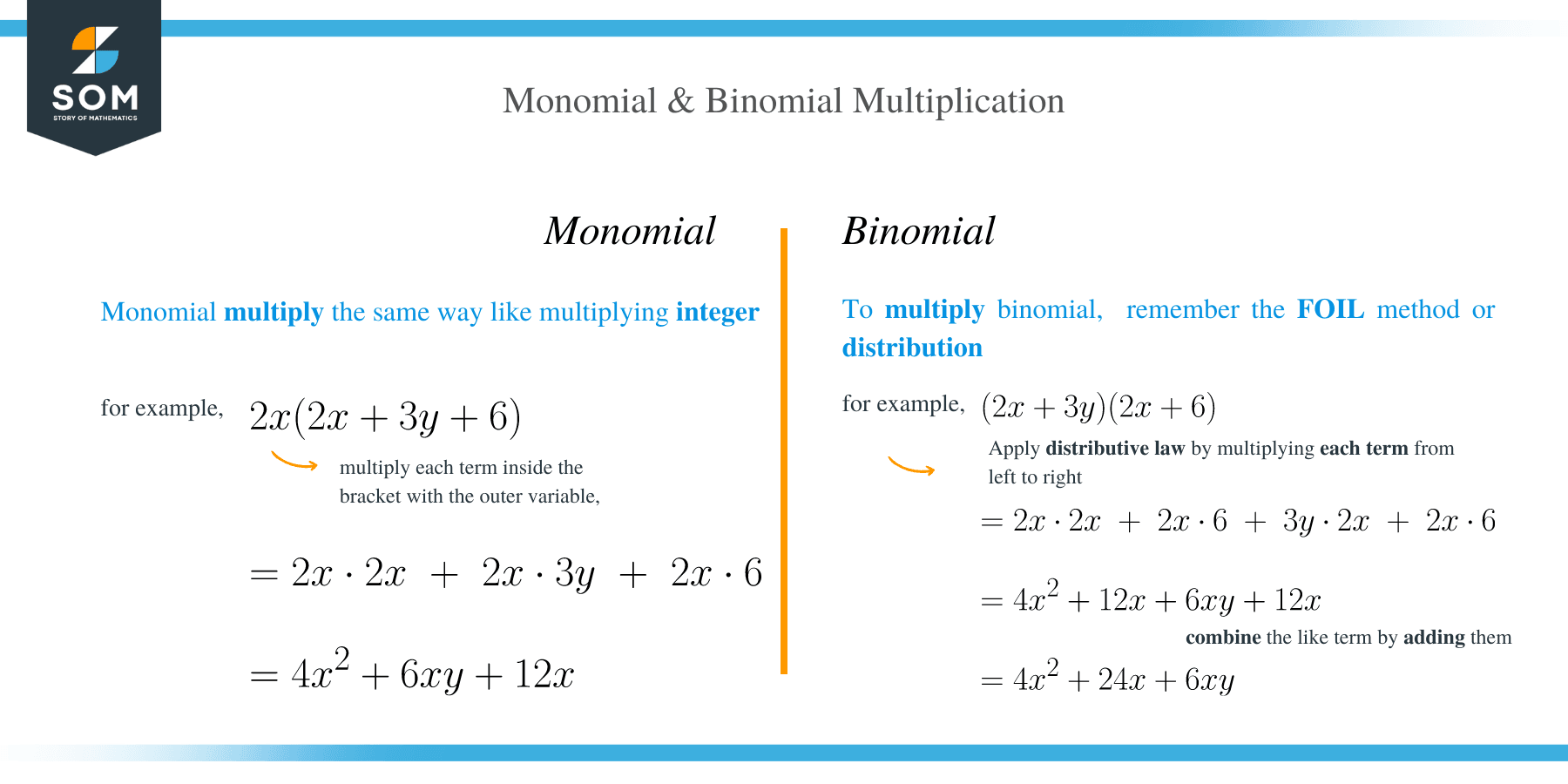

Multiplying a polynomial by a monomial

Let’s understand this concept with a help of a few examples below.

Example 2

Multiply x – y – z by -8x².

Solution

Multiply each term of the polynomial x – y – z by the monomial -8x².
⟹ -8x² * (x – y – z)
= (-8x² * x) – (-8x² *y) – (-8x² * z)

Add the like terms to get;
= -8x³ + 8x²y + 8x²z

Example 3

Multiply 4p³ – 12pq + 9q² by -3pq.

Solution

= 3pq * (4p³ – 12pq + 9q²)

Multiply each term of the polynomial by the monomial
⟹ (-3pq * 4p³) – (-3pq * 12pq) + (-3pq * 9q²)
= 12p⁴q + 36p²q² – 27pq³

Example 4

Find the product of 3x + 5y – 6z and – 5x

Solution

= -5x * (3x + 5y – 6z)

= (-5x * 3x) + (-5x * 5y) – (-5x * 6z)

= -15x² – 25xy + 30xz

Example 5

Multiply x² + 2xy + y² + 1 by z.

Solution

= z * (x² + 2xy + y² + 1)

Multiply each term of the polynomial by the monomial
⟹ (z * x²) + (z * 2xy) + (z * y²) + (z * 1)
= x²z + 2xyz + y²z + z

Multiplying a polynomial by a binomial

Let’s understand this concept with a help of a few examples below.

Example 6

Multiply (a² − 2a) * (a + 2b − 3c)

Solution

Apply the distributive law of multiplication

⟹ a² * (a + 2b − 3c) − 2a * (a + 2b − 3c)

⟹ (a² * a) + (a² * 2b) + (a² * −3c) − (2a * a) − (2a * 2b) − (2a * −3c)

= a³ + 2a²b − 3a²c − 2a² − 4ab + 6ac

Example 7

Multiply (2x + 1) by (3x​² − x + 4)

Solution

Use the distributive property to multiply the expressions;

⟹ 2x (3x² − x + 4) + 1(3x² – x + 4)
⟹ (6x³ − 2x² + 8x) + (3x² – x + 4)

Combine like terms.

⟹ 6x³ + (−2x² + 3x²) + (8x − x) + 4

= 6x³ + x² + 7x + 4

Example 8

Multiply (x + 2y) by (3x − 4y + 5)

Solution

= (x + 2y) * (3x − 4y + 5)

= 3x² − 4xy + 5x + 6xy − 8y² + 10y

= 3x² + 2xy + 5x − 8y² + 10y