Integration of Hyperbolic Functions – Definition, Formulas, and Examples

This article focuses on the integration of hyperbolic functions and the rules established for these unique functions. In the past, we’ve explored their properties, definition, and derivative rules, so it’s fitting that we are allotting a separate article for their integral rules as well.

We can establish the rules for the integration of hyperbolic functions using their derivatives or their definition in terms of exponential functions. This article will show you how hyperbolic functions exhibit similar forms with the integration of trigonometric functions as well.

By the end of our discussion, you should be able to list down the six integral rules for hyperbolic functions and learn how to apply them when integrating hyperbolic expressions. Make sure to have your notes with you on our fundamental integral properties since we’ll also be applying them in this discussion.

How to integrate a hyperbolic function?

We can integrate hyperbolic functions by establishing the two fundamental rules: $\dfrac{d}{dx}\sinh x = \cosh x$ and $\dfrac{d}{dx}\cosh x=\sinh x$.

In the past, we’ve learned about hyperbolic functions and their derivatives, so it’s now time for us to learn how to integrate expressions that contain any of the six hyperbolic functions as well.

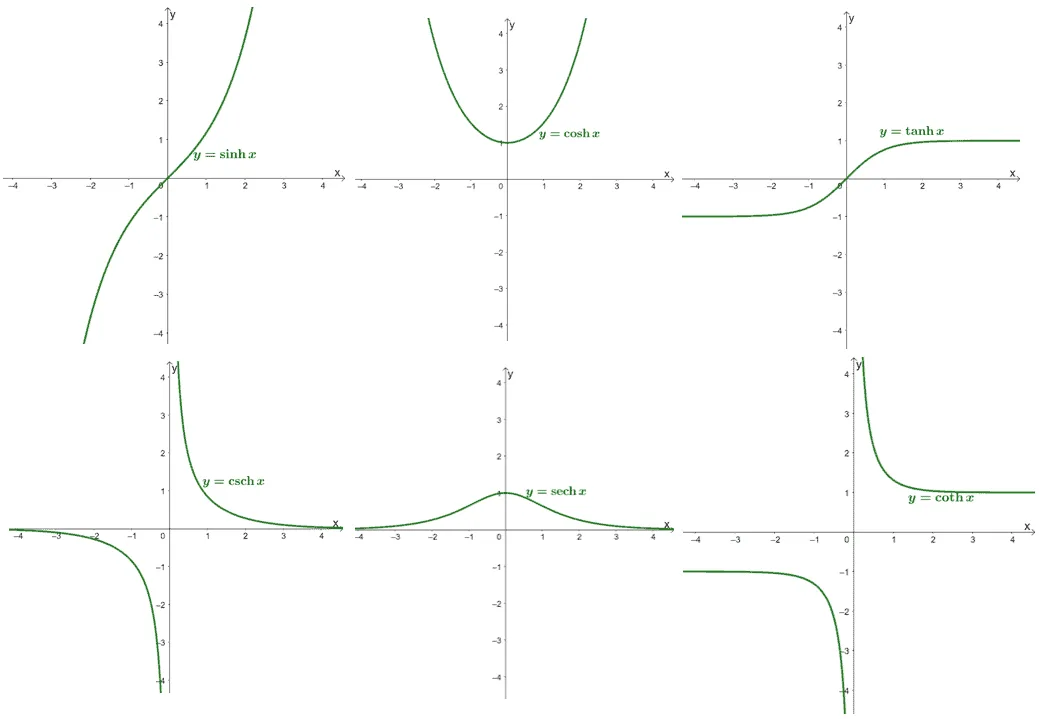
Here are the six graphs of the hyperbolic functions we’ve learned in the past. We can find the integral of $\sinh x$ and $\cosh x$ using their definition in terms of $e^x$:

We can integral this two rational expressions by applying the rules for integrating exponential functions: $\int e^x \phantom{x}dx = e^x + C$. In the past, we’ve also shown that $\int e^{-x} \phantom{x}dx = -e^{-x} +C$. Head over to this article if you want to check the full working out of this integral.

We can use either the derivative rules or the exponential form of the rest of the hyperbolic functions. But no worries, we’ve summarized all six hyperbolic functions’ integration rules as shown below.

We’ve also included their corresponding derivative rule to give you an idea on how each antiderivative formula was derived through the fundamental theorem of calculus. With these rules as well as the antiderivative formulas and integral techniques we’ve learned in the past, we are now equipped to integrate hyperbolic functions.

Below some guidelines on how to use these integral rules to integrate hyperbolic expressions completely:

• Identify the hyperbolic expressions found in the function and take note of their corresponding antiderivative formula.
• If the hyperbolic function contains an algebraic expression within it, apply the substitution method first.
• If the function that needs to be integrated is a product of two simpler functions, use integration by parts only when the substitution method does not apply.

When you’re ready, go ahead and head over to the next section.  Learn how to integrate different types of functions that contain hyperbolic expressions.

Example 1

Evaluate the indefinite integral, $\int x\cosh x^2\phantom{x}dx$.

Solution

Since we’re working with $\cosh (x^2)$, let’s use the substitution method so we can apply the integral rule, $\int \cosh x \phantom{x}dx = \sinh x + C$.

\begin{aligned} u &= x^2 \\du &= 2x \phantom{x}dx\\\dfrac{1}{2x}\phantom{x}du &= dx \end{aligned}

Use these expressions to rewrite the hyperbolic function we’re integrating.

\begin{aligned} \int x\cosh x^2\phantom{x}dx &=\int x \cosh u \cdot \dfrac{1}{2x}\phantom{x}du\\&=\int \dfrac{1}{2} \cosh u\phantom{x}du\\&= \dfrac{1}{2}\int\cosh u \phantom{x}du\\&= dfrac{1}{2}\sinh u + C\end{aligned}

Substitute $u = x^2$ back into the expression. Hence, $\int x\cosh x^2\phantom{x}dx = \dfrac{1}{2}\cosh x^2 +C $.

Example 2

Calculate the integral, $\int \dfrac{\cosh x}{3 + 4\sinh x} \phantom{x}dx$.

Solution

If we take a look at the derivative of the denominator, we have $\dfrac{d}{dx} (3 + 4\sinh x) = 4\cosh x$, so we use the substitution method to cancel the numerator out.

\begin{aligned} u &= 3 + 4\sinh x\\ du &= 4\cosh x \phantom{x}dx\\\dfrac{1}{4 \cosh x} \phantom{x}du &= dx\end{aligned}

If we let $u = 3 + 4\sinh x$, we can cancel out $\cosh x$ once we replace $dx$ with $\dfrac{1}{4 \cosh x} \phantom{x}du$.

\begin{aligned} \int \dfrac{\cosh x}{3 + 4\sinh x} \phantom{x}dx &= \int \dfrac{\cosh x}{u} \phantom{x}\cdot \dfrac{1}{4 \cosh x}\phantom{x}du\\&= \int \dfrac{1}{4}\cdot \dfrac{1}{u}\phantom{x}du\\&=\dfrac{1}{4}\int \dfrac{1}{u}\phantom{x}du \end{aligned}

Use the antiderivative formula, $\int \dfrac{1}{x}\phantom{x} dx = \ln |x| + C$. Rewrite the antiderivative back in terms of $x$ by substituting $u = 3 + 4\sinh x$ back.

\begin{aligned} \dfrac{1}{4}\int \dfrac{1}{u}\phantom{x}du &= \dfrac{1}{4}\ln|u| + C\\&= \dfrac{1}{4}\ln|3 + 4\sinh x| + C \end{aligned}

This means that $\int \dfrac{\cosh x}{3 + 4\sinh x} \phantom{x}dx =\dfrac{1}{4}\ln|3 + 4\sinh x| + C $.

Example 3

Evaluate the indefinite integral, $\int \sinh^2 x \phantom{x}dx$.

Solution

Rewrite $\sinh^2 x$ using the hyperbolic identities, $\cosh^2 x – \sinh^2 x = 1$ and $\cosh 2x = \sinh^2 x + \cosh^2 x$.

\begin{aligned}-\sinh^2 x &= 1 – \cosh^2x\\\sinh^2 x&= \cosh^2x – 1 \\2\sinh^2x&= \sinh^2 x+ \cosh^2x – 1\\2\sinh^2 x&= \cosh 2x – 1\\\sinh^2 &= \dfrac{\cosh 2x – 1}{2}\end{aligned}

Substitute this expression back into our indefinite integral, $\int \sinh^2 x \phantom{x}dx$.

\begin{aligned} \int \sinh^2 x \phantom{x}dx &= \int\dfrac{\cosh 2x – 1}{2} \phantom{x}dx\\&=\dfrac{1}{2}\int (\cosh 2x – 1)\phantom{x}dx\end{aligned}

Apply the substitution method and use $u = 2x \rightarrow du = 2 \phantom{x}dx$. Integrate $\cosh u$ using the integral rule, $\int \cosh u \phantom{x}dx = \sinh x +C$.

\begin{aligned}\dfrac{1}{2}\int (\cosh 2x – 1)\phantom{x}dx &= \dfrac{1}{2}\int (\cosh u – 1) \cdot \dfrac{1}{2}\phantom{x}du\\&= \dfrac{1}{4} \int(\cosh u – 1)\phantom{x} du\\&= \dfrac{1}{4} \left[ \int\cosh u \phantom{x} du- \int 1 \phantom{x} du\right ]\\&= \dfrac{1}{4}(\sinh u – u) + C\\&= \dfrac{1}{4}\sinh u – \dfrac{1}{4}u + C\end{aligned}

Substitute $u =2x$ back into the expression. Hence, we have $\int \sinh^2 x \phantom{x}dx = \dfrac{1}{4}\sinh 2x – \dfrac{1}{2}x + C $.

Example 4

Evaluate the integral, $\int e^x \cosh x\phantom{x}dx$.

Solution

We’re integrating the expression, $e^x \cosh x$, which is the product of two expressions: $e^x$ and $\cosh x$. We can’t apply the substitution method for this expression. Instead, what we’ll do is rewrite $\cosh x$ using its exponential form, $\cosh x = \dfrac{e^x + e^{-x}}{2}$.

\begin{aligned}\int e^x \cosh x\phantom{x}dx &= \int e^x \left(\dfrac{e^{x} + e^{-x}}{2} \right )\phantom{x}dx\\&= \int \left(\dfrac{e^x \cdot e^{x} + e^x \cdot e^{-x}}{2} \right )\phantom{x}dx \\&= \int \dfrac{e^{2x} + e^{0}}{2}\phantom{x} dx\\&= \int \dfrac{1}{2} (e^{2x} + 1)\phantom{x}dx\end{aligned}

We can then let $u$ be $2x$ and apply the substitution method as shown below.

\begin{aligned}u&= 2x\\du &= 2 \phantom{x}dx\\\dfrac{1}{2}\phantom{x}du &= dx\\\\ \int \dfrac{1}{2} (e^{2x} + 1)\phantom{x}dx &= \int \dfrac{1}{2}(e^u + 1) \cdot \dfrac{1}{2}\phantom{x}du\\&= \dfrac{1}{4}\int(e^u + 1) \phantom{x}du\end{aligned}

Evaluate the new integral expression by applying the sum rule and exponential rule, $\int e^x \phantom{x} dx = e^x + C$.

\begin{aligned}\dfrac{1}{4}\int(e^u + 1) \phantom{x}du &= \dfrac{1}{4}\left(\int e^u \phantom{x}du + \int 1 \phantom{x}du \right)\\&= \dfrac{1}{4}(e^u + u) + C\end{aligned}

Substitute $u = 2x$ back into the expression so that we have our antiderivative in terms of $x$.

\begin{aligned}\dfrac{1}{4}(e^u + u) + C &=\dfrac{1}{4}(e^{2x} + 2x) + C\\&= \dfrac{e^{2x}}{4} + \dfrac{x}{2} + C\end{aligned}

This means that $\int e^x \cosh x\phantom{x}dx =\dfrac{e^{2x}}{4} + \dfrac{x}{2} + C $.

Example 5

Find the integral of $\int \tanh 3x\phantom{x}dx$.

Solution

We have no integral rule for $\int \tanh x \phantom{x}dx $ or $\int \tanh 3x \phantom{x}dx$, so what we can do is express $\tanh 3x$ as $\dfrac{\sinh 3x}{\cosh 3x}$. Hence, we have

\begin{aligned}\int \tanh 3x\phantom{x}dx &= \int \dfrac{\sinh 3x}{\cosh 3x} \phantom{x}dx \end{aligned}

Use $u =  \cosh 3x$ then apply the substitution method as shown below.

\begin{aligned}u &= \cosh 3x \\du &= 3 \sinh x \phantom{x}dx\\\dfrac{1}{3\sinh 3x} \phantom{x}du &= dx\\\\\int \dfrac{\sinh 3x}{\cosh 3x} \phantom{x}dx &= \int\dfrac{\sinh 3x}{u} \cdot\dfrac{1}{3\sinh 3x} \phantom{x}du\\&=\dfrac{1}{3}\int \dfrac{1}{u} \phantom{x}du\end{aligned}

Apply the integral rule, $\int \dfrac{1}{x}\phantom{x}dx = \ln |x| + C$, then substitute $u = \cosh 3x$ back into the resulting expression.

\begin{aligned}\dfrac{1}{3}\int \dfrac{1}{u} \phantom{x}du &= \dfrac{1}{3}\ln |u| + C\\&= \dfrac{1}{3}\ln|\cosh 3x| + C\end{aligned}

Hence, we have $\int \tanh 3x\phantom{x}dx = \dfrac{1}{3}\ln|\cosh 3x| + C $.

Example 6

Evaluate the definite integral, $\int_{0}^{1} -2x \sinh x\phantom{x}dx$.             

Let’s disregard the upper and lower limits for now and find the antiderivative of $-2x \sinh x $ first. Factor out $-2$ from the integral then integrate the resulting expression by parts.

\begin{aligned}\int -2x \sinh x\phantom{x}dx &= -2\int x \sinh x\phantom{x}dx \end{aligned}

Now, it’s time to assign which would best be $u$ and $dv$. 

Apply the formula, $\int u \cdot dv = uv – \int v \cdot du$, to integrate our expression by parts.

\begin{aligned}\int u \cdot dv &= uv – \int v \cdot du\\\\-2\int x\sinh x \phantom{x}dx &= -2\left[x\cosh x – \int \cosh x\phantom{x}dx \right ]\\&= -2(x \cosh x – \sinh x) + C\\&= -2x\cosh x + 2\sinh x + C\end{aligned}

Evaluate this antiderivative at $x = 0$ and $x = 1$ to find $\int_{0}^{1} -2x \sinh x\phantom{x}dx$. Keep in mind that $\sinh 0 = 0$.

\begin{aligned}\int_{0}^{1} -2x \sinh x\phantom{x}dx &= -2x\cosh x + 2\sinh x|_{0}^{1}\\&= (-2x\cosh 1 + 2\sinh 1) – (-2(0)\cosh x + 2\sinh 0)\\&= -2\cosh 1 + 2\sinh 1 \end{aligned}

We can further simplify the expression using the exponential forms of $\sinh x$ and $\cosh x$.

\begin{aligned}-2\cosh 1 + 2\sinh 1 &= -2\cdot\dfrac{e^1 + e^{-1}}{2} +2\cdot\dfrac{e^1 – e^{-1}}{2} \\&= -\dfrac{1}{e}-\dfrac{1}{e}\\&=-\dfrac{2}{e}\end{aligned}

Hence, we have $\int_{0}^{1} -2x \sinh x\phantom{x}dx =-\dfrac{2}{e}$.

Practice Questions

1. Evaluate the indefinite integral, $\int x^2 \sinh x^3\phantom{x}dx$.
2. Calculate the integral, $\int \dfrac{2\sinh x}{5 + 6\cosh x} \phantom{x}dx$.
3. Evaluate the indefinite integral, $\int \cosh^2 x \phantom{x}dx$.
4. Calculate the integral, $\int 4e^x \sinh x\phantom{x}dx$.
5. Evaluate the indefinite integral, $\int \text{coth} \dfrac{x}{6} \phantom{x}dx$.
6. Calculate the definite integral, $\int_{0}^{1} -\dfrac{3x}{2} \cosh x\phantom{x}dx$.

Answer Key

1. $\int x^2 \sinh x^3\phantom{x}dx = \dfrac{1}{3} \cosh x^3 + C$
2. $\int \dfrac{2\sinh x}{5 + 6\cosh x} \phantom{x}dx = \dfrac{1}{3}\ln|5 + 6\cosh x| + C$
3. $\int \cosh^2 x \phantom{x}dx = \dfrac{1}{4} \sinh 2x + \dfrac{1}{2}x + C$
4. $\int 4e^x \sinh x\phantom{x}dx = e^{2x} – 2x + C$
5. $\int \text{coth} \dfrac{x}{6} \phantom{x}dx = 6\ln \left|\sinh \dfrac{x}{6}\right| + C$
6. $\int_{0}^{1} -\dfrac{3x}{2} \cosh x\phantom{x}dx = \dfrac{3 – 3e}{2e} \approx -0.948$