Integrating Exponential Functions – Formulas, Process, and Examples

In this article, we’ll master the techniques needed in integrating exponential functions. We’ve learned that exponential functions are essential in modeling population growth, cell growth, radioactive decay, and other significant applications. This means that it’s equally essential for us to know how to measure the changes occurring within the exponential functions.

When integrating exponential functions, we start from the most fundamental rules: the antiderivative of $\boldsymbol{e^x}$ is $\boldsymbol{e^x}$ itself and $\boldsymbol{a^x}$ is simply the $\boldsymbol{a^x}$ divided by the constant, $\boldsymbol{\ln a}$.

We’ll explore different types of exponential functions and learn how to apply other techniques to completely integrate the function. This is why having your notes on integral properties, antiderivative formulas, and other integral techniques will come in handy in our discussion.

What is the integration of exponential function?

Exponential functions’ integrals are very interesting since we still end up with the function itself or a variation of the original function. Our most fundamental rule when integrating exponential functions are as follows:

Understanding $\boldsymbol{\int e^x \phantom{x}dx = e^x +C}$

The derivative of the exponential function,$e^x$, is simply $e^x$ itself. We can apply the fundamental theorem of calculus to confirm the integral rule for $e^x$.

\begin{aligned}\int e^x \phantom{x}dx &= \int \dfrac{d}{dx} (e^x) \phantom{x}dx\\&= e^x +C\end{aligned}

This means that similar to its derivative rule, the antiderivative of $\boldsymbol{e^x}$ is also $\boldsymbol{e^x}$ as well.

Understanding $\boldsymbol{\int a^x \phantom{x}dx = \dfrac{a^x}{\ln a} +C}$

Recall that the derivative of $a^x$ is equal to $a^x \ln a$. Rewrite this rule to express $a^x$ as a derivative of a function.

\begin{aligned}\dfrac{d}{dx} a^x &= a^x \ln a\\ \dfrac{1}{\ln a}\dfrac{d}{dx} a^x &= a^x\\\dfrac{d}{dx}\left(\dfrac{a^x}{\ln a} \right ) &=a^x\end{aligned}

Integrate both sides of the equation then apply the fundamental theorem of calculus to simplify the expression.

This confirms that the integral of $a^x$ is equal to $\boldsymbol{a^x}$ itself divided by the constant, $\boldsymbol{\ln a}$.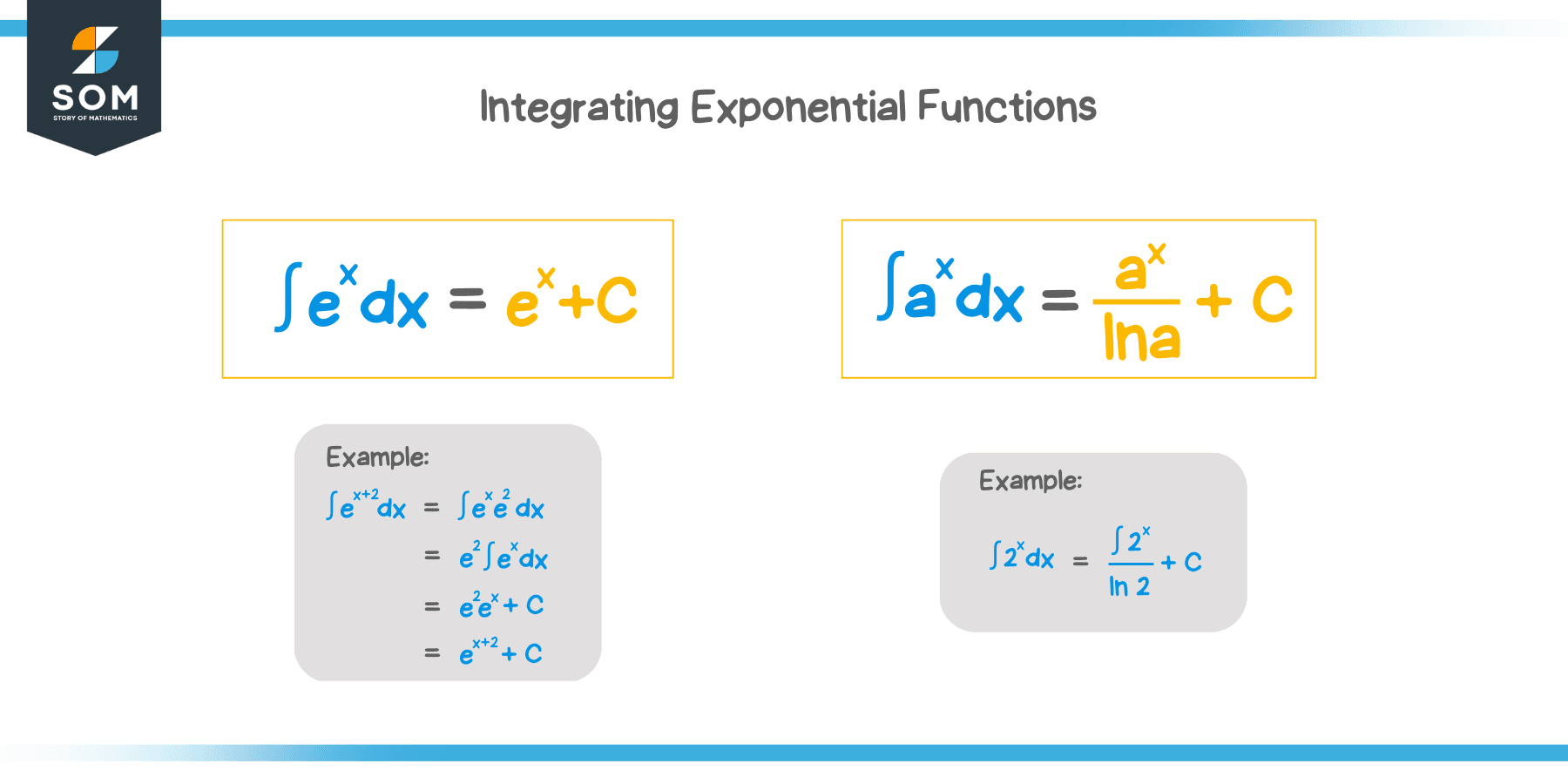

These are the two formulas we can use when integrating exponential functions. When integrating more complex functions, we’ll have to use other integration techniques including the substitution method and integration by parts.

How to integrate exponential functions?

Integrating exponential functions is also a test of our understanding of the integration techniques we’ve learned in the past. Here are some helpful pointers to keep in mind when integrating complex functions.

• Identify whether we’re working with an exponential function with a positive constant as a base or if we have $e$ as the base.
• Use the right formula to integrate the function.
• When the exponent of $a$ or $e$ has a coefficient before $x$ or is an expression in terms of $x$, see if the substitution method applies.
• If the exponential function is multiplied to another expression, check if the substitution method applies first then only use integration by parts as the last option.

Integrating exponential function using the substitution method:

Let us show you how to integrate the expression, $y = e^{-x}$.

\begin{aligned}\int e^{-x}\phantom{x}dx\end{aligned}

The exponential function has a base of $e$, so we use the integral formula, $\int e^x\phantom{x}dx = e^x +C$. Since the exponent has $-1$ before $x$, we’ll need to use the substitution method to integrate the expression.

\begin{aligned}u &= -x \\du &= -1 \cdot dx\\ -du &= dx\end{aligned}

Rewrite $\int e^{-x}\phantom{x}dx$ in terms of $u$ and $du$.

\begin{aligned}\int e^{-x}\phantom{x}dx &= \int u \cdot (-du)\\&= -\int e^u \phantom{x}du\end{aligned}

Apply the integral formula for exponential functions with base, $e$, then substitute $u = -x$ back into the expression.

\begin{aligned}-\int e^u \phantom{x}du &= – (e^u) + C\\&=-e^u + C\\&= -e^{-x} + C\end{aligned}

Hence, through substitution method, we have $\int e^{-x}\phantom{x}dx = -e^{-x} + C$.

Integrating exponential function using integration by parts:

Review what we know of integration by parts to find the antiderivative of $y = x2^x$.

\begin{aligned} \int x2^x \phantom{x}dx\end{aligned}

We use $u = x$ and $dv = 2^x \phantom{x}dx$, so we’ll need to integrate $2^x$ using the integral formula, $\int a^x \phantom{x}dx =\dfrac{a^x}{\ln a} +C$.

Apply the integration by parts formula, $\int u \phantom{x}dv = uv- \int v\phantom{x}du$, to integrate the expression.

\begin{aligned}\int x 2^x\phantom{x}dx &= x\cdot \dfrac{2^x}{\ln 2} – \int \dfrac{2^x}{\ln 2} \phantom{x}dx\end{aligned}

Simplify the expression further by integrating the second term of the right-hand side of the equation. Factor the constant, $\dfrac{1}{\ln 2}$, then apply the same integral formula to integrate $2^x$.

\begin{aligned}\int x 2^x\phantom{x}dx &= x\cdot \dfrac{2^x}{\ln 2} -\dfrac{1}{\ln 2}  \int 2^x\phantom{x}dx \\&= \dfrac{2^xx}{\ln x} – \dfrac{1}{\ln 2} \left(\dfrac{2^x}{\ln 2} \right ) + C\\&= \dfrac{2^xx}{\ln 2} – \dfrac{2^x}{(\ln 2)^2} + C\end{aligned}

Hence, through integration by parts and the integral formula for $a^x$, we have $\int x2^x \phantom{x}dx = \dfrac{2^xx}{\ln 2} – \dfrac{2^x}{(\ln 2)^2} + C $.

Keep in mind that we only use this method when the substitution method can’t be applied to the expression we want to integrate. These are just two of the numerous exponential functions we can integrate using what we already know. This is why we’ve prepared more examples for you to work on and included a word problem. When you’re ready, head over to the section below.

Example 1

Evaluate the indefinite integral, $\int xe^{x^2 – 2}\phantom{x}dx$.

Solution

It may be tempting to integrate this by parts since it’s a product of two expressions, but it’ll be much faster and convenient if we always give the substitution method a try when the exponent is also an algebraic expression.

\begin{aligned}u &= x^2 – 2\\du &= 2x \phantom{x}dx\\\dfrac{1}{2x}du &= dx\end{aligned}

Use $ u = x^2 -2$ and $ dx = \dfrac{1}{2x} du$ then simplify the resulting expression. Use the integral formula for $e^x$: $\int e^x \phantom{x}dx = e^x +C$.

\begin{aligned}\int xe^{x^2 – 2}\phantom{x}dx&= \int x e^{u} \cdot \ dfrac{1}{2x}\phantom{x}du\\&=\int \dfrac{1}{2}e^u \phantom{x}du\\&= \dfrac{1}{2}\int e^u \phantom{x}du\\&=\dfrac{1}{2} e^u + C \end{aligned}

Substitute $u = x^2 -2$ back into the expression. Hence, we have $\int xe^{x^2 – 2}\phantom{x}dx = \dfrac{1}{2} e^{x^2 -2} + C $.

Example 2

Evaluate the definite integral, $\int_{0}^{1} \dfrac{2^x + 3^x}{7^x} \phantom{x}dx$.

Solution

Let’s rewrite the expression, $\dfrac{2^x + 3^x}{7^x}$, as shown below.

\begin{aligned}\dfrac{2^x + 3^x}{7^x} &= \dfrac{2^x}{7^x} + \dfrac{3^x}{7^x}\\&= \left(\dfrac{2}{7} \right )^x + \left(\dfrac{3}{7}\right)^x\end{aligned}

Use this expression instead then integrate the definite integral.

\begin{aligned}\int_{0}^{1} \dfrac{2^x + 3^x}{7^x} \phantom{x}dx&= \int_{0}^{1} \left[\left(\dfrac{2}{7} \right )^x + \left(\dfrac{3}{7}\right)^x \right ]\phantom{x}dx \end{aligned}

Distribute the definite integral operation then use the formula, $\int a^x \phantom{x}dx = \dfrac{a^x}{\ln a} +C$, where $a = \dfrac{2}{7}$ for the first integral and $a = \dfrac{3}{7}$ for the second integral.

\begin{aligned}\int_{0}^{1} \left(\dfrac{2}{7} \right )^x \phantom{x}dx + \int_{0}^{1}\left(\dfrac{3}{7}\right)^x \phantom{x}dx&= \left[\dfrac{\dfrac{2}{7}^x}{\ln (2/7)} + \dfrac{\dfrac{3}{7}^x}{\ln (3/7)}\right ]_{0}^{1}\\&=\left[\dfrac{\dfrac{2}{7}^1}{\ln (2/7)} + \dfrac{\dfrac{3}{7}^1}{\ln (3/7)} \right ] – \left[\dfrac{\dfrac{2}{7}^0}{\ln (2/7)} + \dfrac{\dfrac{3}{7}^0}{\ln (3/7)} \right ]\\&= \dfrac{\dfrac{2}{7} -1}{\ln (2/7)} – \dfrac{\dfrac{3}{7} -1}{\ln (3/7)}\\&= -\dfrac{5}{7\ln (2/7)}  -\dfrac{4}{7\ln (3/7)}\end{aligned}

Hence, we have $\int_{0}^{1} \dfrac{2^x + 3^x}{7^x} \phantom{x}dx =-\dfrac{5}{7\ln (2/7)}  -\dfrac{4}{7\ln (3/7)}$.

Example 3

Determine the antiderivative of the function, $5^x \sqrt{1 + 5^x}$.

Solution

Apply the substitution method by letting $u$ as $1 + 5^x$ then find the expressions of $u$ and $dx$ in terms of $du$.

\begin{aligned}u &= 1 + 5^x\\ du &= 5^x \ln 5 \phantom{x}dx\\\dfrac{1}{5^x \ln 5} \phantom{x}du &= dx\end{aligned}

Rewrite $\int 5^x \sqrt{1 + 5^x} \phantom{x}dx$ using $u = 1 + 5^x$ and $dx = \dfrac{1}{5^x \ln 5} \phantom{x}du$.

\begin{aligned}\int 5^x \sqrt{1 + 5^x} \phantom{x}dx &= \int 5^x \sqrt{u} \cdot \dfrac{1}{5^x \ln 5} \phantom{x}du\\&= \dfrac{1}{\ln 5} \int \sqrt{u} \phantom{x}du\end{aligned}

Use the fact that $\sqrt{u} = u^{\frac{1}{2}}$ then apply the power rule, $\int x^n \phantom{x}dx  =\dfrac{x^{n + 1}}{n + 1} +C$, to integrate the expression completely.

\begin{aligned}\dfrac{1}{\ln 5} \int \sqrt{u} \phantom{x}du &= \dfrac{1}{\ln 5}\int u^{\frac{1}{2}} \phantom{x}du\\&= \dfrac{1}{\ln 5} \cdot \dfrac{u^{\frac{1}{2}+ 1}}{\frac{1}{2}+ 1} +C\\&= \dfrac{1}{\ln 5}  \cdot \dfrac{u^{\frac{3}{2}}}{3/2} + C\\ &= \dfrac{2u^{\frac{3}{2}}}{3\ln 5} + C\end{aligned}

Substitute the expression, $u = 1 + 5^x$, back into the resulting antiderivative.

\begin{aligned}\dfrac{2u^{\frac{3}{2}}}{3\ln 5} + C &= \dfrac{2 (1 + 5^x)^{\frac{3}{2}}}{3\ln 5} + C\end{aligned}

This means that $\int 5^x \sqrt{1 + 5^x} \phantom{x}dx = \dfrac{2 (1 + 5^x)^{\frac{3}{2}}}{3\ln 5} + C $.

Example 4

Jane is observing the rate of growth of bacteria in a Petri dish. Her observation led her to modelling the rate of growth as $b(t) = {2}^{t}$, where $t$ in hours and $b(t)$ is measured in thousands of bacteria per hour. If Jane is observing a culture containing $20000$ bacteria, determine the function, $B(t)$ that returns the number of bacteria in the Petri dish for any given time, $t$. Find the number of bacteria that can be observed in the dish after $3$ hours.

Solution

Jane is has modelled the bacteria’s rate of growth is $b(t) = 2^t$. This defines the rate of change of $B(t)$ over the time, $t$, in hours. This means that $B(t) = \int b(t)\phantom{x} dt$.

Find $B(t)$ by integrating $b(t) = 2^t$ then use the initial value, $B(0) = 20 $ thousands of bacteria, to find $C$. Integrate $b(t)$ using the integral formula, $\int a^x \phantom{x}dx = \dfrac{a^x}{\ln a} + C$.

\begin{aligned}B(t) &= \int b(t) \phantom{x}dt\\&= \int 2^t \phantom{x}dt\\&= \dfrac{2^t}{\ln 2} + C\\\\B(0) &= 20\\\dfrac{2^0}{\ln 2} + C &= 20\\C &= 20- \dfrac{1}{\ln 2}\\\ &\approx 18.557\end{aligned}

Hence, we have $B(t) =  \dfrac{2^t}{\ln 2} + 18.557$. This means that after $t$ hours, there’s $B(t)$ thousands of bacteria left in the Petri dish. To find the amount of bacteria left after $3$ hours, substitute $t = 3$ into the expression.

 \begin{aligned}B(3) &= \dfrac{2^3}{\ln 2} + 18.557\\&\approx 30.099\end{aligned}

This means that after $3$ hours, Jane should expect approximately $30, 099$ bacteria in the Petri dish.

Practice Questions

1. Find the antiderivatives of the following functions:
a. $\int xe^{x^2 + 4}\phantom{x}dx$
b. $\int (x^2 – 1)e^{x^3 – 3x}\phantom{x}dx$
c. $\int (3x^2 + 6)e^{x^3 + 6x}\phantom{x}dx$

2. Evaluate the following definite integrals:
a. $\int_{0}^{1} \dfrac{3^x + 6^x}{7^x} \phantom{x}dx$
b. $\int_{\ln 2}^{\ln 8} \dfrac{3e^x – 5}{4} \phantom{x}dx$
c. $\int_{0}^{e} \dfrac{e^{x} – 6^x}{5} \phantom{x}dx$

3. Find the antiderivatives of the following functions:
a. $\int 3^x \sqrt{1 + 3^x} \phantom{x}dx$
b. $\int e^x \sqrt{1 + e^x} \phantom{x}dx$
c. $\int 2^x\sqrt[3]{1 + 2x} \phantom{x}dx$

4. Alexander is observing the rate of growth of bacteria in a Petri dish. His observation led him to modelling the rate of growth as $b(t) = {3}^{t}$, where $t$ in hours and $b(t)$ is measured in thousands of bacteria per hour. If Alexander is observing a culture containing $40000$ bacteria, determine the function, $B(t)$ that returns the number of bacteria in the Petri dish for any given time, $t$. Find the number of bacteria that can be observed in the dish after $4$ hours.

5. Jack observed that the population of fruit flies increases at a rate of $g(t)= 3 e^{0.03t}$, in flies per day. Jack noted that the initial population is $150$ flies. How many flies should Jack expect to observe after $12$ days?

Answer Key

1.
a. $\int xe^{x^2 + 4} \phantom{x}dx = \dfrac{1}{2}e^{x^2 + 4} +C$
b. $\int (x^2 – 1)e^{x^3 – 3x} \phantom{x}dx = \dfrac{1}{3}e^{x^3 – 3x} +C$
c. $\int (3x^2 + 6)e^{x^3 + 6x} \phantom{x}dx = e^{x^3 + 6x} + C$
2.
a. $\int_{0}^{1} \dfrac{3^x + 6^x}{7^x} \phantom{x}dx = -\dfrac{4}{7\ln \left(\dfrac{3}{7}\right)} – \dfrac{1}{7\ln \left(\frac{6}{7}\right)}$
b. $\int_{\ln 2}^{\ln 8} \dfrac{3e^x – 5}{4} \phantom{x}dx = \dfrac{1}{2} (9 – 5\ln 2)$
c. $\int_{0}^{e} \dfrac{e^{x} – 6^x}{5} \phantom{x}dx = \dfrac{1}{5} \left(e^e -1 -\dfrac{6^e – 1}{\ln 6}\right)$
3.
a. $\int 3^x \sqrt{1 + 3^x} \phantom{x}dx =\dfrac{2 (1 + 3^x)^{\frac{3}{2}}}{3\ln 3} + C $
b. $\int e^x \sqrt{1 + e^x} \phantom{x}dx = \dfrac{2}{3}(1 + e^x)^{\frac{3}{2}} + C$
c. $\int 2^x\sqrt[3]{1 + 2x} \phantom{x}dx = =\dfrac{3 (1 + 2^x)^{\frac{4}{3}}}{4\ln 2} + C $

4. $112, 819$
5.
$\begin{aligned} G(12) &= G(0) +\int_{0}^{12}3 e^{0.03t} \phantom{dt}\\ &\approx 193\end{aligned}$