How To Find Square Root of 9/16: Examples and Explanation

The square root of 9/16 is $\dfrac{3}{4}$.

Solving the square root of a fraction can seem complex initially, but once you get the hang of it, it will be much easier. In mathematics, it is always convenient to divide complex problems into smaller parts before solving them.

This guide will help you break down complex fractions, and you will learn how to calculate the square root of the fraction by dividing the primary expression into smaller parts. We will mainly focus on calculating the square root of $\dfrac{9}{16}$, but in doing so, we will discuss how to calculate the square root of any given fraction.

What Is the Square Root of a Fraction?

The first step when solving for the square root of a fraction involves solving the square root of the number given as the numerator, the second step involves taking the square root of the number provided in the denominator, and the final step consists of the division of the numerator and denominator to get the final answer. The square root of a fraction is easy to find, but to solve the fraction square root, we have to solve it by parts.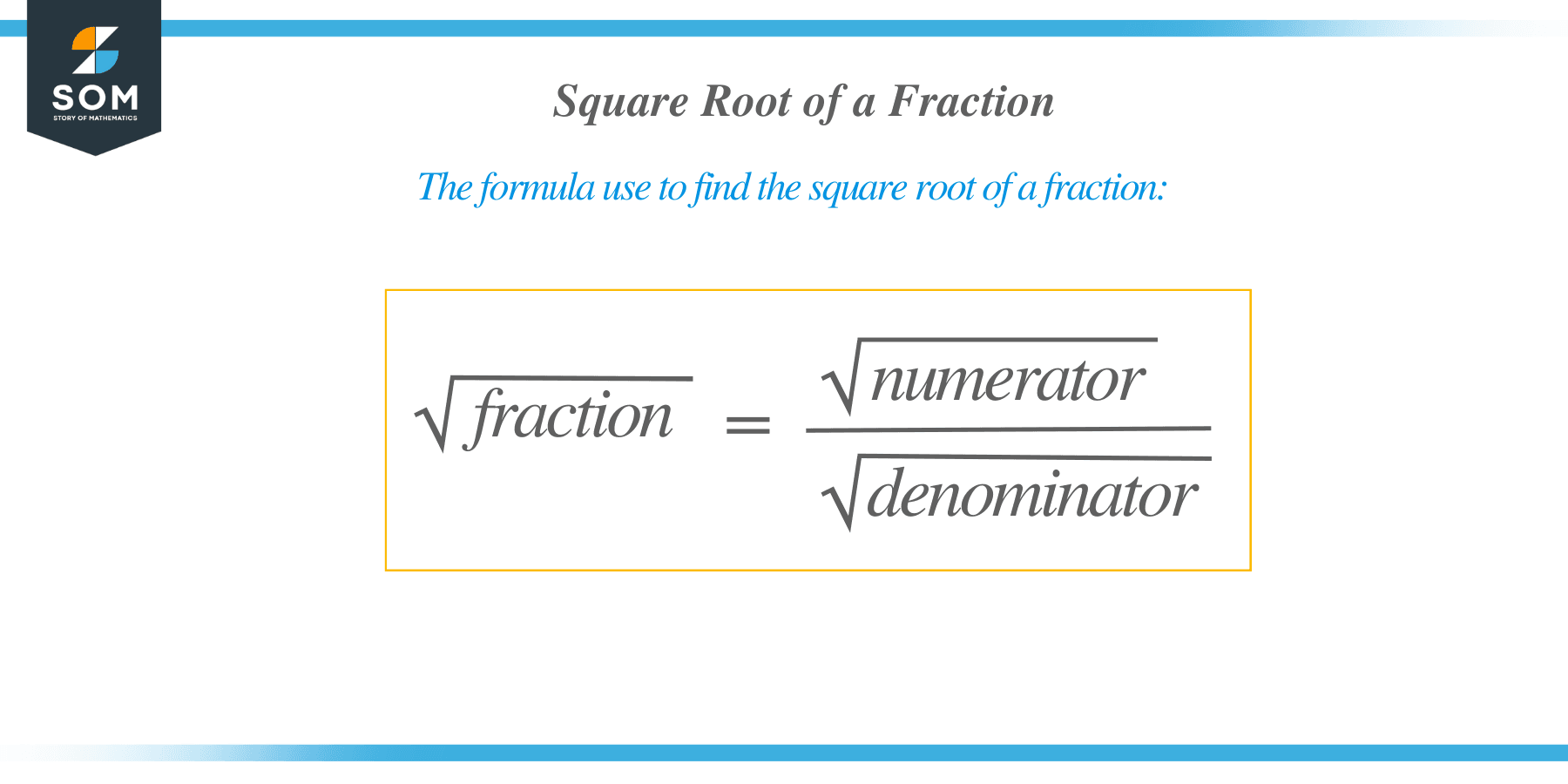

How To Calculate Square Root of 9/16

To calculate the square root of $9/16$, you will have to follow the steps given below.

Conversion to Fraction

To solve the square root $\dfrac{9}{16}$ it is essential that the number is denoted in a proper fraction form instead of any other form. For example, the fraction $\dfrac{9}{16}$ has an equivalent decimal notation 0.5625. The number $\dfrac{9}{16}$ may be written as

1. $\dfrac{9}{16}$= $0.5625$

2. $56.25$ %

So, to determine the square root of a fraction, it is important that the fraction with the correct numbers is given. Otherwise, we would first need to convert the given number to a proper fraction. The numerator is given in the fraction’s upper or top part. The bottom of the fraction contains the denominator.

Square Root of Numerator

The second step involves taking the square root of the numerator of the fraction and calculating the square root. We have to find the factors of the numerator and see if it is a perfect square. The answer for the perfect square will always be a whole number, and no decimal will be involved.

To find the factor, we have to find numbers that, if multiplied by themselves, give us the numerator of the fraction. In our case, the number is $9$. The number $9$ can be written as $9 = 3 \times 3 = 3^{2}$. So if we take the square root of $9$, it can be written as:

$\sqrt{9} = \sqrt{3\times 3}= \sqrt{3^{2}}= 3$

Square Root of Denominator

The third step is to calculate or find the square root of the denominator of the fraction, so we have to calculate the square root of 16. Just like in the previous step, we will find the factors for the number given in the denominator, and in this example, the number is 16. Hence, the factors of $16$ are $16 = 4 \times 4$. So the square root of $16$ is:

$\sqrt{16} = \sqrt{4\times 4}= \sqrt{4^{2}}= 4$

Division of Numerator and Denominator

The fourth and final step involves the division of the square root of the numerator with the square root of the denominator. For example, in this scenario, the square of 9 is 3, while the square root 16 is 4, so the final answer in the fraction form will be $\dfrac{3}{4}$. If we want the square root of 9/16 simplified to a mixed number, it can be written as 0.75 or 75%.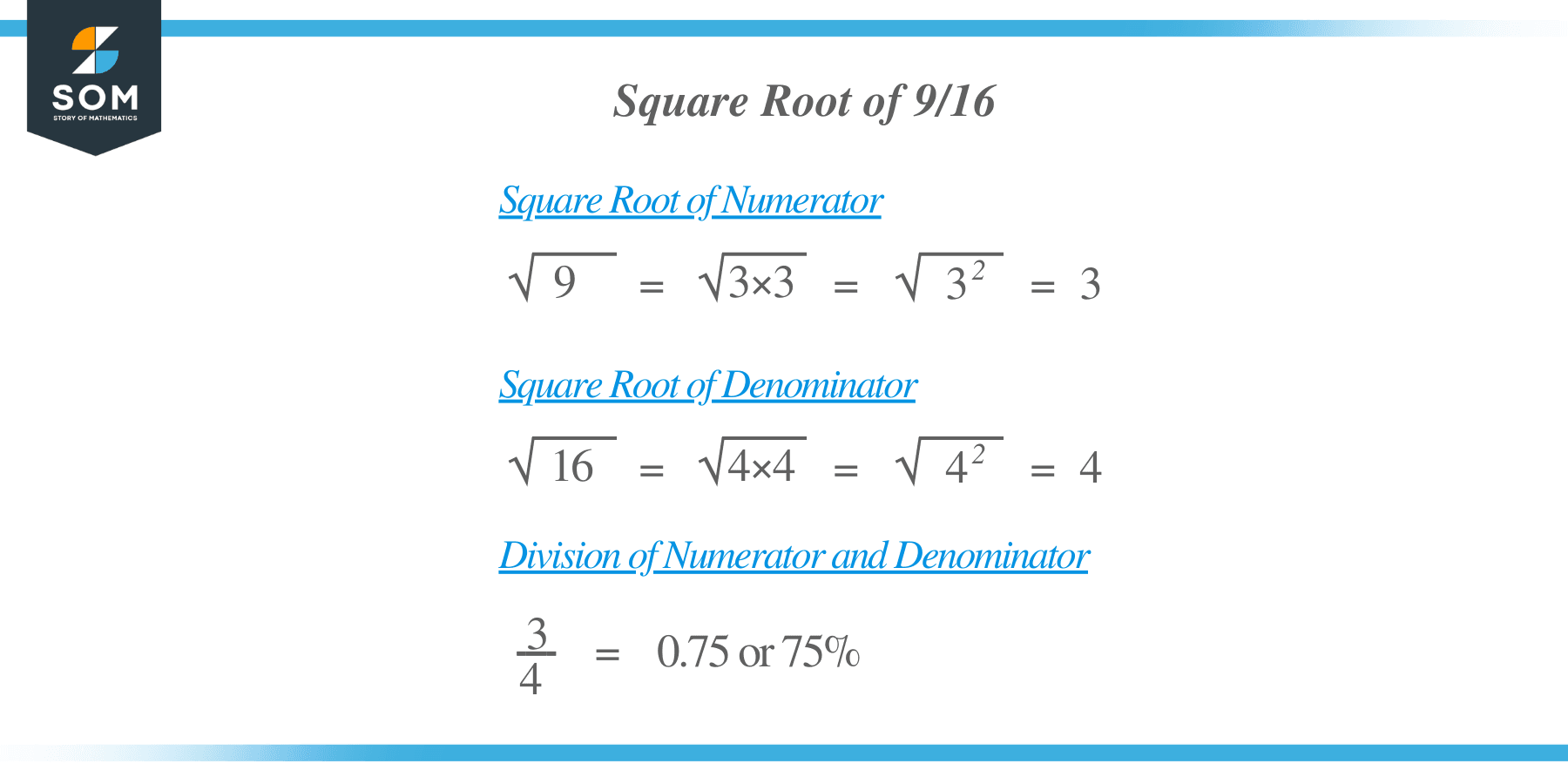

Example 1

Find the area of the square

Solution:

The area of the square = a \times a

$= \sqrt{\dfrac{3}{4}}.\sqrt{\dfrac{3}{4}}$

$= [\sqrt{\dfrac{3}{4}}]^{2} = \dfrac{3}{4}$

Example 2

Find the area of the square

Solution:

The area of the square = a \times a

$= \sqrt{\dfrac{9}{16}}.\sqrt{\dfrac{9}{16}}$

$= [\sqrt{\dfrac{9}{16}}]^{2} = \dfrac{9}{16}$

Example 3

Find the square root of $\dfrac{64}{25}$

Solution:

For the given numerical expression, the numerator is $64$ while the denominator is $25$. First step is to calculate the square root of the numerator. So let us find the factors of $64$. The factors of $64 = 8 \times 8$. So, the square root of $64$ is $\sqrt{64}= \sqrt {8\times 8}= \sqrt{8^{2}} = 8$.

The second step involves the square root of denominator, so we have to find the factors of $25$. The factors of $25$ can be written as $25 = 5 \times 5$. So the square root of $25 \sqrt{25}= \sqrt {5\times 5} = \sqrt{5^{2}} = 5$. The final step involves division of the numerator and denominator $\dfrac{8}{5} = 1.6$

Example 4

Find the square root of $\dfrac{49}{4}$

Solution:

The numerator is $49$ while the denominator is $4$. First step is to calculate the square root of the numerator, so let us find the factors of $49$. The factors of $49 = 7 \times 7$. So the square root of $49$ is $\sqrt{49}= \sqrt {7\times 7} = \sqrt{7^{2}} = 7$.

The second step involves the square root of denominator, so we have to find the factors of $4$. The factors of $4$ can be written as $4 = 2 \times 2$. So the square root of $4 \sqrt{4}= \sqrt {2\times 2} = \sqrt{2^{2}} = 2$. The final step involves division of the numerator and denominator $\dfrac{7}{2} = 3.5$

Example 5

Find the square root of $\dfrac{100}{81}$

Solution:

For the given numerical expression, the numerator is $100$ while the denominator is $81$. First step is to calculate the square root of the numerator. So let us find the factors of $100$. The factors of $100 = 10 \times 10$. So the square root of $100$ is $\sqrt{100}= \sqrt {10\times 10} = \sqrt{10^{2}} = 10$.

The second step involves the square root of denominator, so we have to find the factors of $81$. The factors of $81$ can be written as $81 = 9 \times 9$. So the square root of $81 \sqrt{81}= \sqrt {9\times 9} = \sqrt{9^{2}} = 9$. The final step involves division of the numerator and denominator $\dfrac{10}{9} = 1.1$

Tips for Solving the Square Root of a Fraction

Let us discuss some tips you can use while trying to solve problems related to the square root of the fractions.

Sheer Practice

It is essential to keep practicing different numerical problems related to the square root of fractions, as solving different types of problems will increase your mathematics skills and you will gain experience in solving fraction problems.

You can practice solving the square root of rational numbers, and you can even use the online tools to get you going and jump on to more complex numerical problems. Solving such problems will also increase your basic mathematics techniques, and you will feel more confident in tackling complex fractions-related problems.

Seek Help

If you are finding it difficult to understand the concept of factors and square roots or you are finding it difficult to solve the problems related to fractions, you can always ask your friends, teachers, or colleagues. Solving complex square root fraction problems can sometimes become tricky, so asking for help is not a bad option. You can also study our article related to solving radical fractions.

Re-Check Your Work

Whenever you are solving any problem involving taking the square root of a fraction, it is a good practice to re-check your work to ensure that you have solved the problem correctly. One of the great ways to re-check your work is to use the calculator, as you can solve various square roots of fractions using a calculator. If your answer does not match the calculator’s answer, then you have to go back and find the mistake and correct it.

The second way to re-check your answer is to perform the same calculation again, and if you have ample time, you can do the same calculation thrice and see if you get the same answer. If your answer does not match, then there is a mistake, and you have to identify the mistake and correct it. This is a good practice and will help you solve all kinds of mathematical problems.

Examples

What Is the Square Root of 9/225 as a Fraction?

Answer: The square root of $\dfrac{9}{225}$ is $\dfrac{3}{25} = 0.12$

What Is the Square Root of 9/100?

Answer: The square root of $\dfrac{9}{100}$ is $\dfrac{3}{100} = 0.03$

What Is the Square Root of 9/31?

Answer: The square root of $\dfrac{9}{31}$ is $\dfrac{3}{\sqrt{31}} = 0.54$

Is Square Root of 9/16 Rational or Irrational?

Answer: The square root of $9/16$ can be written in the $\dfrac{p}{q}$ form; hence it is a rational number

Conclusion

Let us conclude our discussion and summarize what we have learned so far.

• To find the square root of a fraction, we use the following formula:

$\sqrt{fraction} = \dfrac{\sqrt{ numerator }}{\sqrt{ denominator }}$

• First, calculate the square root of the numerator. The numerator is the number given at the top of the fraction.

• Second, calculate the square root of the denominator. The denominator is the number that is given at the bottom of the fraction.

• Divide both values found by solving the square root in the first two steps.

Understanding the concept of finding the square of $\dfrac{9}{16}$ and, more importantly, finding the square root of any given fraction should be much easier for you now that you have studied our guide in detail.