JUMP TO TOPIC
Term|Definition & Meaning
Definition
A term is any single number or variable. An expression contains two or more terms. If two numbers or variables or both are multiplied by each other, we consider them as a single term. Since division can be converted into multiplication and vice versa, division also does not separate terms. Therefore, in an equation, terms are only separated by the +, -, and = signs.
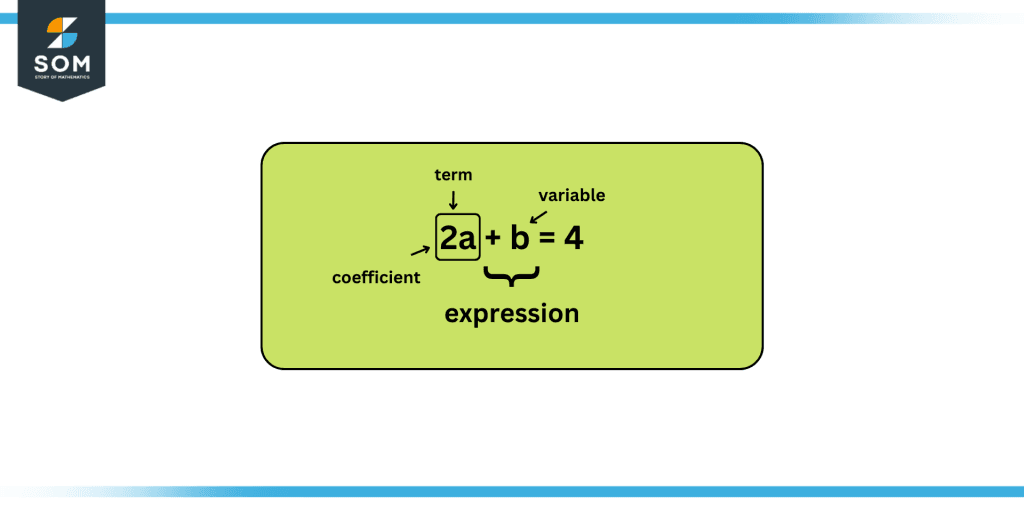
Figure 1: An algebraic term in a mathematical expression.
A term in mathematics is a numerical or algebraic expression that represents a value or a set of values. It is used to describe the components of a mathematical equation or formula.
Numbers, variables, and mathematical operations like addition, subtraction, multiplication, and division can all be incorporated into terms. For instance, the terms “3x” and “2y” both reflect values that can change depending on the precise values of “x” and “y” in the equation “3x + 2y = 6.”
Degree of a Term
The degree of a term is yet another crucial idea in terms. The highest power of a variable within a term is referred to as the term’s degree. For instance, the degree of the term 3x2 is 2, the degree of the term 2x is 1, and the degree of the term 7 is zero. Whereas the degree of the expression 3x2 + 2x + 7 is the degree of the term with the highest power, i.e., 2.
The sort of function that an expression expresses is identified by the degree of a term. For instance, a quadratic function is an expression with terms of degree 2, whereas a cubic function is an expression with terms of degree 3.
Factors of a Term
The following are some of the properties of the factors of a term:
- A term’s factors are the numbers or variables that are multiplied to create it. For instance, the word 3ab has the elements 3, a, and b.
- It is impossible to factorize the elements further. One cannot, for instance, write 3ab as the product of the numbers 3 and ab. This is due to the fact that ab can be reduced to a and b.
- The factors of the term 5x4 are 5, x, x, x, and x.
- 1 is not considered a separate variable.
Types of Terms
Terms can be divided into like terms and unlike terms. We can also categorize expressions on the basis of the number of terms they entail. They are monomials, binomials, and polynomials.
Like Terms
Like terms are those with the same variable or variables raised to the same power (s). By adding or subtracting their coefficients, like terms can be combined or made simpler (numerical values).

Figure 2: Two expressions having like terms.
For instance, the terms 3x2 and 4x2 are like terms in the expression 3x2 + 4x2 because they both have the variable x raised to the same power 2. This expression can be simplified by adding the coefficients of the like terms as:
3x2 + 4x2 = 7x2
The terms “5x” and “2x” are like terms in the expression “5x + 2x” because they both have the variable “x” raised to the same power 1. This expression can be simplified by adding the coefficients of the like terms as:
5x + 2x = 7x
Regardless of the coefficient values, like terms are terms that have the same variables raised to the same powers. Expressions and equations can be made simpler by combining like terms by adding or removing the coefficients from each other.
Unlike Terms
Terms with various variables or variables raised to different powers are considered to be unlike terms. In contrast to like terms, unlike terms cannot be combined by merely adding or subtracting their coefficients.
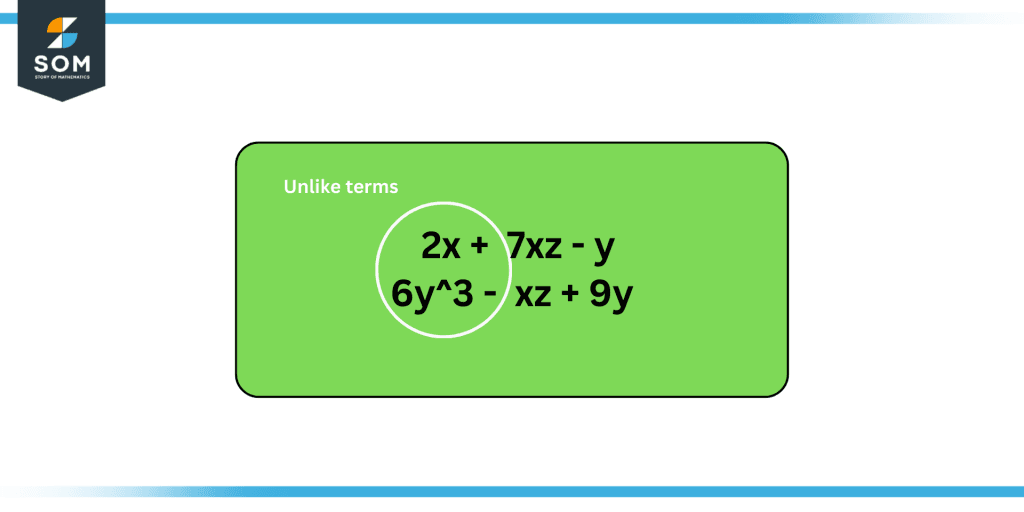
Figure 3: Two expressions with unlike terms.
For instance, the terms “3x” and “4y” in the statement “3x + 4y” are unlike terms since they each have a unique variable. The coefficients of these terms cannot be added or subtracted to simplify them further.
The terms “5x2” and “2x” in the expression “5x2 + 2x” are also unlike terms because they have the same variable “x” raised to different powers (2 and 1, respectively). The coefficients of these terms cannot be added or subtracted to simplify them further.
Unlike terms must remain as separate terms in an expression or equation since they cannot be combined by simply adding or subtracting their coefficients.
Terms in Algebra
In algebra, terms are often used to simplify expressions and to help find solutions to equations. For instance, the expression “3x + 2y + 7z” can be simplified into the form “3x + 2y + 7z = k,” where “k” is a constant value. The expression on the left side of the equation represents a set of terms that are related to each other and can be manipulated to find the solution.
In addition to variables, terms can also include constants, which are values that do not change. For example, in the equation “3x + 2 = 7,” the term “2” is a constant value.
Terms can also be combined or grouped together in various ways. For example, in the equation “3x + 2y + 7z = k,” the terms “3x” and “2y” can be grouped together as “3x + 2y” and the entire expression can be written as “(3x + 2y) + 7z = k.” Grouping terms in this way can help simplify expressions and make them easier to manipulate.
Terms in Calculus
Calculus also uses terms to explain the elements of a function. A function in calculus is a mathematical model that gives each input value a specific output value. Equations can be used to express functions, and the terms in the equation stand in for the parts of the function.
For instance, the terms “3x” and “2” in the equation “y = 3x + 2” stand for the slope and y-intercept, respectively, of the function.
A Quick Summary
The concept of terms is essential in mathematics. They are applied to model functions, simplify expressions, and describe the elements of equations. In order to solve mathematical problems and comprehend the underlying concepts of different mathematical fields, it is critical to understand terms and how they can be combined and used.
Solved Examples Involving Terms
Example 1
Identify how many terms, like terms, and unlike terms are there in the following equations:
9a + 6b
4p2 + 3p + 4q + 8p + 10p2
3xyz
Solution
9a and 6b are the two terms in equation 9a + 6b. However, despite having two unlike terms with the variables a and b, there are no like terms.
The equation 4p2 + 3p + 4q + 8p + 10p2 has 5 terms. Despite having 3 unlike terms, there are only 2 like terms. In contrast, when it is reduced, it becomes:
= (4p2 + 10p2) + (3p+ 8p) + 4q
= 14p2 + 11p + 4q.
Despite having three unlike terms with the variables p2, p, and q, there exist three terms with no like terms.
In equation 3xyz, there is only one term. However, since there are no additional terms to compare it to, there are no like and unlike terms.
Example 2
In the following algebraic expression:
2x – 5y + 8
what are the terms, variables, and constants?
Solution
In the mathematical expression 2x – 5y + 8, the terms are 2x, -5y, and 8.
Both x and y are variables. Numbers with multiple possible numerical values are called variables.
The number 8 is a constant. Numbers with a fixed numerical value are known as constants.
All images/mathematical drawings were created using GeoGebra.
