Multiplicative Identity|Definition & Meaning

Before exploring the concept of multiplicative identity let us first explore the fundamental properties of numbers and what is identity property. Commutative, associative, distributive, and identity are the four fundamental qualities that are deployed with numbers. As opposed to only individual numbers, these qualities apply to groups of numbers. 

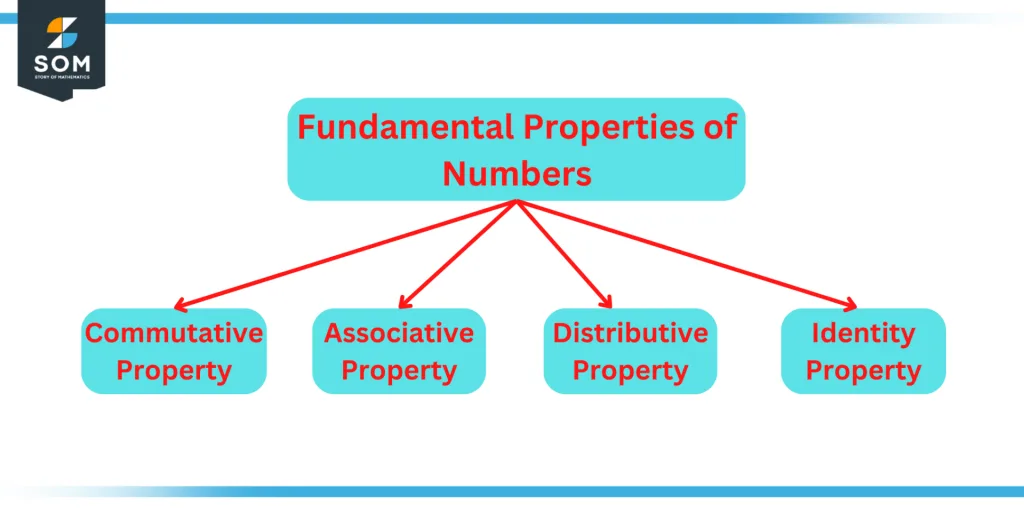
Figure 1: Fundamental Properties of Numbers

Commutative Property

The term “commutative” originates from the term “commute,” which means “to move about.” Therefore, the “commutative feature” alludes to the ability to rearrange numbers within numeric sentences.

For instance, adding 2 and 3 results in the number 5, so if we rearrange the numbers such that 3 and 2 equal 5, we still obtain the correct answer. Likewise, the result of multiplying 6 by 4 is 24, just as the result of multiplying 4 by 6 is 24.

Associative Property

According to the associative property, when you plus or multiply integers, it does not make a difference how you group the numbers.

This principle applies to sequences of integers that are enclosed in brackets. Calculations are enclosed in brackets if we require a person to carry out the computation in the bracket first.

When it comes to a collection of integers that require to be added together, it does not make a difference where the brackets are because the result will always be similar.

In a similar manner, when there is a set of integers that requires to be multiplied, the result will remain the same irrespective of the way the integers are put together.

Distributive Property

The distributive property is a useful tool in mathematics that can be used to simplify difficult issues since it reduces expressions to either the addition or subtraction of two integers.

Under this property, if we multiply the sum of two integers by an integer, we will achieve a similar result as if we multiplied each integer by the number separately and then added the products together.

The distributive property teaches us how to answer statements of the following type x (y + z) by showing us how to multiply the integer directly outside the parenthesis with the numbers that are contained within the brackets and plus them into one.

Now, since the topic we are discussing here is multiplicative identity, let us first discuss what precisely is the identity property.

Identity Property

An integer that you can multiply by, divide into, add to, subtract from, or divide into another number and not have its identity altered is referred to as an identity element. These actions do not alter the initial number. The other number is referred to as an identity element because the identity of the initial number is preserved.

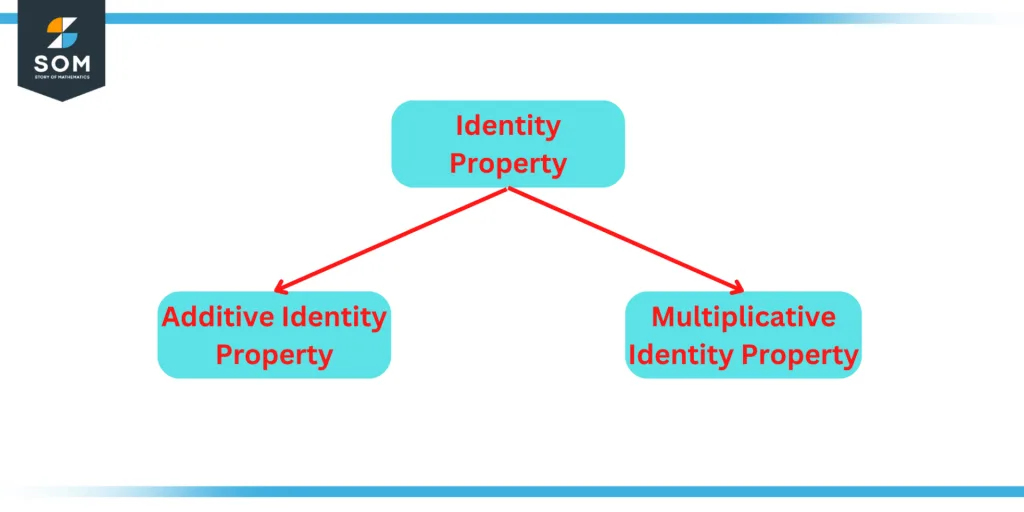
Figure 2: Types of Identity Property

 Identity Element

The identity elements are the following two numbers:

• Zero
• One

Varying operations make use of each of these above-mentioned identity elements. Use for the identity element zero is done in the following operations:

• Add
• Subtract

Use for the identity element one is done in the following operations

• Multiply
• Divide

Multiplicative Identity

A mathematical notion known as “multiplicative identity” describes the character of a number being identical to itself whenever the number is multiplied by 1. To put in other words, multiplying an integer by one always results in the same result: the integer itself.

The term multiplicative identity is also referred to as the identity property of multiplication. Due to its use in numerous algebraic operations as well as its importance in understanding how integers respond when multiplied, this idea is crucial in mathematics.

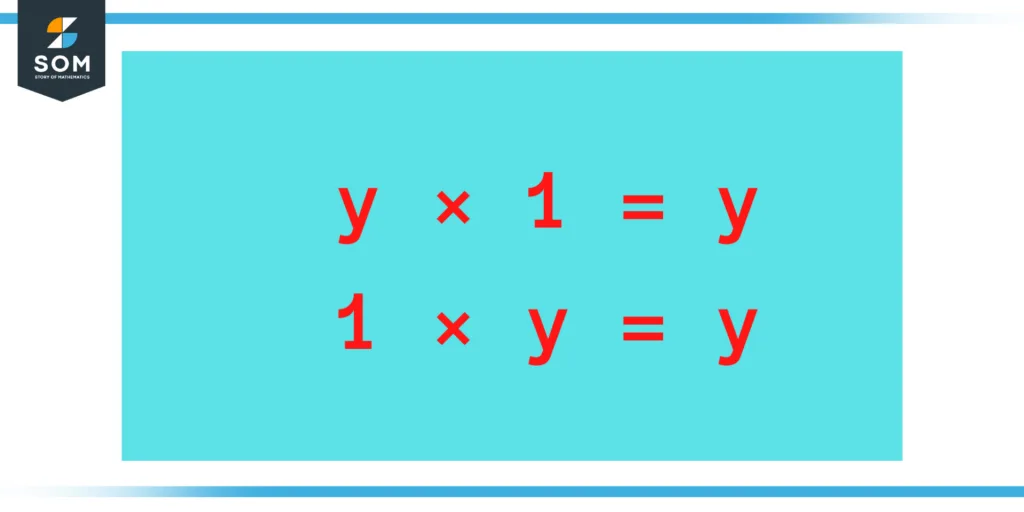
Figure 3: Illustration of Multiplicative Identity Property

Zero as The Multiplicative Identity for All Non-Zero Numbers

The multiplicative identity of zero for all non-zero integers is one of the fundamental characteristics of the multiplication identity property. This indicates that any nonzero integer multiplied by zero would always give zero as an answer.

For instance, the outcome of 5 times 0 is 0. Understanding this characteristic is crucial for us because it shows the way in which integers respond when they are multiplied by zero.

The fact that the number “one” is the multiplicative identity for every integer is another significant aspect of multiplicative identity. By extension, this indicates that multiplying any integer by 1 will always yield a similar result.

For instance, the result of multiplying 5 by 1 is 5. It is crucial to comprehend this feature since it explains how one multiplies a number, which is a fundamental mathematical concept.

Use of The Multiplicative Identity in Solving Equations

There are various techniques to solve equations using the multiplicative identity. Eliminating the constant that is multiplied by the equation’s variable is among the most popular applications of multiplicative identity in equation solving. As an illustration, think about the equation below:

3y = 9

The identity property of multiplication of one-half of the equation can be used to get rid of the constant of three that multiplies y and then solve the equation for y. To accomplish this, divide each side of the equation by three, as it is shown below.

3y / 3 = 9 / 3

That amounts to:

y = 3

In this manner, you can utilise the identity property of multiplication of one-half to find the answer of the variable y in the equation.

Eliminating constants that are dividing the equation’s variable would be another technique to solve equations using the identity property of multiplication. Take the equation below as an illustration:

y / 2 = 4

The identity property of multiplication of two can be used to get rid of the constant of two that is dividing y and then solve the equation for y. To achieve this, multiply each side of the equation by two as shown in the example below:

(y / 2) × 2 = 4 × 2

That amounts to:

y = 8

In this fashion, you may find the answer for the variable of y in the equation using the identity property of multiplication of two.

Thus, we can say that the multiplicative identity is a helpful technique for resolving equations since it gets rid of constants that are multiplying or dividing a particular equation’s variables. Equations can be made simpler, and the answer of a variable can be determined by utilizing the proper multiplicative identity.

In general, the identity property of multiplication is considered to be a core concept in the field of mathematics, with numerous applications in a variety of methods and areas. That perhaps is the reason why success in several mathematical disciplines depends on one’s ability to comprehend and use the multiplicative identity property.

Example

Which of the following mathematical Expressions can be considered an Illustration of the multiplicative identity

1) 3 × 3 = 9

2) 3 × 1 = 3

3) 3 ÷ 3 =1

Solution

Amongst the given expressions, “3 × 1 = 3” is an example of the multiplicative identity.

All images/mathematical drawings were created with GeoGebra.

Multiplication Tables Definition < Glossary Index > Multiplier Definition