What Is 5 1/3 as a Decimal + Solution With Free Steps

The fraction 5 1/3 as a decimal is equal to 5.333.

In Mathematics, a Fraction is defined as a numerator divided by a denominator and it is equal to a Quotient.  Whereas Numerator and Denominator both are integers. Fractions are of different types such as proper fraction, improper fraction, and complex fraction.

A complex Fraction is the one in which a fraction appears in its numerator or denominator. It can occur in both numerator and denominator as well.

If a numerator is greater than a  denominator it is called a Proper Fraction. And if a denominator is greater than a numerator it’s called an Improper Fraction. And there is one more type called Mixed number fraction which is a whole number quotient with a proper fraction remainder.

A decimal form of a fraction can be found simply by dividing a numerator with a denominator. One or more digits may repeat indefinitely or the result may come to an end at some point. A decimal number with a digit that repeats, again and again, is called a Recurring decimal.

We have a fraction of 5 1/3 and we are going to solve it by using the Long division method.

Solution

The given complex fraction is first converted to a simple fraction by multiplying its denominator with a whole number and then adding its numerator.

                                                    5 + 1/3 = 16/3

This is our case is 16/3. Here we have dividend and divisor.

Dividend = 16

Divisor = 3

When we divide this fraction a Quotient is obtained.

Quotient = Dividend $\div$ Divisor = 16 $\div$ 3

We are left with some integers while performing a division called the Remainder.

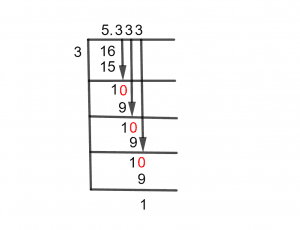
Figure 1

5 1/3 Long Division Method

The fraction we have:

16 $\div$ 3

As the divisor in the given fraction is smaller than the dividend so we don’t need to multiply the dividend by 10 to add a decimal point but it needs to be done if the divisor is greater than the dividend. The fraction 16/3 is divided as illustrated in the instance shown below:

16 $\div$ 3 $\approx$ 5

3 x 5 = 15

16 – 15 = 1

Here,  1  is the Remainder left after division.

Now 1 is dividend and 3 is divisor as the divisor is greater than the dividend therefore multiply the dividend by 10. The necessary steps are shown below:

10 $\div$ 3 $\approx$ 3

3 x 3 = 9

10 – 9 = 1

Our division is still incomplete. To further simplify add a zero with the remainder so that the dividend becomes 10 which is greater than 3 and can undergo division. The detailed division is shown below: 

10 $\div$ 3 $\approx$ 3

3 x 3 = 9

Again the remainder is 10 – 9 = 1

After doing the third iteration the same result as above is obtained which shows that it is a recurring decimal. Solve up to at least the third decimal place.

10 $\div$ 3 $\approx$ 3

3 x 3 = 9

10 – 9 = 1

Remainder = 1,

After three iterations, we stop the division with a conclusion that the remainder is  1 and the quotient is 5.333

Images/mathematical drawings are created with GeoGebra

Fractions as Decimals List