Foil Method to Distribute Two Binomials – Explanation & Examples

What is the Foil Method?

Many students will start thinking of a kitchen when they first hear a mention of the term foil.

Here, we are talking about the FOIL – a mathematical series of steps used to multiply two binomials. Before we learn what the term foil entails, let’s take a quick review of what the word binomial is.

A binomial is simply an expression that consists of two variables or terms separated by either the addition sign (+) or subtraction sign (-). Examples of binomial expressions are 2x + 4, 5x + 3, 4y – 6, – 7y – y etc.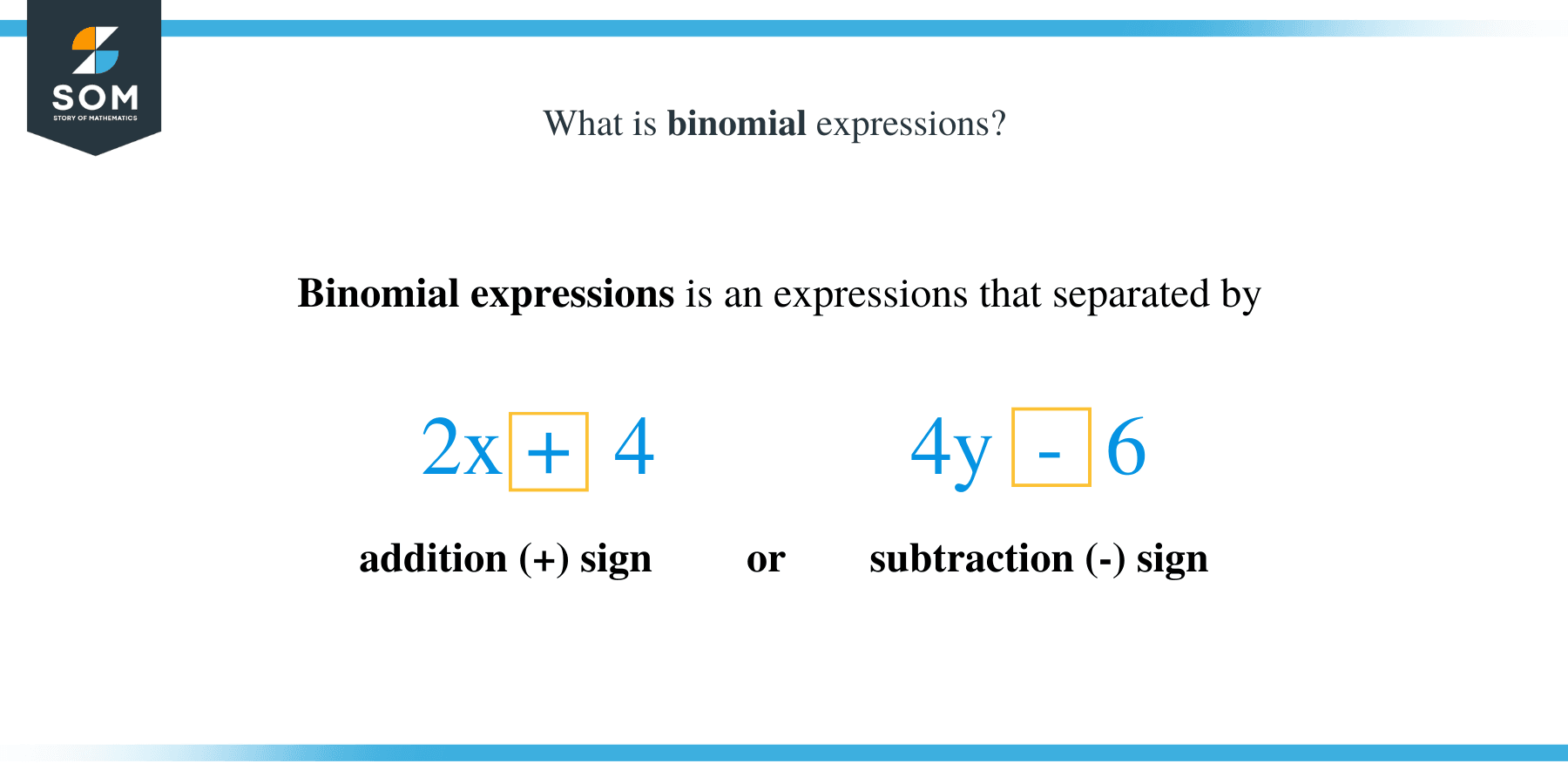

How to do Foil Method?

The foil method is a technique used for remembering the steps required to multiply two binomials in an organized manner.

The F-O-I- L acronym stands for first, outer, inner, and last.

• First, which means multiplying the first terms together, i.e. (a + b) (c + d)
• Outer means that we multiply the outermost terms when the binomials are placed side by side, i.e. (a + b) (c + d).
• Inner means multiply the innermost terms together i.e. i.e. (a + b) (c + d).
• Last. This implies that we multiply together the last term in each binomial, i.e., i.e. (a + b) (c + d).

How do you distribute binomials using the foil method?

Let us put this method into perspective by multiplying two binomials, (a + b) and (c + d).

To find multiply (a + b) * (c + d).

• Multiply the terms which appear in the first position of binomial. In this, case a and c are the terms, and their product are;

(a *c) = ac

• Outer(O) is the next word after the word first(F). Therefore, multiply the outermost or the last terms when the two binomials are written side by side. The outermost terms are b and d.

(b * d) = bd

• The term inner implies that we multiply two terms that are in the middle when the binomials are written side-by-side;

(b * c) = bc

• The last implies that we find the product of the last terms in each binomial. The last terms are b and d. Therefore, b * d = bd.

Now we can sum up the partial products of the two binomials beginning from the first, outer, inner, and then the last. Therefore, (a + b) * (c + d) = ac + ad + bc + bd.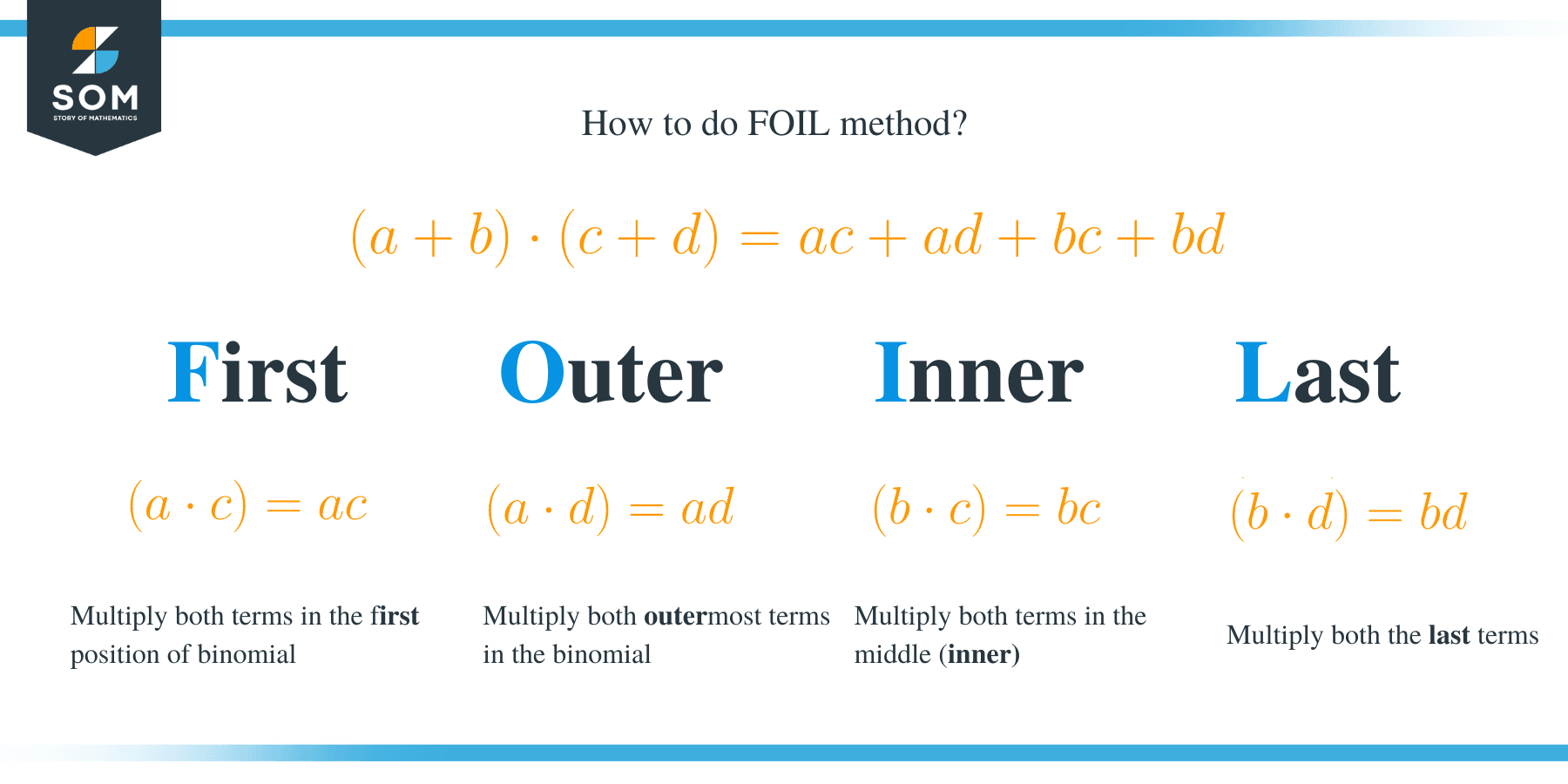

The foil method is an effective technique because we can use it to manipulate numbers, regardless of how they might look ugly with fractions and negative signs.

How do you multiply binomials using the foil method?

To master the foil method better, we shall solve a few examples of binomials.

Example 1

Multiply (2x + 3) (3x – 1)

Solution

• Begin, by multiplying together, the first terms of each binomial

= 2x * 3x = 6x ²

• Now multiply the outer terms.

= 2x * -1= -2x

• Now multiply the inner terms.

= (3) * (3x) = 9x

• Finally, multiply the last team in each binomial together.

= (3) * (–1) = –3

• Sum up the partial products starting from the first to last product and collect the like terms;

= 6x ² + (-2x) + 9x + (-3)

= 6x ² + 7x – 3.

Example 2

Use the foil method to solve:(-7x−3) (−2x+8)

Solution

• Multiply the first term:

= -7x * -2x = 14x ²

• Multiply the outer terms:

= -7x * 8 = -56x

• Multiply the inner terms of the binomial:

= – 3 * -2x = 6x

• Finally, multiply the last terms:

= – 3 * 8 = -24

• Find the sum of the partial products and collect the like terms:

= 14x ² + ( -56x) + 6x + (-24)

= 14x ² – 56x – 24

Example 3

Multiply (x – 3) (2x – 9)

Solution

• Multiply the first terms together:

= (x) * (2x) = 2x ²

• Multiply the outermost terms of each binomial:

= (x) *(–9) = –9x

• Multiply the inner terms of the binomial:

= (–3) * (2x) = –6x

• Multiply the last terms of each binomial:

= (–3) * (–9) = 27

• Sum up the products following the foil order and collect the like terms:

= 2x ² – 9x -6x + 27

= 2x ² – 15x +27

Example 4

Multiply [x + (y – 4)] [3x + (2y + 1)]

Solution

• In this case, the operations are broken down into smaller units, and the results combine:
• Begin by multiplying the first terms:

= (x) * 3x = 3x ²

• Multiply the outer terms of each binomial:

= (x) * (2y + 1) = 2xy + x

• Multiply the inner terms of each binomial:

= (y – 4) (3x) = 3xy – 12x

• Now finish by multiplying the last terms:

= (y – 4) (2y + 1)

Since the last terms area gain two binomials; Sum up the products:

= 3x ² + 2xy + x + 3xy – 12x +(y – 4) (2y + 1)

= 3x ² + 5xy – 11x + (y – 4) (2y + 1)

Again, apply the foil method on (y – 4) (2y + 1).

• (y) * (2y) = 2y²
• (y) *(1) = y
• (–4) * (2y) = –8y
• (–4) * (1) = –4

Sum up the totals and collect the like terms:

= 2y² – 7y – 4

Now replace this answer into the two binomials:

= 3x ² + 5xy – 11x + (y – 4) (2y + 1) = 3x ² + 5xy – 11x + 2y² – 7y – 4

Therefore,

[x + (y – 4)] [3x + (2y + 1)] = 3x ² + 5xy – 11x + 2y² – 7y – 4