
This question aims to find the directional derivative of the function f at the given point in the direction indicated by the angle $\theta$.
Time
A directional Derivative is a type of derivative that tells us the change of the function at a point with time in the vector direction.
Vector direction
We also find partial derivatives according to the directional derivative formula. The partial derivatives can be found by keeping one of the variables constant while applying derivation of the other.
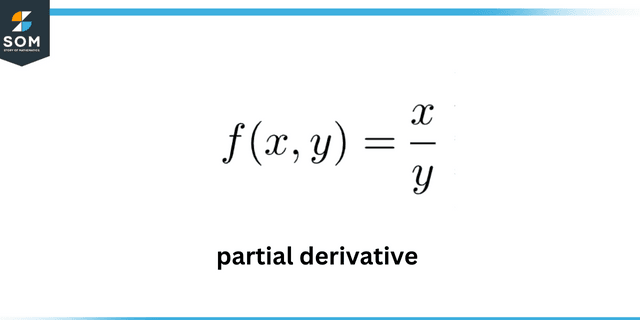
Partial derivative
Expert Answer
The given function is:
\[f(x, y) = e^x cos y\]
\[(x, y) = ( 0, 0 )\]
The angle is given by:
\[\theta = \frac{\pi}{4}\]
The formula to find the directional derivative of the given function is:
\[D_u f(x, y) = f_x (x, y) a + f_y (x, y) b\]
To find the partial derivatives:
$f_x = e ^ x cos y$ and $f_y = – e ^ x sin y$
Here, a and b represent the angle. In this case, the angle is $\theta$.
By putting values in the above-mentioned formula of directional derivative:
\[D_u f(x, y ) = ( e ^ x cos y ) cos ( \frac { \pi } { 4 } ) + ( – e ^ x sin y ) sin ( \frac { \pi } { 4 } ) \]
\[D_u f(x, y) = ( e ^ x cos y ) ( \frac { 1 } { \sqrt { 2 } } ) + ( – e ^ x sin y ) ( \frac { 1 } { \sqrt { 2 } } ) \]
\[ D _ u f ( x, y ) = \frac { \sqrt { 2 }} { 2 } [ ( e ^ x cos y ) + ( – e ^ x sin y ) \]
By putting values of x and y:
\[ D _ u f ( x, y ) = \frac { \sqrt { 2 }} { 2 } [ ( e ^ 0 cos 0 ) + ( – e ^ 0 sin 0 ) \]
\[ D _ u f ( 0, 0 ) = \frac { \sqrt { 2 }} { 2 } \]
Numerical Solution
The directional derivative of the function f at the given point in the direction indicated by the angle $\theta$ is $ \frac {\sqrt {2}} {2} $.
Example
Find the directional derivative at $ \theta = \frac{\pi}{3} $
\[D_u f(x, y) = (e^x cos y) cos(\frac{\pi}{3}) + (-e^x sin y) sin(\frac{\pi}{3})\]
\[= (e ^ x cos y ) (\frac{1}{2}) + (-e^x sin y)(\frac {\sqrt{3}}{2})\]
\[= \frac { \sqrt { 3 } +1}{2} [(e^x cos y) + (- e^x sin y ) \]
\[= \frac { \sqrt {3} + 1}{2} [(e^0 cos 0 ) + ( – e ^ 0 sin 0 )\]
\[D _ u f ( 0, 0 ) = \frac { \sqrt {3} + 1} { 2 } \]
Image/Mathematical drawings are created in Geogebra
