Take note of the following points:
$P(1,0,1) , Q(-2,1,4) , R(7,2,7)$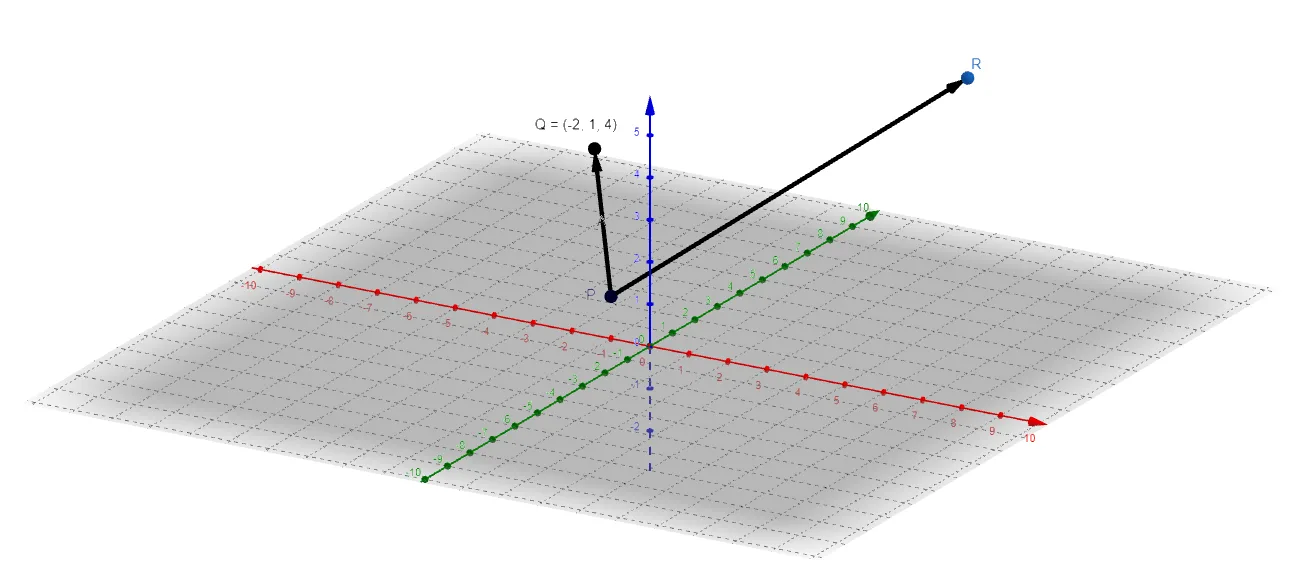 Let $\overrightarrow{C}$ be a non-zero vector orthogonal to the plane through the points $P,Q$ and $R$, then$\overrightarrow{C}=\overrightarrow{A}\times\overrightarrow{B}$$=\begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\-3&1&3\\6&2&6\end{vmatrix}$$=(6-6)\hat{i}-(-18-18)\hat{j}+(-6-6)\hat{k}$$=0\hat{i}+36\hat{j}-12\hat{k}$$=<0,36,-12>$Since it is known that $\overrightarrow{A}$ and $\overrightarrow{B}$ are two sides of a triangle, we also know that the magnitude of the cross-product can be used to calculate the area of the triangle, thereforeArea of the triangle $=\dfrac{1}{2}|\overrightarrow{A}\times \overrightarrow{B}|$$=\dfrac{1}{2}\sqrt{0^2+36^2+(-12)^2}$$=\sqrt{1296+144}=\dfrac{1}{2}(12\sqrt{10})$$=6\sqrt{10}$
Let $\overrightarrow{C}$ be a non-zero vector orthogonal to the plane through the points $P,Q$ and $R$, then$\overrightarrow{C}=\overrightarrow{A}\times\overrightarrow{B}$$=\begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\-3&1&3\\6&2&6\end{vmatrix}$$=(6-6)\hat{i}-(-18-18)\hat{j}+(-6-6)\hat{k}$$=0\hat{i}+36\hat{j}-12\hat{k}$$=<0,36,-12>$Since it is known that $\overrightarrow{A}$ and $\overrightarrow{B}$ are two sides of a triangle, we also know that the magnitude of the cross-product can be used to calculate the area of the triangle, thereforeArea of the triangle $=\dfrac{1}{2}|\overrightarrow{A}\times \overrightarrow{B}|$$=\dfrac{1}{2}\sqrt{0^2+36^2+(-12)^2}$$=\sqrt{1296+144}=\dfrac{1}{2}(12\sqrt{10})$$=6\sqrt{10}$
- Find a nonzero vector orthogonal to the plane through the points $P, Q$, and $R$.
- Find the area of the triangle $PQR$.
Expert Answer
Assume that $\overrightarrow{A}$ and $\overrightarrow{B}$ are two linearly independent vectors. We know that the cross-product of two linearly independent vectors yields a non-zero vector that is orthogonal to both.Let$\overrightarrow{A}=\overrightarrow{PQ}$$\overrightarrow{A}=(-2,1,4)-(1,0,1)$$\overrightarrow{A}=(-3,1,3)$And$\overrightarrow{B}=\overrightarrow{PR}$$\overrightarrow{B}=(7,2,7)-(1,0,1)$$\overrightarrow{B}=(6,2,6)$ Let $\overrightarrow{C}$ be a non-zero vector orthogonal to the plane through the points $P,Q$ and $R$, then$\overrightarrow{C}=\overrightarrow{A}\times\overrightarrow{B}$$=\begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\-3&1&3\\6&2&6\end{vmatrix}$$=(6-6)\hat{i}-(-18-18)\hat{j}+(-6-6)\hat{k}$$=0\hat{i}+36\hat{j}-12\hat{k}$$=<0,36,-12>$Since it is known that $\overrightarrow{A}$ and $\overrightarrow{B}$ are two sides of a triangle, we also know that the magnitude of the cross-product can be used to calculate the area of the triangle, thereforeArea of the triangle $=\dfrac{1}{2}|\overrightarrow{A}\times \overrightarrow{B}|$$=\dfrac{1}{2}\sqrt{0^2+36^2+(-12)^2}$$=\sqrt{1296+144}=\dfrac{1}{2}(12\sqrt{10})$$=6\sqrt{10}$
Let $\overrightarrow{C}$ be a non-zero vector orthogonal to the plane through the points $P,Q$ and $R$, then$\overrightarrow{C}=\overrightarrow{A}\times\overrightarrow{B}$$=\begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\-3&1&3\\6&2&6\end{vmatrix}$$=(6-6)\hat{i}-(-18-18)\hat{j}+(-6-6)\hat{k}$$=0\hat{i}+36\hat{j}-12\hat{k}$$=<0,36,-12>$Since it is known that $\overrightarrow{A}$ and $\overrightarrow{B}$ are two sides of a triangle, we also know that the magnitude of the cross-product can be used to calculate the area of the triangle, thereforeArea of the triangle $=\dfrac{1}{2}|\overrightarrow{A}\times \overrightarrow{B}|$$=\dfrac{1}{2}\sqrt{0^2+36^2+(-12)^2}$$=\sqrt{1296+144}=\dfrac{1}{2}(12\sqrt{10})$$=6\sqrt{10}$