Dividing Mixed Numbers – Methods & Examples

How to Divide Mixed Numbers?

Mixed numbers consist of an integer followed by a fraction. It is initially an improper fraction, which then broken down into a mixed number form. Division of mixed numbers is very similar to the multiplication of the mixed numbers.

Here are the steps followed when dividing mixed numbers:

• Begin by converting each mixed fraction to an improper.
• Invert or flip upside down the improper fraction that is the divisor
• Multiply the first fraction by the second fraction. Multiplication of numerators and denominators are done separately.
• Convert the resultant fraction into a mixed number if it is improper.
• Simplify the mixed number to its lowest terms possible.

Example 1

Solve the following

1 ³/₄ ÷ 2 ²/₅

Solution

• Convert each mixed number into improper fraction.

1 ³/₄ = 7/4 and 2 ²/₅ = 12/5

• Now proceed with the division as:

1 ³/₄ ÷ 2 ²/₅ = 7/4 ÷ 12/5

• Determine the reciprocal of the second fraction as 5/12

7/4 ÷ 12/5 = 7/4 x 5/12

• Multiply the numerators together and denominators also together.

7/4 x 5/12= (5 x 7)/(12 x 4)

= 35/48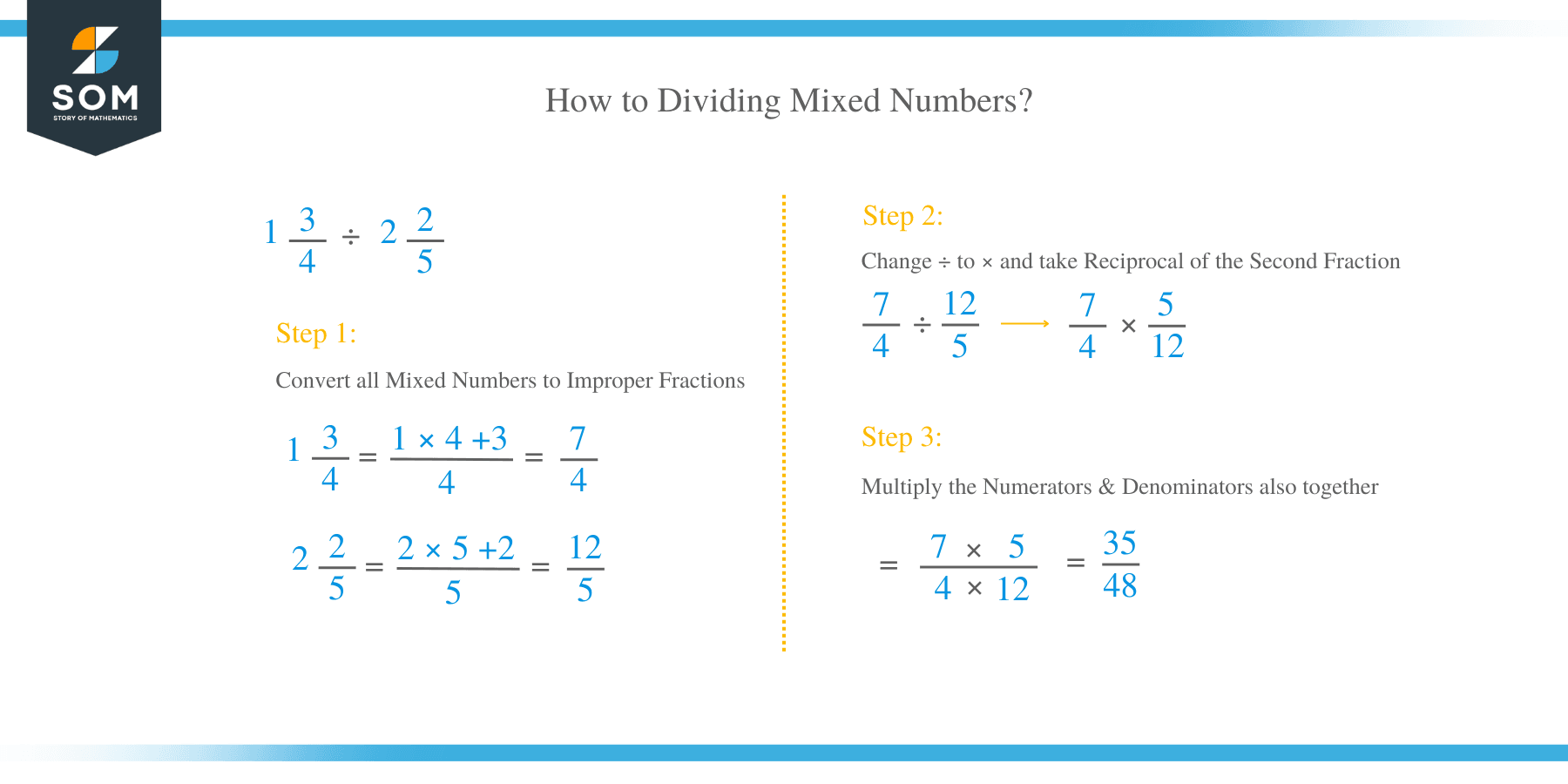

Example 2

Workout:

2 ¾ ÷ 1 ²/₃

Solution

2 ¾ ÷ 1 2/3

= 11/4 ÷ 5/3

= 11/4 × 3/5

= (11 × 3)/(4 × 5)

= 33/20

= 1 ¹³/₂₀

Example 3

Simplify the following,

2 ⁴/₁₇ ÷ 1 ⁴/₁₇

Solution

2 ⁴/₁₇ ÷ 1 ⁴/₁₇

= 38/17 ÷ 21/17

= 38/17 × 17/21

= (38 × 17)/(17 × 21)

= 646/357

= 38/21

= 1 ¹⁷/₂₁

Example 4

Work out: 3 ¹/₃ ÷ 1 ⁵/₆

Solution

Step 1:

Convert each mixed number to an improper fraction.

3 ¹/₃ = 10/3 and 1 ⁵/₆ = 11/6

Now, 3 ¹/₃ ÷ 1 ⁵/₆ = 10/3 ÷ 11/6

Step 2:

Invert the second fraction and change the operator to multiplication.

10/3 ÷ 11/6 = 10/3 x 6/11

Step 3:

Multiply the numerators at the top and denominators at the bottom.

10/3 x 6/11 = (10 x 6)/(11 x 3)

= 60/33

Step 4:

Simplify the answer.

Both the numerator and denominator have a common factor 3, and therefore simplify the fraction to its lowest terms.

60/33 = 20/11

Now convert the answer back to a mixed number.

20/11= 1 ⁹/₁₁

Therefore, 3 ¹/₃ ÷ 1 ⁵/₆ = 1 ⁹/₁₁

Example 5

Work out: 4 ÷ 2 ¹/₃

Solution

Step 1:

Convert the mixed numbers into improper fractions.

2 ¹/₃ = 7/3

4 ÷ 2 ¹/₃ = 4/1÷ 7/3

Step 2:

Find the reciprocal of the second fraction and change the operator to multiplication.

4/1÷ 7/3 = 4/1 x 3/7

Step 3:

Multiply the fractions

4 × 3/7 = 12/7

Step 4:

Simplify and convert.

Now convert the fraction back to a mixed number.

12/7 = 1 ⁵/₇

Example 6

Two number have a product of 18. If one number is 8 ²/₅, Calculate the value of the other number.

Solution

The product of the numbers = 18

One of the numbers = 8 ²/₅ = {(8 × 5) + 2}/5 = 42/5

To find the value of the other number, divide 18 by the fraction.

= 18 ÷ 42/5 = 18 × 5/42

= 90/42

= 15/7

Therefore, the other number is:

= 2 ¹/₇

Example 7

A 25 m long pole is cut into logs of each 1 ²/₃ meters. Calculate the total number of logs cut from the pole.

Solution

Total number of logs cut can be calculated by dividing 25 m by 1 ²/₃ = 25 ÷ 1 ²/₃

= 25 ÷ 5/3

= 25 × 3/5

= 75/5

Therefore, the number of logs cut = 15