Dividing Fractions – Methods & Examples

A fraction is normally written in two parts, where the numerator is displayed above a line or before a slash whereas, the denominator is displayed below or before the line.

How to Divide Fractions?

In this article we are going to learn how division of fractions is carried out. There are two methods of dividing fractions. Let’s see them one by one below.

Multiplication by the Reciprocal

In this method the second fraction is inverted in such a way that numerator becomes the denominator and denominator becomes the numerator of the fraction.

Multiply the first fraction by the inverted fraction and simplify the result if possible. For instance,

1/2 ÷ 1/6

• Turn the second fraction upside down or find its reciprocal:

1/6 = 6/1

• Multiply the first fraction by that reciprocal of the second fraction:

1/2 × 6/1 = 6/2

• Simplify the fraction t to its lowest terms:

6/2 = 3

Example 1

3/8 ÷ 5/11
Rewrite the equation and simplify,

3/8 x 11/5 = 33/40

Example 2

2/9 ÷ 7/10

Rewrite the equation and simplify,

2/9 x 10/7 = 20/63

Example 3

6 ÷ 2/7

Rewrite the fraction,

6/1 x 7/2 = 42/2

Simplify the fraction

42/2 = 21

Example 4

9/4 ÷ 5

Rewrite the fraction and simplify,

9/4 x 1/5 = 9/20

Example 5

3/4 ÷ 2/5

Rewrite the fraction by changing the division sign to multiplication.

3/4 ÷ 2/5 = 3/4 x 5/2 = 15/8

Example 6
2/9 ÷ 4/15

Rewrite the fraction and simplify,

2/9 ÷ 4/15 = 2/9 x 15/4 = 30/36

Simplify the fraction

30/36 = 5/6

Dividing Fractions with Different Denominators

This method does work, but it requires you to change the fractions into common denominators before starting to solve.

Yet the first method of dividing fractions does not require common denominators, you only need to invert or flip the second fraction and change the problem to multiplication.
Get common denominators and then divide the numerators.

Example 7

2/3 ÷ 1/2
Rewrite the with common denominators. In this case 6 is the common denominator.
2/3 = 4/6
1/2 = 3/6
Divide the numerators to get the final results

4/6 ÷ 3/6 = 4 ÷ 3= 4/3

Example 8

3/8 ÷ 2/10

Rewrite the fractions with the least common multiple as their denominator.

The L.C.M of 8 and 10 is 40

3/8 = 15/40

2/10 = 8/40
Divide the numerators of the fractions

15/40 ÷ 8/40 = 15 ÷ 8 = 1⁷/₈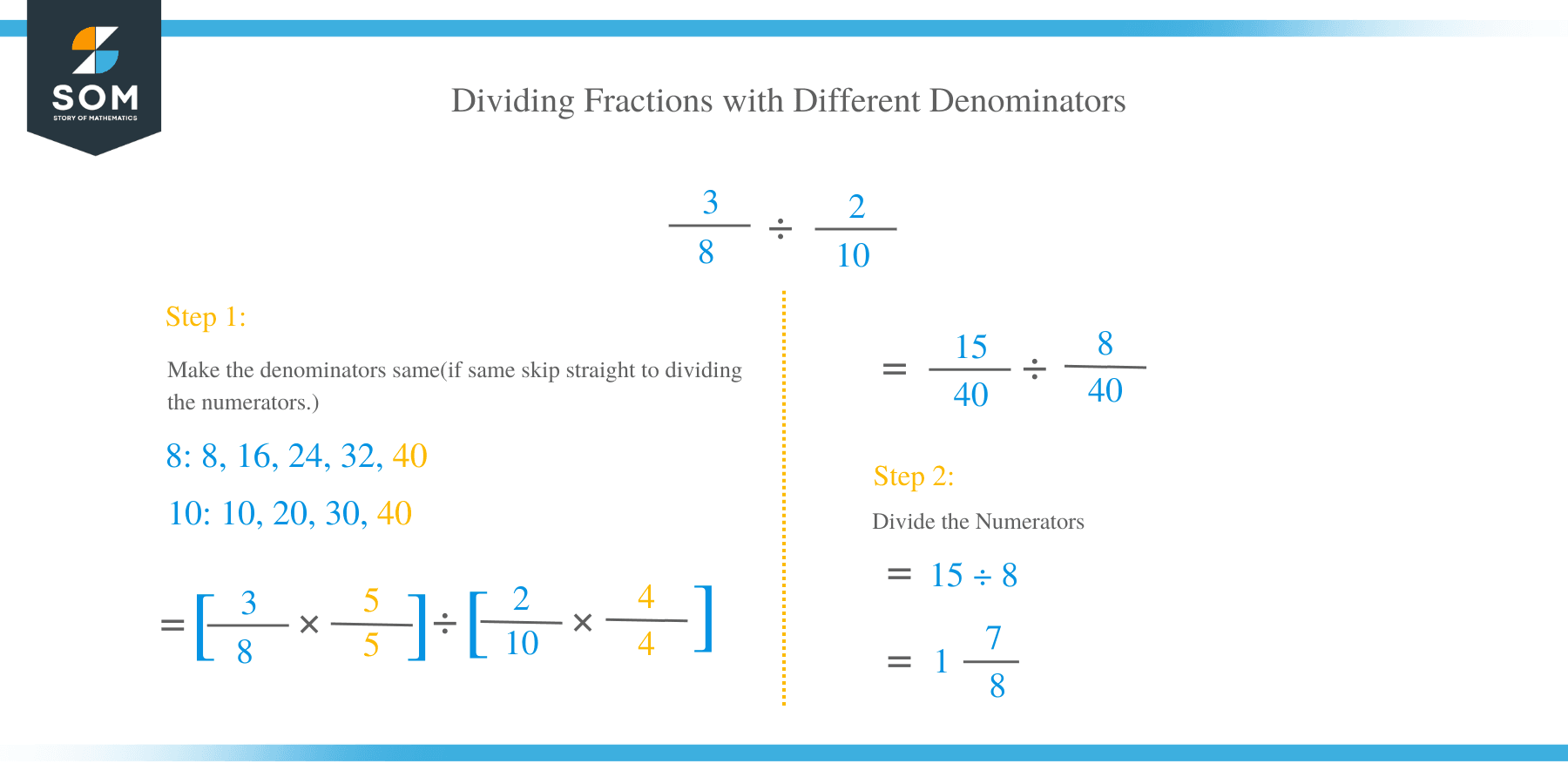

Practice Questions with Solutions

1. Divide 3/5 by 12

Solution

3/5 ÷ 12

Determine the reciprocal of the whole number and multiply with the fractional number.

= 3/5 ÷ 12/1

= 3/5 × 1/12

= (3 × 1)/(5 × 12)

Express the results in its lowest terms.

= 3/60

= 1/20

2. Workout: 5/7 ÷ 10

Solution

Find the inverse of the whole number and multiply with the fraction.

= 5/7 ÷ 10/1

= 5/7 × 1/10

= (5 × 1)/(7 × 10)

= 5/70

Reduce the product in its lowest terms.

= 1/14

3. Divide the following two fractions: 7/8 by 1/5

Solution

7/8 ÷ 1/5

Determine the reciprocal of 1/5 ad multiply it by the first fraction

= 7/8 × 5/1

= (7 × 5)/(8 × 1)

= 35/8

Simplify or convert the product into a mixed fraction

= 4 ³/₈

4. Divide: 5/9 ÷ 10/18

Solution

= 5/9 × 18/10

= (5 × 18)/(9 × 10)

= 90/90

= 1

5. Solve: 2 ¾ ÷ 1 ²/₃

Solution

= 11/4 ÷ 5/3

= 11/4 × 3/5

= (11 × 3)/(4 × 5)

= 33/20

= 1 ¹³/₂₀

6. Divide: 2 ⁴/₁₇ ÷ 1 ⁴/₁₇

Solution

= 38/17 ÷ 21/17

= 38/17 × 17/21

= (38 × 17)/(17 × 21)

= 646/357

= 38/21

= 1 ¹⁷/₂₁

7. Work out: 2/3 ÷ 1/3

Solution

= 2/3 / 1/3

= 2/3 × 3/1

= 2/3 × 3

= 6/3

= 2

8. Divide: 1/3 ÷ 2/5

Solution

Multiply the first fraction by the reciprocal of the second fraction
= 1/3 × 5/2
= (1 × 5)/(3 × 2)
= 5/6

9. Divide the fraction: 2 ¹/₇ ÷ 7/2

Solution

= (2 × 7 + 1)/7 ÷ 7/2
= 15/7 ÷ 7/2
= 15/7 × 2/7
= (15 × 2)/(7 × 7)
= 30/49

10. Workout: 6 ²/₃ ÷ 4 ¹/₅

Solution

= (6 × 3 + 2)/3 ÷ (4 × 5 + 1)/5
= 20/3 ÷ 21/5
= 20/3 × 5/21
= (20 × 5)/(3 × 21)
= 100/63

11. Solve: 5 ¹/₈ ÷ 8 ²/₁₆

Solution

= (5 × 8 + 1)/8 ÷ (8 × 16 + 2)/16
= 41/8 ÷ 130/16
= 41/8 × 16/130

= 41/65