• Home
  • >
  • Directly Proportional – Explanation & Examples

Directly Proportional – Explanation & Examples

Directly ProportionalWhat does Directly Proportional Mean?

Direct proportion is the relationship between two variables whose ratio is equal to a constant value. In other words, direct proportion is a situation where an increase in one quantity causes a corresponding increase in the other quantity, or a decrease in one quantity results in a decrease in the other quantity. 

Sometimes, the word proportional is used without the word direct, just know that they have a similar meaning.

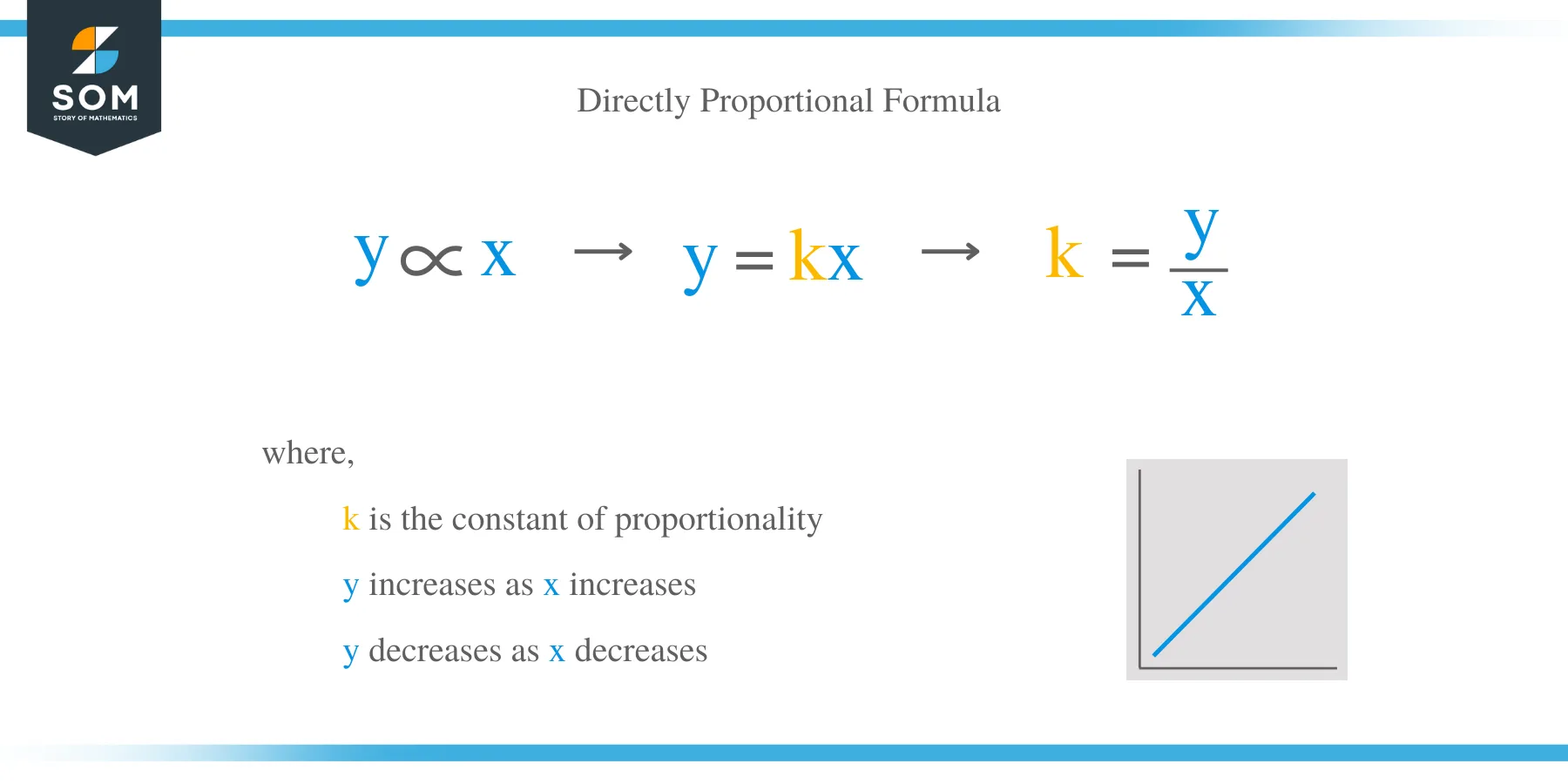
Directly Proportional Formula

Direct proportion is denoted by the proportional symbol (∝). For example, if two variables x and y are directly proportional to each other, then this statement can be represented as x ∝ y.

When we replace the proportionality sign (∝) with an equal sign (=), the equation changes to:

x = k * y or x/y = k, where k is called non-zero constant of proportionality.

In our day-to-day life, we often encounter situations where a variation in one quantity results in a variation in another quantity. Let’s take a look at some of the real-life examples of directly proportional concept.

  • The cost of the food items is directly proportional to the weight.
  • Work done is directly proportional to the number of workers. This means that, more workers, more work and les workers, less work accomplished.
  • The fuel consumption of a car is proportional to the distance covered.Directly Proportional Formula

 

Example 1

The fuel consumption of a car is 15 liters of diesel per 100 km. What distance can the car cover with 5 liters of diesel?

Solution

  • Fuel consumed for every 100 km covered = 15 liters
  • Therefore, the car will cover (100/15) km using 1 liter of the fuel

If 1 liter => (100/15) km

  • What about 5 liters of diesel

= {(100/15) × 5} km

= 33.3
Therefore, the car can cover 33.3 km using 5 liters of the fuel.

 

Example 2

The cost of 9 kg of beans is $ 166.50. How many kgs of beans can be bought for $ 259?

Solution

  • $ 166.50 = > 9 kg of beans
  • What about $ 1 => 9/166.50 kg
    Therefore the amount of beans purchased for $259 = {(9/166.50) × 259} kg
  • =14 kg
    Hence, 14 kg of beans can be bought for $259

 

Example 3

The total wages for 15 men working for 6 days are $ 9450. What is the total wages for 19 men working for 5 days?

Solution

Wages of 15 men in 6 days => $ 9450
The wage in 6 days for 1 worker = >$ (9450/15)
The wage in 1 day for 1 worker => $ (9450/15 × 1/6)
Wages of 19 men in a day => $ (9450 × 1/6 × 19)

The total wages of 19 men in 5 days = $ (9450 × 1/6 × 19 × 5)
= $ 9975
Therefore, 19 men earn a total of $ 9975 in 5 days.

Practice Questions

1. If the total daily wages of $7$ women or $5$ men is $\$525$. What will be the total daily wage of $13$ women and $7$ men?

2. Jackie and her sister are going on a road trip. She noted that her car consumes $6.8$ L for every $102$ km. How far can Jackie’s car if its tank contains $30$ L?

3. In Ryan’s construction company, it costs him $\$7, 200$ to pay for the wages of $12$ workers who are working for $6$ days. How much does it cost Ryan’s company to pay for the wages of $18$ staff working for $5$ days?

4. Alice is known for her handcrafted soaps. It costs her $\$540$ to create $12$ cured bars of soaps each weighing $2.5$ kilograms. How much will it cost her to create $24$ bars of soaps but this time, weighing $3$ kilograms?

5. Felix is studying a city map that is represented with a scale of $1:30000$ (with units of cm : m). He noticed that two blocks are $8$ cm apart on the map, what is the actual distance between the two blocks?

6. A $12$-meter flag post casts a shadow of $8$ meters. What is the height of a flag post that casts a shadow of $18$ m?

7. A train takes $8$ hours to cover $600$ kilometers. How long will it take to cover $1500$ kilometers?

8. In a zero-waste store, it costs $\$120$ to refill $12$ jars of body scrub each weighing $800$ g. How much would it cost to refill $36$ jars of body scrub each weighing one kilogram?


 

Previous Lesson | Main Page | Next Lesson