Degrees and Radians – Explanation & Examples

Like every other quantity, angles also have units for measurement. Radians and Degrees are two basic units for measuring the angles. There are other units to measure the angles (like gradians and MRADs), but in high school, you will only see these two units.

What are Degrees and Radians?

The most popular unit for measuring angles that most people are familiar with is the degree is written (°). The subunits of a degree are minutes and seconds. There are 360 degrees, 180 degrees for a half-circle (semi-circle), and 90 degrees for a quarter circle (a right triangle) in a full circle or one complete rotation.

Degrees basically state direction and angle size. Facing North means you are facing the direction of 0 degrees. If you turn towards South, you are facing the direction of 90 degrees. If you come back to North after full rotation, you have turned through 360 degrees. Usually, the anticlockwise direction is considered positive. If you turn towards West from North, the angle will be either -90 degrees or +270 degrees.

In geometry, there is another unit for measuring angles, known as the radian (Rad).

Now, why do we need radians when we are already comfortable with angles?

Most computation in mathematics involves numbers. Since degrees are not actually numbers, then the radians measure is preferred and often required to solve problems.

A good example that’s similar to this concept is using decimals when we have percentages. Although a percentage can be shown with a number followed by a % sign, we convert it to a decimal (or fraction).

The concept of finding angle by the length of the arc was used long ago. The radian was introduced much later. Roger Cotes gave the concept of radians in 1714, but he didn’t give it this name and just called it a circular measure of an angle.

The term “radians” was first used in 1873. This name, later, got universal attention and got authorization.

In this article, you will learn how to convert degrees to radians and the other way around (radians to degrees). Let’s take a look.

How to convert degrees to radians?

To convert degrees to radians, we multiply the given angle (in degrees) by π/180.

Angle in degrees (°) x π/180 = Angle in radian (Rad)

Where, π = 22/7 or 3.14

Example 1

Convert the following angles from degrees to radians

1. 0°
2. 30°
3. 45°
4. 60°
5. 90°
6. 120°
7. 150°
8. 180°
9. 210°
10. 240°
11. 360°

Solution

Angle in degrees (°) x π/180 = Angle in radian (Rad)

1. 0° x π/180

= 0 Rad

2. 30° x π/180

= π/6

= 0.5 Rad

3. 45° x π/180

= π/4

= 0.785 Rad

4. 60° x π/180

= π/3

= 1.047 Rad

5. 90° x π/180

= π/2

= 1.571Rad

6. 120° x π/180

= 2π/3

= 2.094 Rad

7. 150° x π/180

= 5π/6

= 2.618 Rad

8. 180° x π/180

= π

= 3.14 Rad

9. 210° x π/180

= 7π/6

= 3.665 Rad

10. 240° x π/180

= 3π/2

= 4.189 Rad

11. 360° x π/180

= 2π

= 6.283 Rad

Example 2

Convert 700 degrees to radians.

Solution

Angle in degrees (°) x π/180 = Angle in radian (Rad)

By substitution,

Angle in radian (Rad) = 700 x π/180.

= 35 π/9

= 12.21 Rad.

Example 3

Convert – 300° to radians.

Solution

Angle in radian = -300° x π/180.

= – 5π/3

= – 5.23 Rad

Example 4

Convert – 270° to radians.

Solution

Angle in radian = -270° x π/180.

= – 3π/2

= -4.71 Rad.

Example 5

Convert 43 degrees, 6 minutes, and 9 seconds to radians.

Solution

First express 43 degrees, 6 minutes, and 9 seconds to degrees only.

43° 6′ 9″ = 43.1025°

43.1025° x π/180 = Angle in radian

= 0.752 Rad.

Example 6

Convert 102° 45′ 54″ to radians.

Solution

102° 45′ 54″ is equal to 102.765°

Angle in radian = 102.765°x π/180.

= 1.793 Rad.

How to Convert Radians to Degrees?

To convert radians to degrees, multiply the radian by 180/ π. So, the formula is given by,

Angle in radian x 180/ π = Angle in degrees.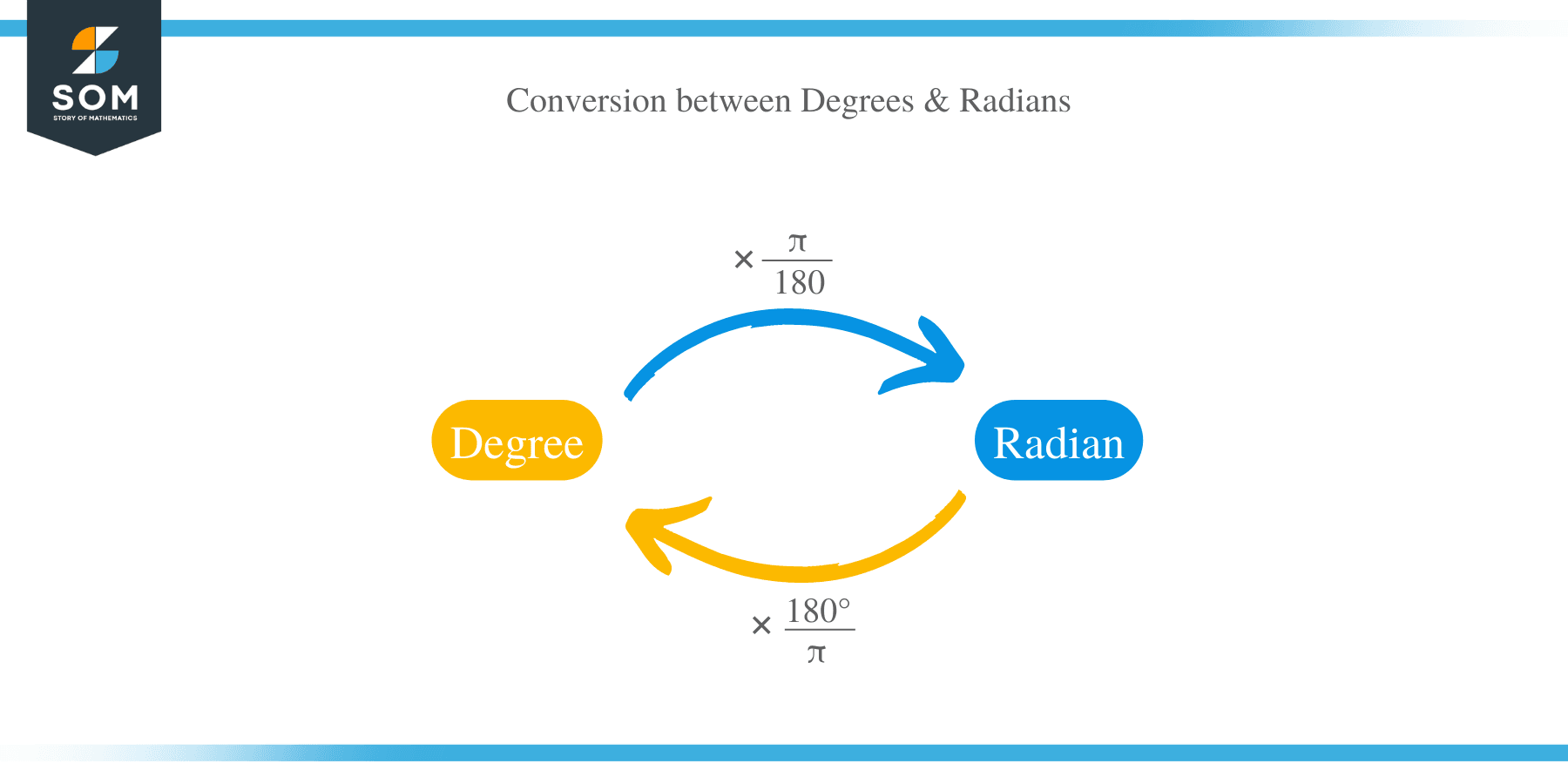

Example 7

Convert each of the following angles in radians to degrees.

1. 1.46
2. 11π/6
3. π/12
4. 3.491
5. 7.854
6. -8.14
7. π/180

Solution

Angle in radian x 180/ π = Angle in degrees.

1. 46 x 180/ π

= 83.69 degrees.

1. 11π/6 x 180/ π

= 330 degrees.

1. π/12 x 180/ π

= 15 degrees.

3. 491 x 180/ π

= 200.1 degrees

7. 854 x 180/ π

= 450.2 degrees.

8. -8.14 x 180/ π

= – 466.6 degrees.

1. π/180 x 180/ π

= 1 degree.

Example 8

Convert the angle π​/5 radians into degrees.

Solution

Angle in radian x 180/ π = Angle in degrees.

By substitution,

π​/5 x 180/ π = 36 degrees.

Example 9

Convert the angle – π​/8 radians into degrees

Solution

-π​/8 x 180/ π = – 22.5 degrees.

Example 10

The radius of a piece of pizza is 9 cm. If the perimeter of the piece is 36.850 cm, find the angle of the piece of pizza in radians and degrees.

Solution

Let arc length of the piece = x

Perimeter = 9 + 9 + x

36.850 cm = 18 + x

Subtract 18 on both sides.

18.85 = x

So, the arc length of the piece is 18.85 cm.

But, arc length = θr

Where θ = angle in radians and r =radius.

18.85 cm = 9 θ

Divide both sides by 9

θ =2.09 Rad

θ in degrees:

Angle in radian x 180/ π = Angle in degrees.

=2.09 x 180/ π

= 120 degrees.

Example 11

The radius of a sector is 3 m, and its area is 3π/4 m². Find the central angle of the sector in degrees and radians.

Solution

Given that,

Area of a sector = (r ²θ)/2

Where θ = central angle in radians.

Substitute.

3π/4 = (3² θ)/2

3π/4 = 9θ/2

Cross multiply.

6 π = 36 θ

Divide both sides by 36 to get,

θ = 0.52 Rad.

Convert the angle to degrees.

= 0.52 x 180/ π

= 29.8 degrees.

Example 12

Find the central angle of a sector whose radius is 56 cm and the area is 144 cm².

Solution

A= (θ/360) πr²

144 = (θ/360) x 3.14 x 56 x 56.

144 = 27.353 θ

Divide both sides by θ.

θ = 5.26

Thus, the central angle is 5.26 degrees.

Example 13

The area of a sector is 625 mm². If the radius of the sector is 18 mm, find the central angle of the sector in radians.

Solution

Area of a sector = (θr²)/2

625 = 18 x 18 x θ/2

625 = 162 θ

Divide both sides by 162.

θ = 3.86 radians.