Converting Fractions to Different Decimal Forms

In fractions, the number above the line is the numerator, and the number below the line is the denominator. The line or slash that separates the numerator and the denominator in a fraction represent division.

Conversion between fractions and decimals can be applied in our daily life when measuring out quantities. A fraction is usually used when determining how much of an ingredient is left in a pack.

However, electronic scales normally measure the weight of quantities in decimals. This makes conversion between fractions and decimals an important skill in cooking.

How to Convert Fractions to Decimals?

A fraction is made up of two parts: a numerator and a denominator. It is used to represent how many parts we have out of the total number of parts.

The line in a fraction that separates the numerator and denominator can be rewritten using the division symbol.

So, to convert a fraction to a decimal, here are the procedures on how to about:

• If the fraction is a mixed number, convert it to an improper fraction.
• The first step is to set up the fraction as a decimal division by dividing the top integer or numerator by the bottom integer (denominator).
• Continue the division by attaching the trailing zeros to the numerator so that you can either find a terminating or repeating decimal answer.

Example 1

4/5 as a fraction is calculated as: 4 ÷ 5 = 0.8

75/100 = 75 ÷100 = 0.75

3/6 = 3 ÷ 6 = 0.

When the Result is a Terminating Decimal

Sometimes, when dividing the numerator of a fraction by the denominator, the division terminates evenly. The results of this type of division are called a terminating decimal.

Below are examples of terminating decimals.

Example 2

2/5 = 2.0 ÷ 5

5 goes into 20 four times, and the decimal point goes in the same place in the top line.

The answer is therefore 0.4.

Example 3

4/25 = 4.00

4÷ 25

25 goes into 40 once, leaving 15 as a remainder.

25 goes into 150 six times exactly.

The answer is, therefore, 0.16.

Converting Fractions to a Recurring Decimal

Sometimes, the conversion of a fraction leads to a repeating decimal. The decimal recurs forever throughout the same number pattern.

For example, to convert 2/3 to a decimal, start by dividing 2 by 3. workout by adding 3 trailing zeros, and check the result.

You can notice that the division continues indefinitely no matter how many trailing zeros you attach to the number 2.

In this case, 2/3 = 0.666666…, a bar is normally placed above the repeating integer to show that the number recurs forever.

2/3 = 0.6¯

There comes a case where more than one integer recurs in the decimal number either consecutively or by alternating. For instance, suppose you want to convert 5/11 to a decimal fraction; here is how this problem works out:

5/11 = 0.45454545…..

It is noticed that the pattern repeats every integer 4 and 5. Adding more trailing zeros to the original decimal only string out the pattern indefinitely. So, you can represent as:

5/11 = 0.4¯5

In this case, the bar is placed above both numbers 4 and 5 to show that these two numbers alternate indefinitely.

Conversion of a Fraction to a Decimal when the Denominator is a Multiple of 10

When the denominator of a fraction is a multiple of 10, 100, 1000, 10000, etc., then convert the fraction to a decimal number is a straightforward process.

The numerator is written down and the decimal point is placed by counting the total number of zeros from the right to left.

Example 4

25/100 as a decimal = 0.25

276/1000 = 0.276

8/10 = 0.8

17/10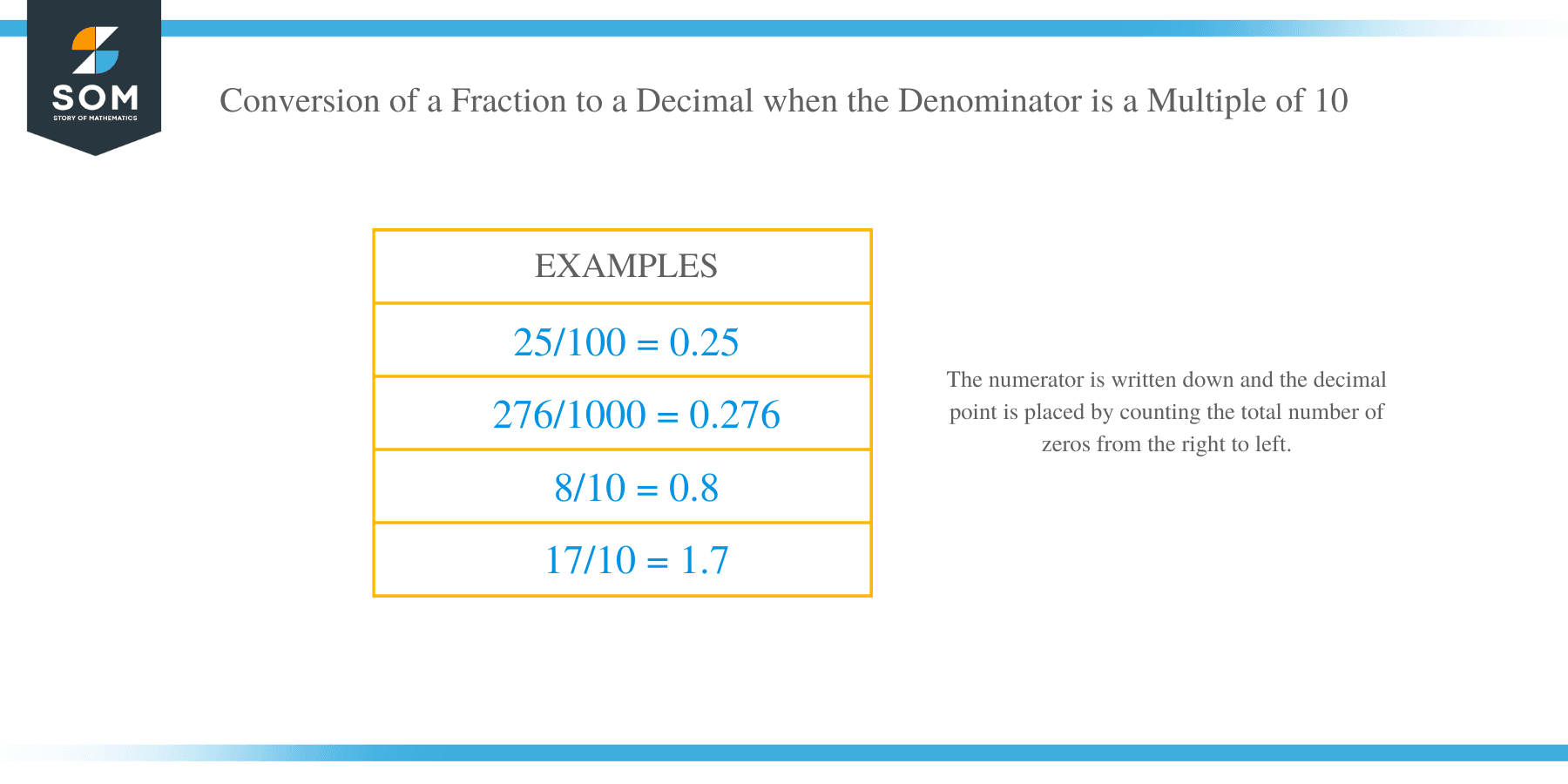

Example 5

Convert 7 5/8 to decimal

Solution

First convert the mixed fraction to an improper fraction
7 5/8 = (7 × 8 + 5)/8

= (56 + 5)/8

= 61/8

Therefore, 7 5/8 = 7.625