- Home
- >
- Conjugate math – Explanation and Examples
JUMP TO TOPIC
Conjugate Math – Explanation and Examples
 Ever seen two pairs of expressions that only differ by the sign in the middle? You may have encountered a pair of conjugates. Conjugates in math are extremely helpful when we want to rationalize radical expressions and complex numbers.
Ever seen two pairs of expressions that only differ by the sign in the middle? You may have encountered a pair of conjugates. Conjugates in math are extremely helpful when we want to rationalize radical expressions and complex numbers.
Two binomials are conjugates when they have the same terms but opposite signs in the middle.
This article will show how to find conjugates, understand why we need them, and apply them when rationalizing expressions. Let’s start with the most fundamental – understanding what conjugates represent.
What is a conjugate in math?
One example of a pair of conjugates is complex numbers a + bi and a – bi. Notice how the terms are the same? Only the signs found in the middle of each binomial differ. That’s exactly what conjugates in math represent.
Conjugate math definition
Conjugates in math are two pairs of binomials with identical terms but sharing opposite operations in the middle. Below are a few more examples of pairs of conjugates:
- x – y and x + y
- 2√2 – 1 and 2√2 + 1
- 3 – 2i and 3 + 2i
Consistent with the definition of conjugates, each pair have identical terms, and each only differs by the sign in the middle.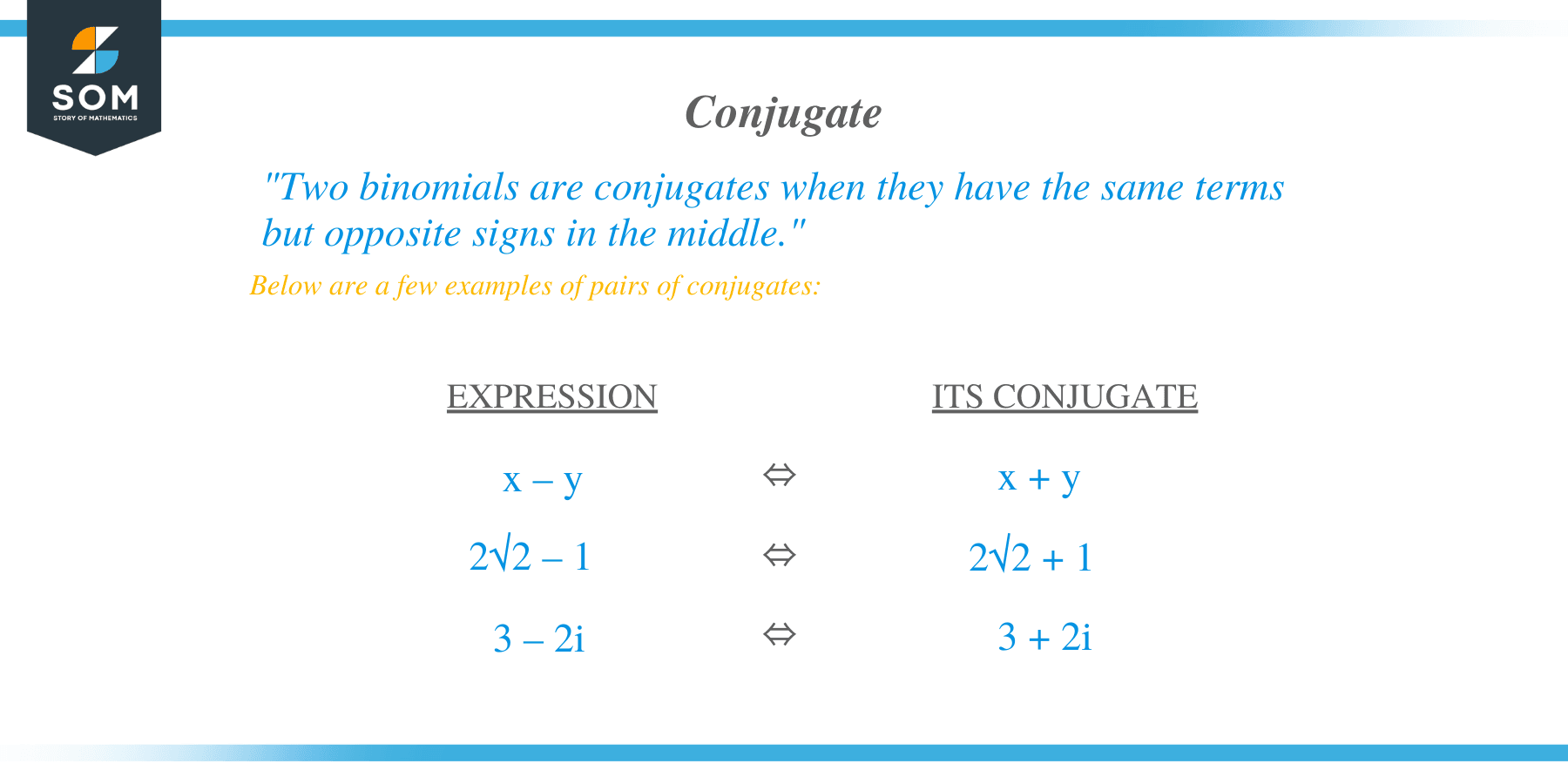
How to find the conjugate?
What if we’re given one binomial, and we need to find its conjugate? We can always determine a given binomial’s conjugate by first identifying the terms and the original term’s sign.
Once we have those, its conjugate will contain the same terms, but the middle sign is changed (from + to – or from – to +). Here’s a table showing how four binomials’ conjugates are determined:
| Given Binomial | Terms | Sign Change | Conjugate |
| 2x – y | 2x , y | – → + | 2x + y |
| √3 + 1 | √3, 1 | + → – | √3 – 1 |
| a2b – ab2 | a2b, ab2 | – → + | a2b + ab2 |
| 5 + 2i | 5, 2i | + → – | √3 – 1 |
How to multiply by the conjugate?
What happens if we multiply a binomial with conjugates? There two possible cases, and in each case, we’ll apply a different method.
Case 1: Multiplying a binomial with its conjugate
If we have a binomial, m + n, its conjugate will be m – n. Notice something about the two? These two, when multiplied, will return the difference of their squares. If you need a refresher on this algebraic property, take a look at this article. For our example, we have:
(m – n)(m + n) = m2 – n2
This means that when a binomial and its conjugate are multiplied together, the result will be the difference of the squares of their terms. Here are a few more examples you can try:
| Binomial | Conjugate | Product |
| 2x – 1 | 2x + 1 | 4x2 – 1 |
| 3ab + c | 3ab – c | 9a2b2 – c2 |
| √3 – 4 | √3 + 4 | 3 – 16 = -13 |
Case 2: Multiplying a binomial’s conjugate with a different expression
In some cases (such as rationalizing radical expressions), we might need to multiply a binomial’s conjugate with a different expression. When dealing with these problems, make sure to review your knowledge on:
- Multiplying two binomials by the FOIL method.
- When multiplying expressions with different terms, apply appropriate techniques.
- Another helpful property is using the technique of squaring binomials.
Let’s say we want to multiply √3 + 1 by the conjugate of √2 – 1. We’ll first have to find the conjugate of √2 – 1. We have √2 + 1. Since both √3 + 1 and √2 + 1 are binomials, we can apply the FOIL method to find and simplify the product of two binomials.
(√3 + 1)( √2 + 1) = (√3)( √2) + (√3)(1) + (1)( √2) + (1)(1)
= √6 + √3 + √2 + 1
It’s time that we now learn the common applications of conjugates in math.
How to rationalize radical expressions using conjugates?
One of the most common applications of conjugates occurs when we want to rationalize an expression that contains radical binomials in the denominator.
When we rationalize a rational expression, our goal is to have a denominator that contains no radical term. Conjugates come in handy when we have a binomial expression in the denominator.
We can multiply both numerator and denominator by the denominator’s conjugates. Why don’t we try one example and see what happens with the expression?
(√2 – 1) / (√2 + 1)
If we want to rationalize the expression shown above, we can multiply both numerator and denominator by the conjugate of the denominator.
(√2 – 1) / (√2 + 1) · (√2 – 1) / (√2 -1)
= [(√2 – 1) ·(√2 – 1)]/ [(√2 + 1)(√2 – 1)]
Simplify the denominator using the difference of two squares and simplify the numerator by squaring the expression (√2 – 1).
= [(√2)2 – 2(√2)(1) + (1)2]/[ (√2)2 – (1)2]
= [2 – 2√2 + 1]/[2 – 1]
= 3 – 2√2
The resulting expression no longer has any radical expressions on its denominator to confirm that it was rationalized using the denominator’s conjugate. Hence, we’ve seen how conjugates are used in rationalizing expressions.
Example 1
Find the conjugates of the following binomials.
a. 2xy – y
b. mn2 + m2n
c. ab – cd
Solution
Go back to the fundamental definition of conjugates: they share identical terms but different signs.
a. For 2xy – y, its conjugate will still have the same terms except that it has + as its operation. Hence, its conjugate is 2xy + y.
b. Similarly, we use the same terms but the opposite operation, so the conjugate of mn2 + m2n is mn2 – m2n.
c. Lastly, if we use the same process, we’ll find that the conjugate of ab – cd is ab + cd.
Example 2
When given a linear expression, ax + b, describe the result when:
a. the linear expression and its conjugate are added.
b. the linear expression’s conjugate is subtracted from it.
Solution
Let’s go ahead and find the conjugate of the linear expression first. Using the same terms but the opposite operation, the conjugate of ax + b is ax – b.
Adding the two binomials, we have ax + b + ax – b = 2ax. The sum is actually twice the value of the term.
a. In general, the sum of a linear expression and its conjugate is equal to twice the value of the binomial’s first term.
Let’s do the same for their difference. We have:
(ax + b) – (ax – b) = ax + b – ax + b
= 2b
The difference is the same as twice the second term of the binomial.
b. This means that when the conjugate of a binomial is subtracted from the binomial, the result is twice the binomial’s second term.
Example 3
Use your knowledge in conjugates to answer the following questions.
a. What is the conjugate of (1000 – 1)?
b. What is the result when you multiply (1000 – 1) by its conjugate?
c. Using what you’ve observed, describe how you can find the product of 81 and 79?
Solution
a. Using the same terms but the opposite operation, the conjugate of (1000 – 1) is (1000 + 1).
b. When we multiply a binomial with is conjugate, we square both terms and subtract the result. Hence, we have (1000)2 – 12 = 999 999.
c. This means that we can express 81 and 79 as conjugates of each other: 81 = 80 + 1 and 79 = 80 – 1. Using the two binomials, the product of 81 and 79 is 802 – 12 = 6399.
Example 4
Find the conjugate of the denominator, then rationalize the expression (-2 + √3) / (6 – 3√3).
Solution
Using the same terms but opposite operations, the conjugate of 6 – 3√3 is 6 + 3√3. Multiply both the numerator and denominator by the conjugate.
(-1 + √3) / (6 – 3√3) · (6 + 3√3)/ (6 +3√3)
= [(-1 + √3) (6 + 3√3)] / [(6 – 3√3) (6 + 3√3)]
Use the FOIL method to simplify the numerator and the difference of two squares in the denominator.
= [(-1)(6) + (-1) (3√3) + (√3)(6) + (√3)(3√3)]/ [(6)2 – (3√3)2]
= [-6 – 3√3 + 6√3 + 9]/ [36 – 27]
= (3√3 + 3)/ 9
= 3(√3 +1)/ 9
= 1/3 (√3 + 1)
We’ve just rationalized the given expression, and we now have 1/3 (√3 + 1).
