Condensing Logarithms – Properties, Explanation, and Examples

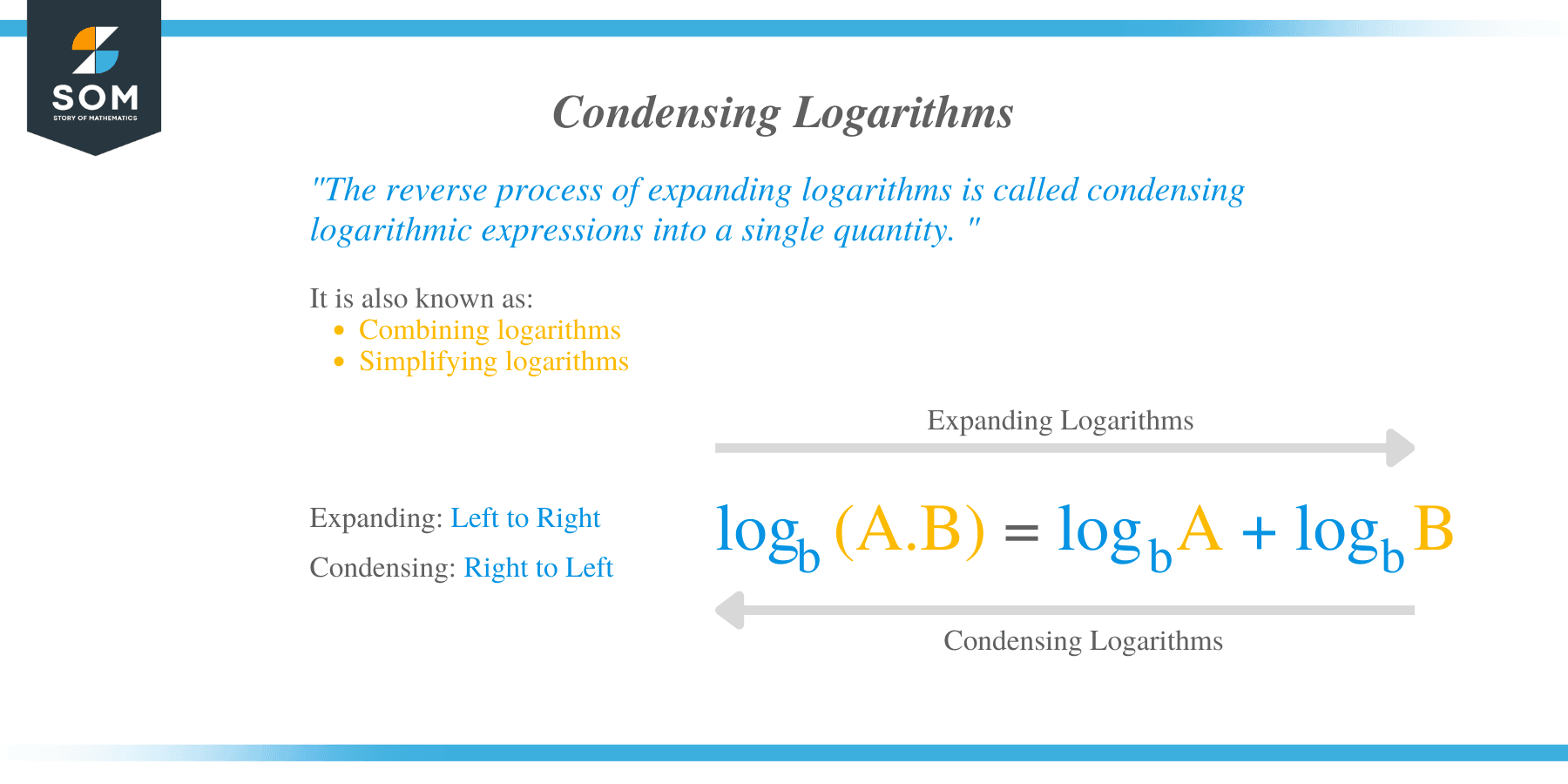
Condensing logarithms are helpful when we’re given a long logarithmic expression haring similar bases. This helps us simplify expressions size-wise and save space by combining the expressions that share common bases.Condensing logarithmic expressions is the process of using different logarithmic properties to combine different logarithmic terms into one quantity.This article makes use of various concepts we’ve learned in the past, so make sure to review these topics on logarithms before diving right into our main topic – condensing logarithms.

• Make sure to review the different parts and fundamental definitions of logarithms.
• Refresh the difference between common and natural logarithms.
• Learn how to apply the different logarithmic rules and properties.

The next section will show you how condensing logarithms is the opposite of expanding logarithms.How to condense logarithms?    When condensing logarithms, our goal is to compress the expressions altogether by using different logarithmic properties.\begin{aligned}\color{blue} \log_4 6 + 3\log_4 x – 3\log_4 y &\Leftrightarrow \color{green} \log_2 \left(\dfrac{6x^3}{y^3} \right ) \\ \phantom{xxxxx} \color{blue}\text{Expanded} &\Leftrightarrow \color{green} \boldsymbol{\text{Condensed}}\end{aligned}This is also why condensing logarithms is the reverse of expanding logarithmic expressions. The example above shows how the two processes are the opposite of each other.Here are some of the helpful rules that we might need to compress or condense logarithmic expressions.

Rule Name	Algebraic Expression
Product Rule	$\log_b({\color{blue} A}{\color{green} B}) = \log_b {\color{blue} A} + \log_b {\color{green} B}$
Quotient Rule	$\log_b\left(\dfrac{\color{blue} A}{\color{green} B}\right) = \log_b {\color{blue} A} – \log_b {\color{green} B}$
Product Rule	$\log_b {\color{blue}A}^n = n\log_b {\color{blue}A}$
Identity Rule	$\log_b b = 1$
Zero Rule	$\log_b 1 = 0$

To avoid getting overwhelmed with the different logarithmic properties, here are some helpful pointers to look out for:

• Whenever a factor is found outside the logarithm, see if you can apply the power rule right away.
• When combining the terms in between a subtraction or addition operation, check-in the quotient or product rule applies.
• Simplify $\log_1$ or $\log_b b$ using the zero or identity rules.
• The final answer is normally in terms of one rational expression, so double-check when you’re left with extra logarithmic terms.

The examples below will show you the common types of problems that involve condensing logarithms. Example 1Condense the logarithmic expression $\log_3 x + \log_3y – \log_3 z$ into a single logarithm. SolutionLet’s group the terms that are to be added up first, then condense them by using the product rule of logarithms. \begin{aligned}\log_3 x + \log_3y – \log_3 z&= (\log_3 x + \log_3y )- \log_3 z\\&= \log_3 xy – \log_3 z \color{green} \text{ Product Rule}\end{aligned}To further condense the expression into one single logarithm, let’s apply the quotient rule for logarithms.\begin{aligned}\log_3 xy – \log_3 z &= \log_3 \dfrac{xy}{z} \color{green} \text{ Quotient Rule}\end{aligned}This means that $\log_3 x + \log_3y – \log_3 z$ can be condensed into $\log_3 \dfrac{xy}{z}$.Example 2Condense the logarithmic expression $2\ln x – \dfrac{1}{2} \ln y$ into a single logarithm. SolutionWe can see that there are coefficients outside $\ln$ – $2$ and $\dfrac{1}{2}$, so we can use the power rule to rewrite the expression. Express $y^{\frac{1}{2}} as $\sqrt{y}$ as well. \begin{aligned}2\ln x – \dfrac{1}{2} \ln y&=\ln x^2 – \ln y^{\frac{1}{2}} \color{green} \text{ Power Rule}\\&=\ln x^2 – \ln \sqrt{y} \end{aligned}We are now left with the difference between the two terms to condense this further by applying the quotient rule.\begin{aligned}\ln x^2 – \ln \sqrt{y} &=\ln \dfrac{x^2}{\sqrt{y}} \color{green} \text{ Quotient Rule}\\&=\ln \left(\dfrac{x^2}{\sqrt{y}} \cdot \dfrac{\sqrt{y}}{\sqrt{y}}\right)\\&= \ln \dfrac{x^2\sqrt{y}}{y}\end{aligned}The last few steps are unnecessary, but we’re just showing you how the condensed expression would be like once the rational expression is rationalized. This means that $2\ln x – \dfrac{1}{2} \ln y$ is equal to $\ln \dfrac{x^2}{\sqrt{y}}$ or $\ln \dfrac{x^2\sqrt{y}}{y}$.Example 3Condense the logarithmic expression $\dfrac{1}{4} \log_4 x – 6\log_4 y – 5\log_4 z$ into a single logarithm. SolutionSince each term has coefficients before $\log$, we can apply the power rule to condense the logarithmic expression. We can then group the last two terms and apply the product rule to combine the two.\begin{aligned}\dfrac{1}{4} \log_4 x – 6 \log_4 y – 5\log_4 z &=\log_4 x^{\frac{1}{4}} – \log_4 y^6 – \log_4 z^5 \color{green} \text{ Power Rule}\\&=\log_4 x^{\frac{1}{4}} – (\log_4 y^6 + \log_4 z^5)\\&=\log_4 x^{\frac{1}{4}} – \log_4 y^6z^5 \color{green} \text{ Product Rule}\end{aligned}We can condense the expression further by applying the quotient rule. Keep in mind that $x^{\frac{1}{4}} = \sqrt[4]{x}$ as well.\begin{aligned}\log_4 x^{\frac{1}{4}} – \log_4 y^6z^5 &= \log_4 \dfrac{x^{\frac{1}{4}} }{y^6z^5}\color{green} \text{ Quotient Rule}\\&= \log_4 \dfrac{\sqrt[4]{x} }{y^6z^5}\end{aligned}Hence, we have $\dfrac{1}{4} \log_4 x – 6 \log_4 y – 5\log_4 z = \log_4 \dfrac{\sqrt[4]{x} }{y^6z^5}$.Example 4Condense the logarithmic expression $\ln x- 3[\ln (4x + 1)- \ln (3x -2)]$ into a single logarithm.SolutionLet’s condense the terms inside the bracket first by applying the quotient rule. We then apply the power rule so that $3$ becomes a power of the new condensed expression.\begin{aligned}\ln x – 3[\ln (4x + 1) – \ln(3x -2)] &= \ln x – 3\ln\left(\dfrac{4x + 1}{3x – 2}\right)\color{green} \text{ Quotient Rule}\\&=\ln x – \ln\left(\dfrac{4x + 1}{3x – 2}\right)^3\color{green} \text{ Power Rule}\end{aligned}We can further condense the expression by applying the quotient rule once more. Simplify the rational expression inside to return the final condensed form of the logarithmic expression.\begin{aligned}\ln x – \ln\left(\dfrac{4x + 1}{3x – 2}\right)^3 &= \ln \dfrac{x}{\left(\dfrac{4x + 1}{3x – 2}\right)^3} \color{green} \text{ Quotient Rule}\\&= \ln x \cdot \dfrac{(3x – 2)^3}{(4x + 1)^3}\\&= \ln \dfrac{x(3x – 2)^3}{(4x + 1)^3} \end{aligned}This means that $\ln x – 3[\ln (4x + 1) – \ln(3x -2)] =\ln \dfrac{x(3x – 2)^3}{(4x + 1)^3} $.Example 5Condense the logarithmic expression $\dfrac{1}{3}[3\log(x + 4) + \log(x – 1) – \log (x^2 -1)]$ into a single logarithm.SolutionLet’s go ahead and condense the expressions inside the bracket first. Let’s begin by applying the power rule in the first term. Use the quotient and product rules to compress the three groups of expression, as shown below. \begin{aligned}\dfrac{1}{3}[3\log (x + 4) + \log(x – 1) – \log (x^2 -1)] &= \dfrac{1}{3}[\log (x + 4)^3 + \log(x – 1) – \log (x^2 -1)] \color{green} \text{ Power Rule}\\&= \dfrac{1}{3}[\log (x + 4)^3 (x – 1) – \log (x^2 -1)] \color{green} \text{ Product Rule}\\&= \dfrac{1}{3}\left[\log \dfrac{(x + 4)^3 (x – 1)}{(x^2 – 1)}\right] \color{green} \text{ Quotient Rule} \end{aligned}We can factor out $x^2 -1$ using the difference of two squares property, $a^2 – b^2 = (a – b)(a + b)$. Cancel out common factors shared by the numerator and denominator. \begin{aligned}\dfrac{1}{3}\left[\log \dfrac{(x + 4)^3 (x – 1)}{(x^2 – 1)}\right] &= \dfrac{1}{3}\left[\log \dfrac{(x + 4)^3 (x – 1)}{(x – 1)(x + 1)}\right]\\&= \dfrac{1}{3}\left[\log \dfrac{(x + 4)^3 \cancel{(x – 1)}}{\cancel{(x – 1)}(x + 1)}\right] \\&=\dfrac{1}{3}\log \left[ \dfrac{(x + 4)^3}{x + 1}\right ]\end{aligned}Apply the power rule to further condense the logarithmic expression. Use the radical property, $x^{\frac{1}{n}} = \sqrt[n]{x}$.\begin{aligned}\dfrac{1}{3}\log \left[ \dfrac{(x + 4)^3}{x + 1}\right ] &= \log \left[ \dfrac{(x + 4)^3}{x + 1}\right ]^{\frac{1}{3}} \color{green} \text{ Power Rule}\\&= \log \sqrt[3]{\dfrac{(x + 4)^3}{x + 1}} \end{aligned}We can multiply both the numerator and denominator by $(x + 1)^2$ to rationalize the expression inside $\log$.\begin{aligned} \log \sqrt[3]{\dfrac{(x + 4)^3}{x + 1}} &= \log \sqrt[3]{\dfrac{(x + 4)^3}{x + 1} \cdot \dfrac{(x + 1)^2}{(x + 1)^2}}\\&=\log \sqrt[3]{\dfrac{(x + 4)^3(x + 1)^2}{(x + 1)^3}}\\&= \log \dfrac{\sqrt[3]{(x + 4)^3(x + 1)^2}}{x + 1} \end{aligned}This means that $\dfrac{1}{3}[3\log (x + 4) + \log(x – 1) – \log (x^2 -1)]$ is equal to $\log \sqrt[3]{\dfrac{(x + 4)^3}{x + 1}}$ or $\log \dfrac{\sqrt[3]{(x + 4)^3(x + 1)^2}}{x + 1}$.Example 6Condense the logarithmic expression $2\log_{9} 3 – 6\log_{9} 3 + \log_{9} \left(\dfrac{1}{243}\right)$ into a single logarithm then find its exact value.SolutionWe can apply the power rule to transfer the coefficients into the logarithmic expression. Rearrange the terms so that we can apply the product rule next.\begin{aligned} 2\log_9 3 – 6\log_9 3 + \log_9 \left(\dfrac{1}{243}\right)&= \log_9 3^2 – \log_9 3^6 + \log \left(\dfrac{1}{243}\right) \color{green}\text{ Power Rule}\\&= \log_9 3^2 + \log \left(\dfrac{1}{243}\right)- \log_9 3^6\\&= \log_9 \dfrac{3^2}{243} – \log_9 3^6\color{green}\text{ Product Rule}\end{aligned}We can further condense the expression by applying the quotient rule. Express $243$ as a power of $3$ then simplify the rational expression inside the logarithm.\begin{aligned} \log_9 \dfrac{3^2}{243} – \log_9 3^6 &= \log_9 \dfrac{3^2}{(243)(3^6)} \color{green}\text{ Quotient Rule}\\&=\log_9 \dfrac{3^2}{(3^5)(3^6)}\\&=\log_9 \dfrac{3^2}{3^{11}}\end{aligned}Simplify $\dfrac{3^2}{3^{11}}$ by subtracting the exponents, $2$ from $11$. Use the property, $\dfrac{1}{x^m} = x^{-m}$ to simplify the expression further.To find the exact value of the expression, it will be best to change the base of expression to $3$. Use the change of base formula, $\log_a x = \dfrac{\log_b x}{\log_b a}$ and the property, $\log_b b^x = x$, to evaluate the expression.\begin{aligned} \log_9 3^{-9} &= \dfrac{\log_3 3^{-9}}{\log_3 9}\\&=\dfrac{\log_3 3^{-9}}{\log_3 3^2} \\&= \dfrac{-9}{2}\\&= -\dfrac{9}{2}\end{aligned}Hence, $2\log_9 3 – 6\log_9 3 + \log_9 \left(\dfrac{1}{243}\right)$, once condensed, it equal to $\log_9 \dfrac{3^2}{3^{11}}$ which is equivalent to $-\dfrac{9}{2}$.