Completing the Square – Explanation & Examples

So far, you’ve learned how to factorize special cases of quadratic equations using the difference of square and perfect square trinomial method.

These methods are relatively simple and efficient; however, they are not always applicable to all quadratic equations.

In this article, we will learn how to solve all types of quadratic equations using a simple method known as completing the square. But before that, let’s have an overview of the quadratic equations.

A quadratic equation is a polynomial of second degree, usually in the form of f(x) = ax² + bx + c where a, b, c, ∈ R, and a ≠ 0. The term ‘a’ is referred to as the leading coefficient, while ‘c’ is the absolute term of f (x).

Every quadratic equation has two values of the unknown variable, usually known as the roots of the equation (α, β). We can obtain the root of a quadratic equation by factoring the equation.

What is Completing the Square?

Completing the square is a method of solving quadratic equations that we cannot factorize.

Completing the square means manipulating the form of the equation so that the left side of the equation is a perfect square trinomial.

How to Complete the Square?

To solve a quadratic equation; ax² + bx + c = 0 by completing the square.

The following are the procedures:

• Manipulate the equation in the form such that the c is alone on the right side.
• If the leading coefficient a is not equals to 1, then divide each term of the equation by a such that the co-efficient of x² is 1.
• Add both sides of the equation by the square of half of the co-efficient of term-x

⟹ (b/2a)².

• Factor the left side of the equation as the square of the binomial.
• Find the square root of both sides of the equation. Apply the rule (x + q) ² = r, where

x + q= ± √r

• Solve for variable x

Complete the square formula

In mathematics, completing the square is used to compute quadratic polynomials. Completing the Square Formula is given as: ax² + bx + c ⇒ (x + p)² + constant.

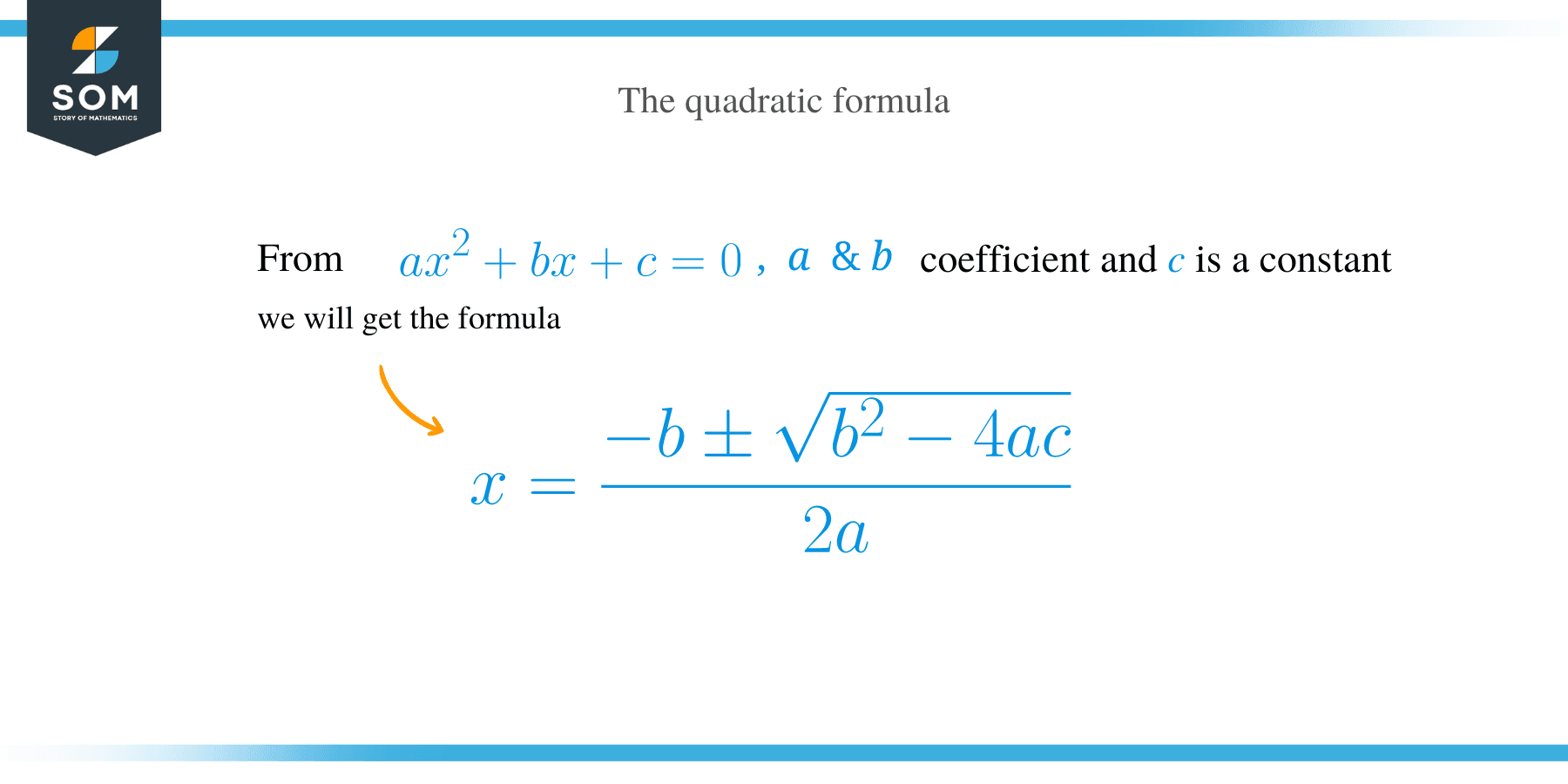
The quadratic formula is derived using a method of completing the square. Let’s see.

Given a quadratic equation ax² + bx + c = 0;

Isolate the term c to right side of the equation

ax² + bx = -c

Divide each term by a.

x² + bx/a = -c/a

Write as a perfect square
x ² + b​x/a + (b​/2a)² = – c/a ​+ (b/2a)²

(x + b/2a) ²= (-4ac+b²)/4a²

(x + b/2a) = ±√ (-4ac+b²)/2a

x = – b/2a ±√ (b²– 4ac)/2a

x = [- b ±√ (b²– 4ac)]/2a………. (This is the quadratic formula)

Now let’s solve a couple of quadratic equations using the completing square method.

Example 1

Solve the following quadrating equation by completing square method:

x² + 6x – 2 = 0

Solution

Transform the equation x² + 6x – 2 = 0 to (x + 3)² – 11 = 0

Since (x + 3)² =11

x + 3 = +√11 or x + 3 = -√11

x = -3+√11

OR

x = -3 -√11

But √11 =3.317

Therefore, x = -3 +3.317 or x = -3 -3.317,

x = 0.317 or x = -6.317

Example 2

Solve by completing square x² + 4x – 5 = 0

Solution

The standard form of completing square is;
(x + b/2)² = -(c – b²/4)

In this case, b = 4, c = -5. Substitute the values;
So, (x + 4/2)² = -(-5 – 4²/4)
(x + 2)² = 5 + 4
⇒ (x + 2)² = 9
⇒ (x + 2) = ±√9
⇒ (x + 2) = ± 3
⇒ x + 2 = 3, x + 2 = -3
⇒ x = 1, -5

Example 3

Solve x² + 10x − 4 = 0

Solution

Rewrite the quadratic equation by isolating c on the right side.

x² + 10x = 4

Add both sides of the equation by (10/2​)² = 5² = 25.

= x² + 10x + 25 = 4 + 25

= x² + 10x + 25 = 29

Write the left side as a square

(x + 5) ² = 29

x = -5 ±√29

x = 0.3852, – 10.3852

Example 4

Solve 3x² – 5x + 2 = 0

Solution

Divide each term of the equation by 3 to make the leading coefficient equals to 1.
x² – 5/3 x + 2/3 = 0
Comparing with the standard form; (x + b/2)² = -(c-b²/4)
b = -5/3; c = 2/3
c – b2/4 = 2/3 – [(5/3)2/4] = 2/3 – 25/36 = -1/36
Therefore,
⇒ (x – 5/6)² = 1/36
⇒ (x – 5/6)= ± √(1/36)
⇒ x – 5/6 = ±1/6
⇒ x = 1, -2/3

Example 5

Solve x² – 6x – 3 = 0

Solution

x² – 6x = 3
x² – 6x + (-3)² = 3 + 9

(x – 3)² = 12

x – 3= ± √12

x = 3 ± 2√3

Example 6

Solve: 7x² − 8x + 3=0

Solution

7x² − 8x = −3

x² −8x/7 = −3/7

x² – 8x/7 +(−4/7)² = −3/7+16/49

(x − 4/7)² = −5/49

x = 4/7 ± (√7) i/5

(x – 3)² = 12

x − 3 = ±√12

x = 3 ± 2√3

Example 7

Solve 2x² – 5x + 2 = 0

Solution

Divide each term by 2

x² – 5x/2 + 1 = 0

⇒ x² – 5x/2= -1

Add (1/2 × −5/2) = 25/16 to both sides of the equation.

= x² – 5x/2 + 25/16 = -1 + 25/16

= (x – 5/4)² = 9/16

= (x – 5/4)² = (3/4)²

⇒ x – 5/4= ± 3/4

⇒ x = 5/4 ± 3/4

x = 1/2, 2

Example 8

Solve x²– 10x -11= 0

Solution

Write the trinomial as a perfect square
(x² – 10x + 25) – 25 – 11 = 36

⇒ (x – 5)² – 36 =0

⇒ (x – 5)² = 36

Find the square roots on both sides of the equation

x – 5 = ± √36

x -5 = ±6

x = −1 or x =11

Example 9

Solve the following equation by completing the square

x² + 10x – 2 = 0

Solution

x² + 10x – 2 = 0

⇒ x² + 10x = 2

⇒ x² + 10x + 25 = 2 + 25

⇒ (x + 5)² = 27

Find the square roots on both sides of the equation

⇒ x + 5 = ± √27

⇒ x + 5 = ± 3√3

x = -5 ± 3√3

Example 10

Solve x² + 4x + 3 = 0

Solution

x² + 4x + 3 = 0 ⇒ x² + 4x = -3

x² + 4x + 4 = – 3 + 4

Write the trinomial as a perfect square

(x + 2)² = 1

Determine the square roots on both sides.

(x + 2) = ± √1

x= -2+1= -1

OR

x = -2-1= -3

Example 11

Solve the equation below using the method of completing the square.

2x² – 5x + 1 = 0

Solution

x²−5​x/2 + 1/2​=0

x² −5​x/2 = −1/2

(1/2​) (−5/2​) =−5​/4

(−5/4​)² = 25/16

x² − 5​x/2 + 25/16​ = −1/2​ + 25​/16

(x – 5/4) ² = 17​/16

Find the square of both sides.

(x – 5/4) = ± √ (17/16)

x = [5 ± √ (17)]/4