Combine Like Terms – Methods & Examples

Before discussing like and unlike terms, let’s take a quick review of an algebraic expression. In mathematics, an algebraic expression is a mathematical sentence made up of variables and constants, and operators such as addition and subtraction.

A variable in the expression is a term whose value is unknown, whereas a constant term has a definite value. The numerical number that accompanies a variable is called a coefficient. Examples of algebraic expressions are 3x + 4y -7, 4x – 10, 2x² − 3xy + 5 etc.

In this article, we will learn the meaning of like terms and how to combine them.

What does Combine Like Terms mean?

Terms in an algebraic expression are normally separated by addition or subtraction.

For instance, a monomial expression has only one term. For example, 3x, 5y, 4x, etc. Similarly, a binomial expression contains two terms, for instance, 3x + y, 2x + 7, x + y etc. A trinomial contains three terms, whereas polynomials of higher degrees contain many terms.

Like terms in Algebra are terms that contain identical variables and exponents, regardless of their coefficients. Like terms are combined in algebraic expression so that the result of the expression can be calculated with ease.

For example, 7xy + 6y + 6xy is an algebraic equation whose terms are 7xy and 6xy. Therefore, this expression can be simplified by combining like terms as 7xy + 6xy + 6y = 13xy + y. You can note that, when combining like terms, we only add the coefficients of the terms.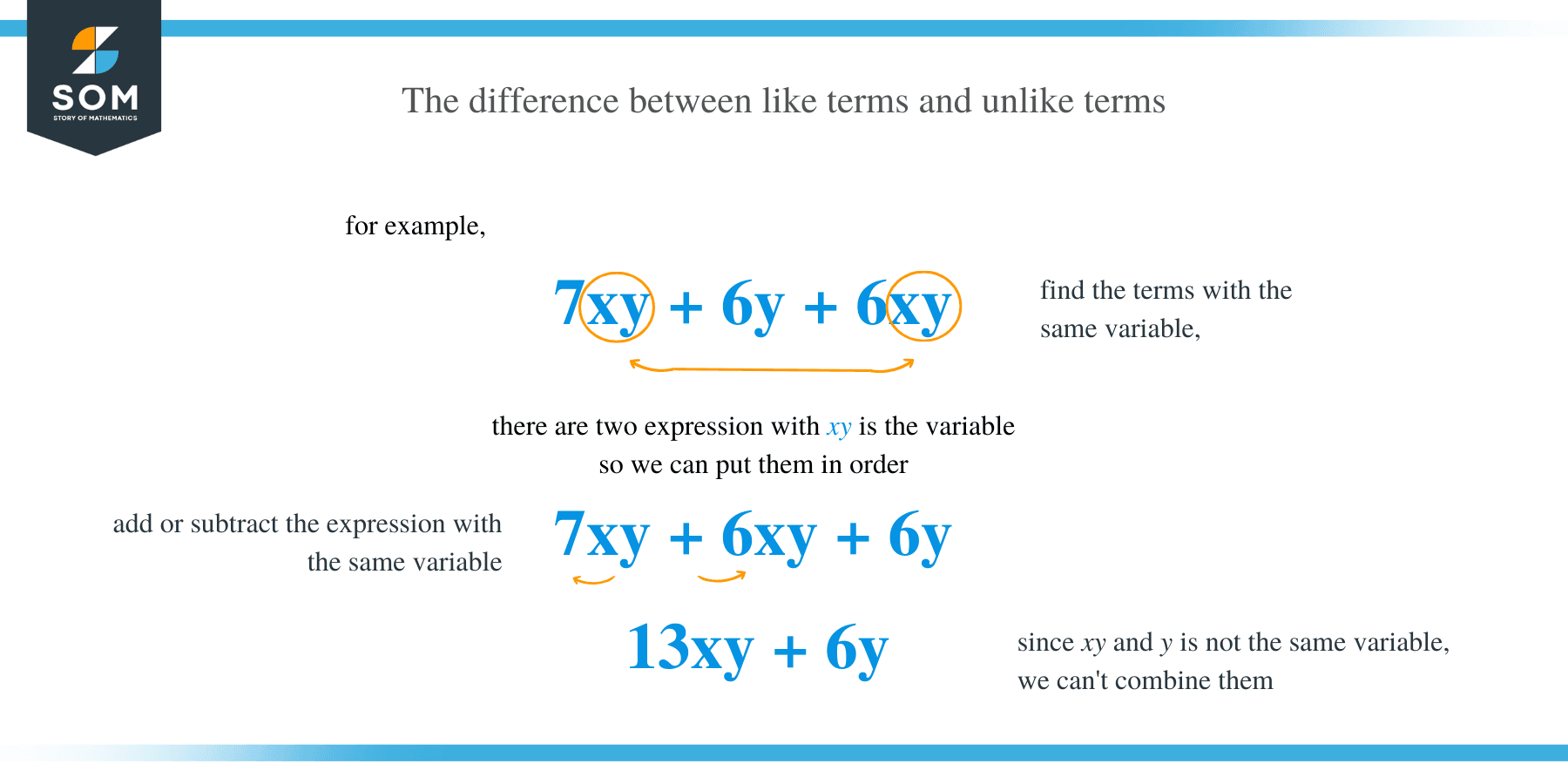

On the other hand, unlike terms are terms that do not have identical variables and exponents.

For example, an expression 4x + 9y contains terms because variables x and y are different and are not raised to the same power.

How to Combine the Like Terms?

Let’s understand this concept with the help of a few examples.

Example 1

Consider the expression: 4x + 3y.

This expression cannot be simplified because x and y are two different variables;

Example 2

To simplify an expression 4x² + 3x + 4y + 8x + 10x²;

Solution

Collect and add the like terms which gives; 10x² + 4x²+ 8x + 3x + 4y => 14x² + 11x + 4y.

From this example, we can conclude as the terms also have the same variables raised to the same exponent.

Example 3

Simplify 2xy + 4x² + 5yx +5y² +16x².

Solution

In this example, the terms 2xy and 5yx, as well as 4x² and 16 x² have identical variables. 2xy and 5yx are identical because of the commutative property of multiplication. Therefore, 2xy + 5yx = 7xy and 4x² +16x² = 20 x².

Hence, 2xy + 4x² + 5yx +5y² +16x² = 7xy + 20 x²

Example 4

Simplify 7m + 14m – 6n – 5n + 2m

Solution
Rewrite the expression so that the like terms are next to each other.
7m + 14m – 6n – 5n + 2m
Combine the coefficients.
(7 + 14 + 2) m + (-6 + -5) n
23m – 11n

Example 5

Simplify 2x² + 3x – 4 – x² + x + 9

Solution

Group the like terms according to their degree;

2x² + 3x – 4 – x² + x + 9

(2x² – x²) + (3x + x) + (–4 + 9)

(2 – 1) x² + (3 + 1) x + (5)

(1) x² + (4) x + 5

x² + 4x + 5

Example 6

10x³ – 14x² + 3x – 4x³ + 4x – 6

Solution

Group terms according to their degree or exponential;

10x³ – 14x² + 3x – 4x³ + 4x – 6

(10x³ – 4x³) + (–14x²) + (3x + 4x) – 6

6x³ – 14x² + 7x – 6

Example 7

[(6x – 8) – 2x] – [(12x – 7) – (4x – 5)]

Solution

Start simplifying from inside out;

[(6x – 8) – 2x] – [(12x – 7) – (4x – 5)]

[6x – 8 – 2x] – [12x – 7 – 1(4x) – 1(–5)]

[6x – 2x – 8] – [12x – 7 – 4x + 5]

[4x – 8] – [12x – 4x – 7 + 5]

4x – 8 – [8x – 2]

4x – 8 – 1[8x] – 1[–2]

4x – 8 – 8x + 2

4x – 8x – 8 + 2

–4x – 6

Example 8

Simplify the expression –4y – [3x + (3y – 2x + {2y – 7}) – 4x + 5]

Solution

Start from simplifying from the innermost grouping;

–4y – [3x + (3y – 2x + {2y – 7}) – 4x + 5]

–4y – [3x + (3y – 2x + 2y – 7) – 4x + 5]

–4y – [3x + (–2x + 3y + 2y – 7) – 4x + 5]

–4y – [3x + (–2x + 5y – 7) – 4x + 5]

–4y – [3x – 2x + 5y – 7 – 4x + 5]

–4y – [3x – 2x – 4x + 5y – 7 + 5]

–4y – [3x – 6x + 5y – 7 + 5]

–4y – [–3x + 5y – 2]

–4y – 1[–3x] – 1[+5y] – 1[–2]

–4y + 3x – 5y + 2

3x – 4y – 5y + 2

3x – 9y + 2