Yes, Business Calculus can be challenging for many students, but it is typically less intricate than the more theory-intensive calculus courses required for science and engineering majors. In my experience, Business Calculus focuses more on practical application and less on the theoretical aspects. It is designed for those pursuing a business degree, emphasizing methods relevant […]
Category Archives: Blog
To find the sum of an arithmetic sequence, I first identify the common difference between consecutive terms. This is because the essential feature of an arithmetic sequence is that each term increases by a steady amount from the one before. With this common difference and knowing the first term, the sequence is completely defined. […]
Algebra 2 and trigonometry serve as critical stepping stones in a student’s mathematical journey through high school and into college. I remember that uncovering the intricacies of Algebra 2’s equations and inequalities laid the groundwork for my understanding of more complex math concepts, serving as an essential prerequisite for other advanced courses. Whereas algebra provides […]
To use trigonometry to find lengths, I first identify the specific functions related to the sides of a right-angled triangle: sine, cosine, and tangent. These trigonometric ratios compare the lengths of two sides of the triangle, depending on the angle in question. When I have one side of a right-angled triangle and an angle, I […]
Yes, trigonometry is indeed a part of precalculus. In my study of mathematics, I’ve found that precalculus serves as the foundation that eases students into the complexities of calculus. Within this preparatory stage, trigonometry plays an integral role, furnishing students with the skills to understand and manipulate functions that describe angles, circles, and oscillations. I […]
Trigonometry is typically introduced to students during high school, often encountered after building a foundational understanding through subjects such as algebra and geometry. In most educational systems, this means that trigonometry is usually taught in the later years of high school, often during Algebra II or Pre-Calculus, which are courses that students take in 10th, 11th, […]
To learn trigonometry effectively requires a strategic approach. I’ve found that starting with the basics, like understanding the functions of sine, cosine, and tangent, lays a strong foundation. These functions relate the angles of a triangle to the lengths of its sides, which is the bedrock of trigonometry. Making these concepts intuitive is key to […]
What Does ‘exp’ Mean in Math? In mathematics, ‘exp‘ refers to the exponential function, which is the function ex, where e is Euler’s number, approximately equal to 2.71828. This function is fundamental in various areas of math, representing continuous growth or decay. Introduction In the world of mathematics, certain functions hold a special place due […]
What Is a Fact Family in Math? In mathematics, a fact family consists of a group of related arithmetic operations that connect a set of three numbers through addition and subtraction or multiplication and division. For example, in addition and subtraction, the numbers 2, 4, and 6 form a fact family: 2 + 4 = […]
What Is Unit Form in Math? In math, unit form refers to writing numbers to show the value of each digit, emphasizing place value by expressing the digits as units (ones, tens, hundreds, etc.). For example, the number 507 in unit form is written as 5 hundreds, 0 tens, and 7 ones. Introduction In the […]
What Does the Therefore (∴) Symbol Mean in Mathematics? The “therefore” symbol in mathematics, represented by three dots arranged in a triangular shape ( ∴ ), is used to denote that the statement following it is a logical consequence or result of the statements preceding it. It serves as a shorthand notation to signal the […]
What Does ‘Interpret’ Mean in Math? In math, ‘interpret‘ means to understand and give meaning to mathematical results or data, such as analyzing graphs, evaluating the significance of numerical outcomes, or explaining the real-world implications of a mathematical model. It involves translating mathematical language into everyday language and clarifying the practical impact or theoretical insight […]
What Does Inclusive Mean in Math? In mathematics, “inclusive” means that the endpoints of an interval or range are considered part of the solution. For example, in the interval [1, 10], the numbers 1 and 10 are included within the set. Introduction Inclusivity is a term that has gained significant attention across various domains, including […]
What Is Contemporary Math? Contemporary math refers to mathematics courses designed for liberal arts majors that emphasize practical applications of math in various fields such as economics, management, health, and social sciences. It often includes topics like logic, statistics, finance, and probability, rather than abstract algebra or advanced calculus. Introduction Contemporary mathematics, often referred to […]
What Does a Dot Mean in Math? In math, a dot typically represents multiplication between numbers or variables, a decimal point, a placeholder, or a vector dot product. In advanced mathematics, it can denote a derivative with respect to time when placed over a variable. Introduction In the language of mathematics, symbols play a crucial […]
Linear algebra often presents a notable challenge for many students, especially those encountering it for the first time at university level. We recognize it as a fundamental branch of mathematics, pivotal for various STEM fields like engineering and physics. Its reputation for being difficult primarily stems from the abstract nature of the concepts involved—such as […]
What Do You Learn in 3rd Grade Math? In 3rd grade math, students learn to master basic arithmetic operations—addition, subtraction, multiplication, and division—and are introduced to concepts like fractions, basic geometry, and measurement. They also develop problem-solving skills by applying these concepts to real-world scenarios. Introduction Third grade marks a significant stage in a child’s […]
What Do You Learn in 3rd Grade Math? In 4th grade math, students delve into multi–digit multiplication and division, expand their understanding of fractions and decimals, and explore more complex geometric concepts and measurements. They also begin to apply these skills to solve word problems and real-life mathematical scenarios. Introduction Fourth grade is a critical […]
What Do You Learn in 9th Grade Math? In 9th grade math, students typically engage with Algebra I, covering linear equations, inequalities, functions, and introductory geometry, or they may begin Geometry, focusing on proofs, theorems, and the properties of shapes. They also start to apply these concepts to more abstract and real-world problems, laying a […]
What Do You Learn in 5th Grade Math? In 5th grade math, students enhance their understanding of fractions and decimals, learn to perform operations with larger numbers and explore basic concepts of volume and geometry. They also apply their mathematical skills to real-world problems, such as using graphs and data to make decisions. Introduction Fifth […]
What Do You Learn in 10th Grade Math? In 10th grade math, students typically expand their knowledge in geometry, exploring more complex theorems and proofs, and diving deeper into algebra with quadratic equations, functions, and inequalities. They may also be introduced to trigonometry, focusing on the relationships between the angles and sides of triangles. Introduction […]
What Do You Learn in 6th Grade Math? In 6th grade math, students learn about fundamental concepts including fractions, decimals, percentages, basic algebra, and geometry. They also develop problem-solving and critical thinking skills through more advanced arithmetic and introduction to data analysis. Introduction Sixth-grade math is a critical milestone in a student’s mathematical journey. It […]
What Do You Learn in 7th Grade Math? In 7th-grade math, students learn fundamental concepts such as ratios, expressions, geometry, and data analysis, laying the foundation for more advanced math topics in the future. They also apply math to real-world scenarios, honing practical problem-solving skills. Seventh grade marks a crucial phase in a student’s mathematical […]
What Do You Study in 8th Grade Math? In 8th-grade math, students study advanced algebraic concepts, geometry, real and irrational numbers, probability, and functions, and apply these skills to practical scenarios. They also explore transformations, exponents, and equations with radicals, and strengthen their problem-solving abilities in preparation for high school math. Eighth grade marks a […]
In my experience, learning algebra is not just about adopting new mathematical tools, but also about changing my approach towards problem-solving. This transition can be difficult, as it requires a certain level of mental flexibility, which comes from consistent practice and patience. Furthermore, the role of education and teaching plays a significant part in cultivating […]
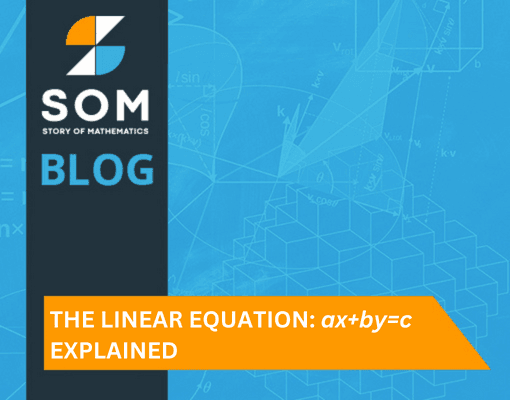
$ax+by=c$ is the standard form for linear equations in two variables. It is relatively simple to find both intercepts when an equation is provided in this form, that is $x$ and $y$. This type is also beneficial for resolving two linear equation systems. This complete guide will provide a detailed examination of the standard form, the […]
The Percentage of any given real number is defined as the relative real value which represents the hundredths parts of that given real number. It is represented by the symbol “%”. One Percent (expressed as 1%) corresponds to the hundredth part. Fifty Percent (expressed as 50%) corresponds to half of the given quantity and Hundred […]
The triangle proportionality theorem states that if we draw a line parallel to one side of a triangle so that it intersects the remaining two sides, then both sides are divided in the same proportion or divided equally. The triangle proportionality theorem is also known as the side-splitting theorem as it splits both sides into […]
The question, ‘How many sides does a circle have?‘ seems deceptively straightforward. Yet, it opens Pandora’s box of mathematical subtleties, leading to some of the most fundamental concepts in geometry. This article invites you to embark on a thought-provoking journey, aiming to explore this age-old question, shedding light on both traditional mathematical insights and modern […]
This article explores redexes, unveiling the mechanics behind transforming complex expressions into simpler ones. Definition In computer science and lambda calculus, a redex, short for ‘reducible expression,’ refers to a part of an expression that can be simplified or ‘reduced‘ according to certain rules. The concept of redexes is particularly central to lambda calculus, a […]
This article aims to delve deep into this captivating aspect of the concept of ‘r P n’ – permutations of ‘n’ items taken ‘r’ at a time, offering a comprehensive understanding of how the mathematics of ‘r P n’ is a cornerstone in both theoretical analysis and practical applications. Definition of r P n In […]
This article aims to provide an in-depth exploration of the concept of ln(0), demystifying its nature, its undefined status in real numbers, and its implications within the field of mathematics. Delving into the mathematical fabric that constitutes this unique expression, we aspire to bring clarity and understanding to this compelling aspect of logarithmic functions. Definition […]
This article aims to demystify how to divide radicals, providing a clear, detailed guide on handling such operations. We’ll break down the rules, use illustrative examples, and provide insights illuminating the path to mastering this important mathematical skill. Defining How to Divide Radicals Radicals, or roots, are mathematical expressions that represent a particular “root” of […]
What Does Xer Mean in Math? In mathematics, “xer” means an element that is neither a member of a given set nor outside of it, often used to resolve paradoxes related to self-reference in set theory, notably Russell’s Paradox. In the labyrinthine world of mathematics, where symbols and notations hold profound significance, there exists a […]
This article aims to illuminate the normal line, demystifying their theoretical origins, practical applications, and the sublime beauty inherent in their geometric simplicity. Definition of the Normal Line In geometry, the normal line is perpendicular to a given line, plane, or surface at a specific point of contact. When the context involves a curve or […]
What Math Do You Take In 11th Grade? Eleventh-grade mathematics is a critical year that builds on the foundation laid in previous years and prepares students for more advanced mathematical concepts. The math courses available at this stage can vary depending on your school’s curriculum and your academic track. Here’s an overview of the typical […]
This discussion aims to illuminate the mathematical properties of secant circles, their relationship with tangents, and the rich tapestry of applications they find in science, engineering, and everyday life. Definition of Secant Circle In geometry, a secant circle, with respect to another circle, is a circle that intersects the given circle at exactly two distinct […]
What Math Do You Take In 12th Grade? Most students’ last year of high school is the 12th, and the math courses offered to them at this point might differ from one institution to the next and from one academic track to another. Students in the 12th grade often have a variety of math courses […]
This article explores the non adjacent angles, demystifying their properties, their relationships, and their roles in shaping the geometric world as we know it. Defining Non Adjacent Angles Non adjacent angles are two or more angles that do not share a common side (ray) or a common vertex (endpoint of the rays). They are distinct […]
This article aims to take you on an immersive journey into the realm of direction cosines. We’ll unearth their definition, properties, and significant applications in various fields, revealing their profound impact on our comprehension and manipulation of multi-dimensional spaces. Definition of Direction Cosines In mathematics, particularly in vector calculus and physics, direction cosines are three […]
Who Added Letter to Math? The introduction of letters into mathematics was not the product of a single genius but rather a method refined over many centuries by many brilliant brains. The voyage of incorporating letters into mathematics has been a rich and collaborative undertaking, from the early inklings in ancient civilizations to the structured […]
This article explores the enthralling world of function addition, diving into its definition, properties, and varied applications. Whether you’re a math enthusiast seeking to enrich your understanding or a curious reader venturing into the captivating depths of calculus, this exploration of function sums aims to unravel its complexity, shedding light on how this concept helps […]
The derivative of $xln x $ is $ln x+1$. In mathematics, a derivative is the rate of change of a function with respect to a parameter. Derivatives are essential for solving differential equations and calculus problems. Throughout this complete guide, we will go over the steps for calculating the derivative of $xln x$. What Is […]
This article invites you to explore the cone net, its properties, application, and examples. Definition of Cone Net The cone net refers to a two-dimensional pattern that can be folded along its edges to create a three-dimensional shape, in this case, a cone. The cone net generally consists of a circle (representing the base of […]
This article explores the intriguing world of joint relative frequency, offering readers a clear and concise understanding of this statistical approach. We aim to provide a comprehensive understanding of how joint relative frequencies can contribute to making sense of the complex data systems surrounding us. Definition of Joint Relative Frequency Joint relative frequency is a […]
This article aims to elucidate the principles of scalar and vector projections, underscoring their importance and how these concepts provide vital tools for understanding multidimensional spaces. We will delve into their mathematical underpinnings, explore the differences between scalar and vector projections, and illustrate their real-world implications through various examples. Defining Scalar and Vector Projections In […]
This article will delve into the fascinating world of u-substitution in definite integrals, aiming to provide readers with a comprehensive understanding of its concept, application, and significance. We’ll unravel its intricacies, explore its properties, and demonstrate its utility with practical examples, offering a holistic view of this vital calculus tool. Definition of U Substitution Definite […]
This article aims to dive deep into monotonic sequences, demystifying their concept, properties, and significance in mathematical theory and its practical applications. Whether you’re a mathematics enthusiast or a curious learner, this exploration of monotonic sequences promises to enrich your understanding and appreciation of this intricate mathematical construct. Defining Monotonic Sequence In mathematics, a sequence […]
The Hardest Math Subject The “hardest math subject” is a very relative expression that may mean vastly different things to different people based on their own experiences, viewpoints, and skill sets. Choosing “the hardest math subject” is very subjective and depends on the learner’s background, aptitude, and interests. Typically, it encompasses advanced areas of mathematics […]
It is possible to multiply a $4times 2$ and a $2times4$ matrix, and the resulting matrix will be a $4times4$ matrix. In mathematics, a matrix refers to a rectangular arrangement or numbers table, expressions, or symbols arranged in columns and rows. On matrices, you can carry out different operations — for instance: addition, subtraction, multiplication, […]