Adding and Subtracting Rational Expressions – Techniques & Examples

Before jumping into the topic of adding and subtracting rational expressions, let’s remind ourselves what rational expressions are.

Rational expressions are expressions of the form f(x) / g(x) in which the numerator or denominator are polynomials, or both the numerator and the numerator are polynomials.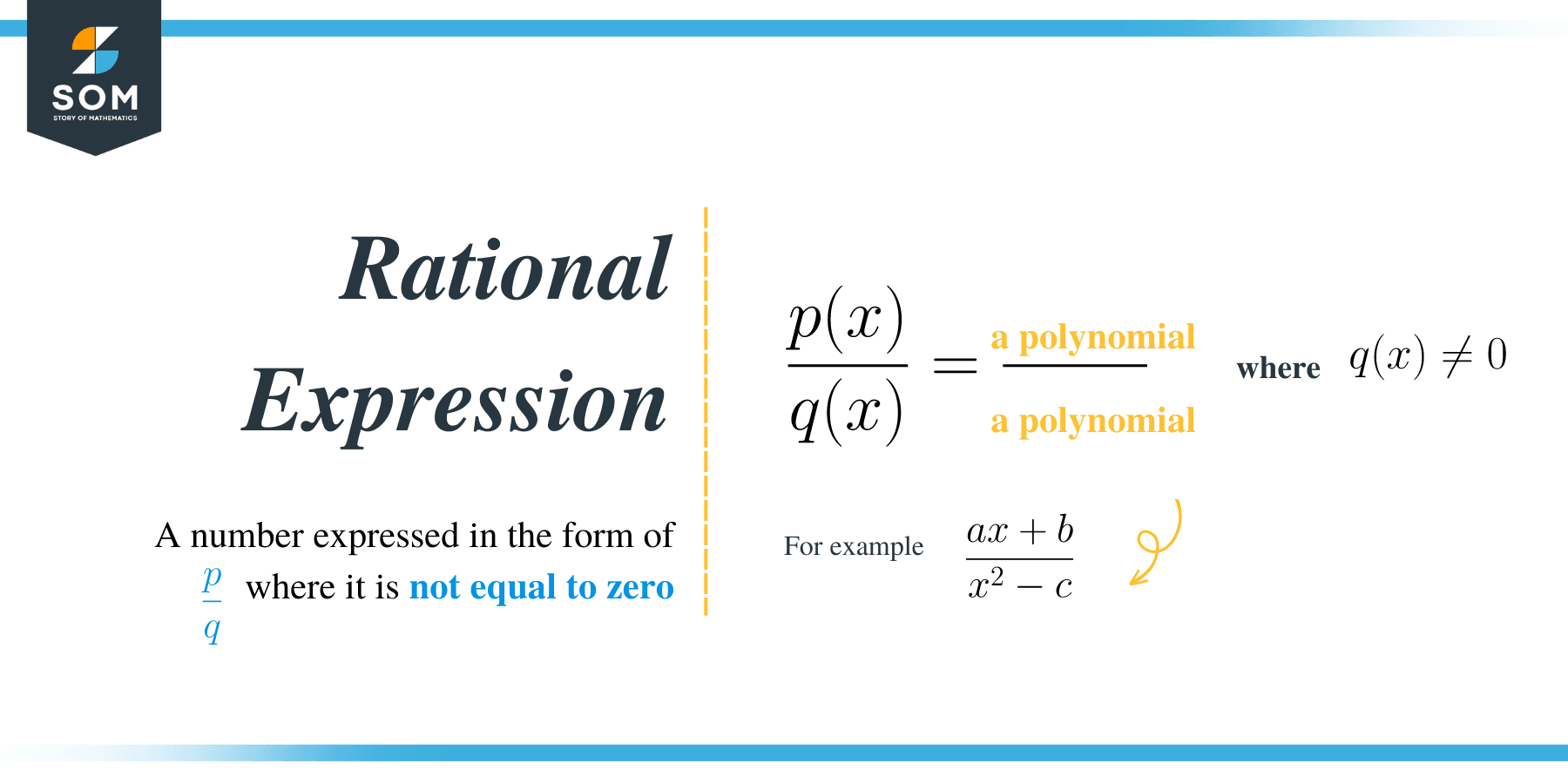

A few examples of rational expression are 3/(x – 1), 4/(2x + 3), (-x + 4)/4, (x² + 9x + 2)/(x + 3), (x + 2)/(x + 6), (x² – x + 5)/x etc.

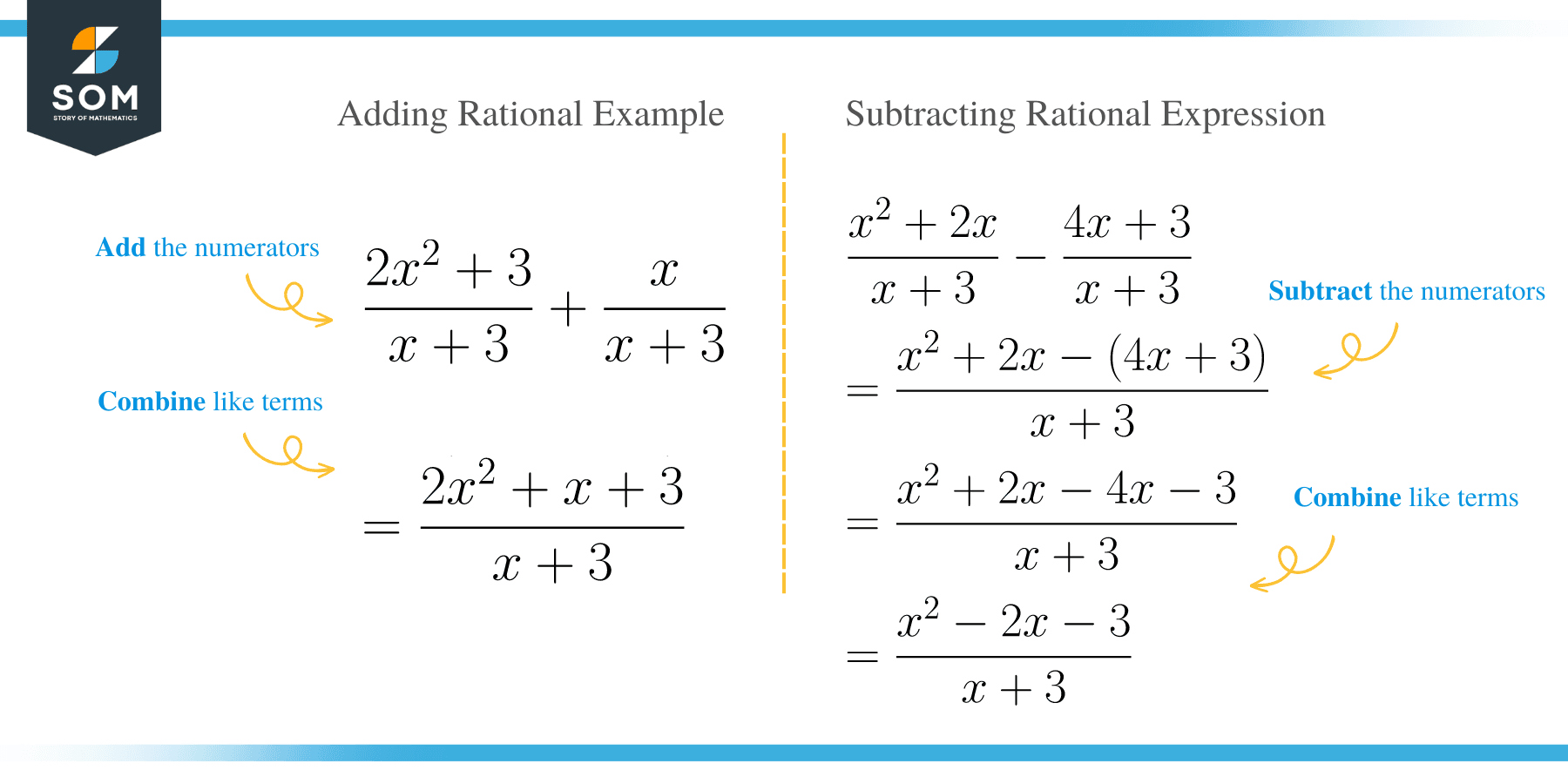
Adding and Subtracting Rational Expressions

To add or subtract rational expressions, we follow the same steps used for adding and subtracting numerical fractions.

Just like fractions, adding and subtracting rational expressions of the same denominator is performed by the formula given below:

a/c + b/c = (a + b)/c and a/c – b/c = (a – b)/c

If the denominators of rational expressions are different, we apply the following steps for adding and subtracting rational expressions:

• Factor the denominators to find the least common denominator(LCD)
• Multiply each fraction by the LCD and write the resultant expression over the LCD.
• By keeping the LCD, add or subtract the numerators. Remember to enclose the subtracting numerator in parentheses in order to distribute the subtraction sign.
• Factor the LCD and simplify your rational expression to the lowest terms

How to subtract rational expressions?

Below are a few examples regarding how to subtract the two rational expressions.

Example 1

Solve: 4/x+1 – 1/x + 1

Solution

Here, the denominators of both fractions are the same, therefore only subtract the numerators by keeping the denominator.

4/x+1 – 1/x + 1 = (4 – 1)/ 4/x + 1

= 3/x + 1

Example 2

Solve (5x – 1)/ (x + 8) – (3x + 8)/ (x + 8)

Solution

(5x – 1)/ (x + 8) – (3x + 8)/ (x + 8) = [(5x 1) – (3x + 4)]/ (x + 8)

Now remove the parentheses. Remember to distribute the negative sign accordingly.

= 5x – 1 – 3x – 4/ x +8

subtract the like terms to get;

= 2x -5/x + 8

Example 3

Subtract (3x/ x² + 3x -10) – (6/ x² + 3x -10)

Solution

The denominators are the same, therefore subtract the numerators only.

(3x/ x² + 3x -10) – (6/ x² + 3x -10) = (3x – 6)/ (x² + 3x -10)

Now factor both the numerator and the denominator to get;

⟹ 3(x -2)/ (x -2) (x + 5)

Simplify the fraction by cancelling out common terms in the numerator and denominator

⟹ 3/ (x + 5)

Example 4

Solve: 5/ (x – 4) – 3/ (4 – x)

Solution

Factor the denominators to get the LCD

5/ (x – 4) – 3/ (4 – x) ⟹ 5/ (x – 4) – 3/ -1(x – 4)

Therefore, the LCD = x – 4

Multiply each fraction by the LCD.

⟹ 5(x -4)/ (x – 4) – 3(x- 4)/ -1(x – 4)

= [5 – (-3)]/ x – 4

= 8/x -4

Example 5

Subtract (2/a) – (3/a −5)

Solution

The LCD of the fractions = a (a − 5)

Multiply each fraction by the LCD.

a (a − 5) (2/a) – a (a − 5) (3/a −5) = (2a – 10 – 3a)/a (a – 5)

= (-a -10)/ a (a – 5)

Example 6

Subtract 4/ (x² – 9) – 3/ (x² + 6x + 9)

Solution

Factor the denominator of each fraction to get the LCD.

4/ (x² – 9) – 3/ (x² + 6x + 9) ⟹ 4/ (x -3) (x + 3) – 3/ (x + 3) (x + 3)

Therefore, the LCD = (x -3) (x + 3) (x + 3)

Multiply each fraction by LCD to get;

[4(x + 3) – 3(x – 3)]/ (x -3) (x + 3) (x + 3)

Remove the parentheses in the numerator.

⟹ 4x +12 – 3x + 9/ (x -3) (x + 3) (x + 3)

⟹ x + 21/ (x -3) (x + 3) (x + 3)

Since there is nothing to cancel out, distribute the foil for the denominator to get;

= x + 21/ (x -3) (x + 3)²

How to add rational expressions?

Below are a few examples regarding how to add the two rational expressions.

Example 7

Add 6/ (x – 5) + (x + 2)/(x – 5)

Solution

6/ (x – 5) + (x + 2)/(x – 5) = (6 + x + 2)/(x -5)

Combine the like terms

= (8 + x)/(x – 5)

Example 8

Simplify (x-2)/(x + 1) + 3/x

Solution

LCD = x(x + 1)

Multiply each fraction by LCD

⟹ [x(x + 1)(x-2)/(x + 1) +  3x(x + 1)/x]/ x(x + 1)

= [x (x -2) + 3(x + 1)]/ x(x + 1)

Remove the parentheses in the numerator

= x² – 2x + 3x + 3/ x(x + 1)

Combine like terms;

⟹ x² – x + 3/ x(x + 1)

Example 9

Add 1 / (x – 2) + 3 / (x + 4).

Solution

There is nothing to factor out in the denominators, therefore we write the LCD as (x – 2)(x + 4).

Multiply each fraction by the LCD

⟹ 1(x – 2)(x + 4)/ (x – 2)) +  3(x – 2)(x + 4) / (x + 4)

= [1(x + 4) –  3(x -2)]/ (x + 4) (x – 2)

Now, remove the parentheses in the numerator

x + 4 – 3x + 6/ (x – 2)(x + 4).

Collect like terms in the numerator.

-x + 10/(x – 2)(x + 4).

There is nothing to factor out, so we FOIL for the denominator to get

= -x + 10 / (x² + 2x – 8)