Adding and Subtracting Polynomials – Explanation & Examples

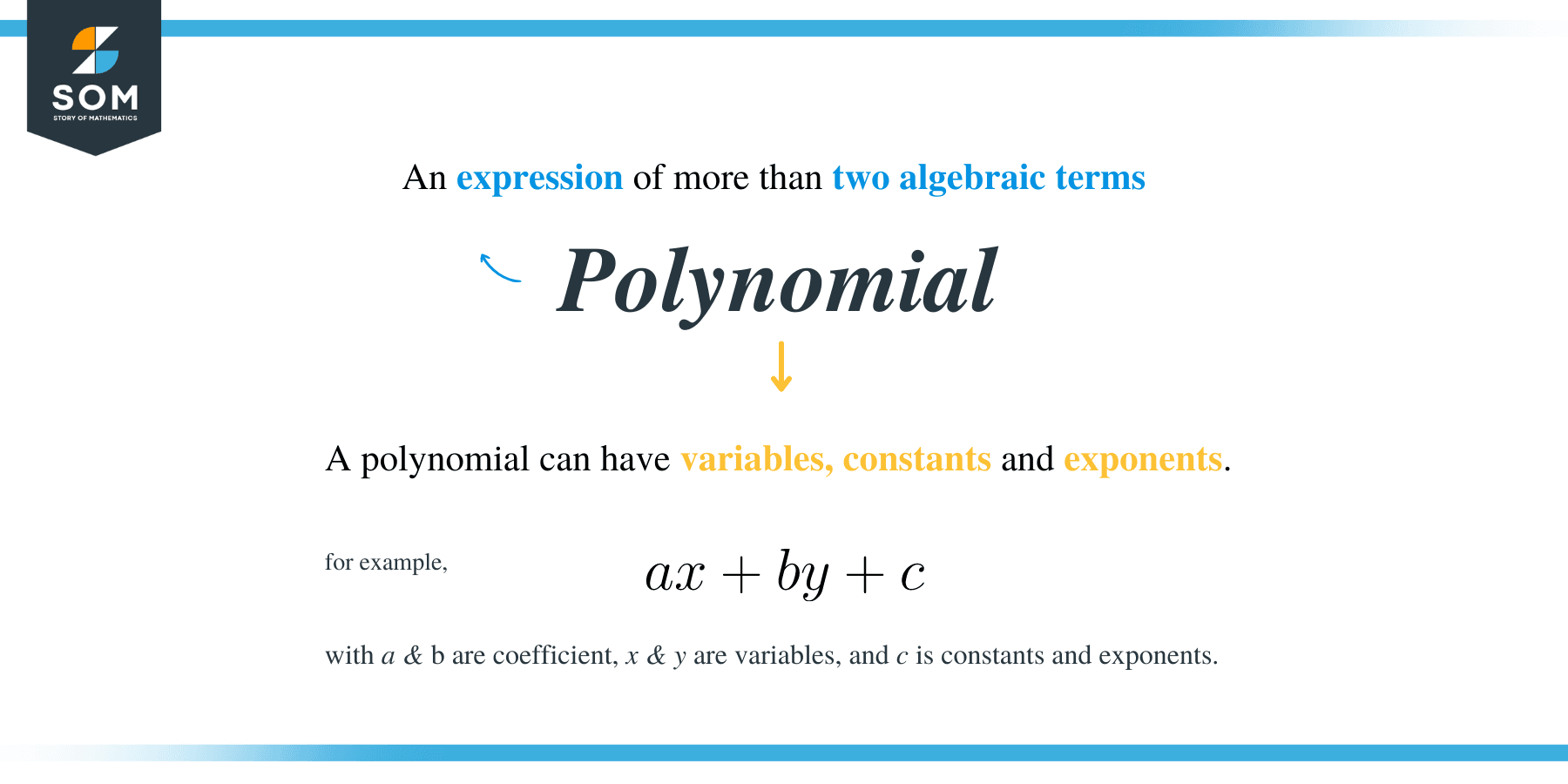
A polynomial is an expression that contains variables and coefficients.

For example, ax + b, 2x² – 3x + 9 and x⁴ – 16 are polynomials.

The word “polynomial” is derived from the words “poly” and “nomial,” which means many and terms respectively. A polynomial can have variables, constants, and exponents, but an expression is not a polynomial if the variable is in the denominator, like 2/x + 3, 9xy^-2, etc.

Like numbers, they can undergo the same type of operations. The operation of adding and subtracting polynomials is as easy as pie. You only need to be familiar with combining like terms and the order of operations within the question. Before we can begin, let’s us recall what like terms are.

In mathematics, like terms are terms that contain identical variables and exponents, regardless of their coefficients. You can simplify an expression by adding or subtracting depending on the signs before the terms.

For example, 7xy + 6y + 6xy is a polynomial whose terms are 7xy and 6xy. Therefore, we can simplify this polynomial by combining like terms as 7xy +6xy +6y = 13xy + y. When combining like terms, we only add or subtract the coefficients of the identical variables.

On the other hand, unlike terms are terms that are not identical in terms of either variables or exponents.

For example, an expression 4x + 9y², contain unlike terms because variable x and y are different and are not raised to the same power.

How to Add Polynomials?

The addition of polynomials involves arranging the like terms together and summing them up.

You can perform the operation by arranging the polynomials either vertically or horizontally. Whichever method you will use, the final answer will remain the same.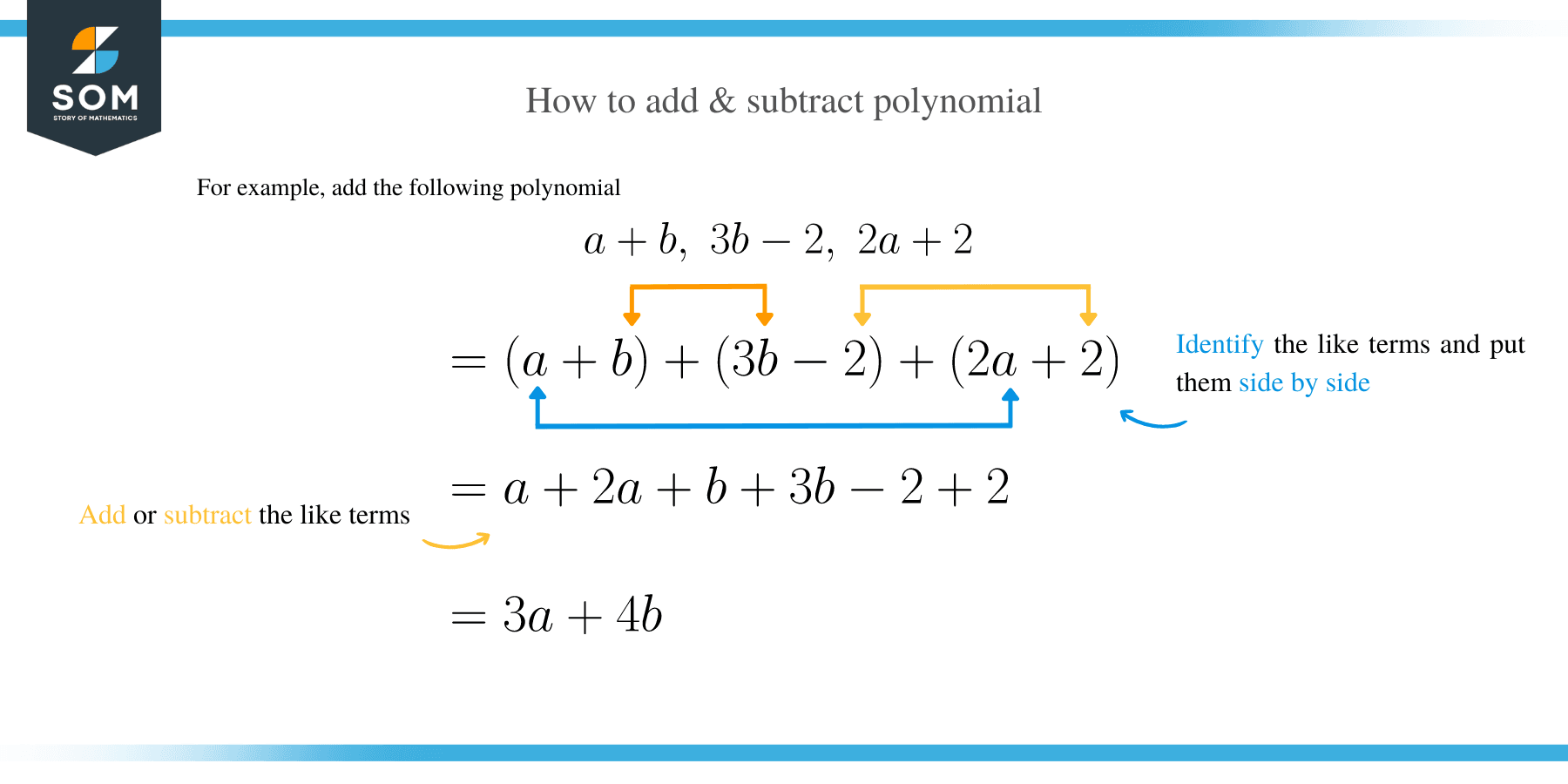

Example 1

Add the following polynomials:

5x + 3y, 4x – 4y + z and -3x + 5y + 2z

Solution

The first step is to combine the polynomials by the addition operators.

= (5x + 3y) + (4x – 4y + z) + (-3x + 5y + 2z)

= 5x + 3y + 4x – 4y + z – 3x + 5y + 2z

Now arrange the like terms together and add

= 5x + 4x – 3x + 3y – 4y + 5y + z + 2z

= 6x + 4y + 3z

Example 2

Add: 3a² + ab – b², -a² + 2ab + 3b² and 3a² – 10ab + 4b²

Solution

Combine the polynomials by the addition operators.
= (3a² + ab – b²) + (-a² + 2ab + 3b²) + (3a² – 10ab + 4b²)
= 3a² + ab – b² – a² + 2ab + 3b² + 3a² – 10ab + 4b²
Arrange the like terms together and then add
= 3a² – a² + 3a² + ab + 2ab – 10ab – b² + 3b² + 4b²
= 5a² – 7ab + 6b²

Example 3

Add the polynomials below.

15x³ – 6x – 23, 3x³ – 5x² + 8x + 10, -8x³ + 2x² – 7x and 9x² – 4x + 15

Solution

Combine the polynomials:

(15x³ – 6x – 23) + (3x³ – 5x² + 8x + 10) + (-8x³ + 2x² – 7x) + (9x² – 4x + 15)

Arrange the like terms together and add;

= (15x³ + 3x³ – 8x³) + (– 5x² + 2x² + 9x²) + (– 6x + 8x – 7x– 4x) + (– 23 + 10 +15)

= 10x³ + 6x² – 9x + 2

Example 4

Add: (3x³ – 5x + 9) + (6x³ + 8x – 7)

Solution

If the problem has parentheses, remove them by applying the distributive property of multiplication.

(3x³ – 5x + 9) + (6x³ + 8x – 7) ⟹ 3x³ – 5x + 9 + 6x³ + 8x – 7

Arrange the like terms together and add;

⟹ 3x³ + 6x³ + (-5x) + 8x + 9 + (-7)

= 9x³ + 3x + 2

Example 5

Add the following polynomial:

(2x^{2 +} 5x + 7) + (3x² −2x + 5)

Solution

Apply the commutative property to group like terms.

⟹ (2x² + 3x²) + (5x −2x) + (7 + 5)

Now use the distributive property.

⟹ (2 + 3) x² + (5−2) x + (7 + 5)

=5x² + 3x + 12

How to Subtract Polynomials?

Polynomials can be subtracted by either method. You can subtract by arranging the polynomials in a horizontal or vertical form.

To subtract polynomials horizontally, here are the steps:

• First, enclose the subtracting polynomial into brackets such that the minus sign is prefixed.
• Now remove the brackets by manipulating the sign in each term of a polynomial, i.e. (– changes to + and vice versa).
• Arrange the like terms together and add the likes together. We add instead of subtracting because the minus sign was changed when removing the parentheses.

NOTE: The polynomial or expression that comes before the word “from” is the subtracting quantity.

Example 6

Subtract the following polynomial 2x – 5y + 3z from 5x + 9y – 2z.

Solution

Enclose the subtracting polynomial and place a negative sign in front of the parentheses.

⟹ 5x + 9y – 2z – (2x – 5y + 3z)

Now open the parentheses by manipulating the signs

= 5x + 9y – 2z – 2x + 5y – 3z

= 5x – 2x + 9y + 5y – 2z – 3z

= 3x + 14y – 5z

Example 7

Subtract the polynomials below:

-6x² – 8y³ + 15z from x² – y³ + z.

Solution

Enclose the subtracting polynomial.

⟹ x² – y³ + z – (-6x² – 8y³ + 15z)

Remove the parentheses by changing the operators within the parentheses

= x² – y³ + z + 6x² + 8y³ – 15z

Arrange the like terms together.

= x² + 6x² – y³ + 8y³ + z – 15z

= 7x² + 7y³ – 14z

Example 8

Subtract: 3x³ + 5x² – 7x + 10 from 6x³ – 8x² + x + 10

Solution

Enclose the subtracting trinomial in parentheses

⟹ 6x³ – 8x² + x + 10 – (3x³ + 5x² – 7x + 10)

Remove the parentheses by changing the sign of each term inside the parentheses

⟹ 6x³ – 8x² + x + 10 – 3x³ – 5x² + 7x – 10)

Arrange the like terms and add to get;

= 3x³ – 13x² + 8x