Adding and Subtracting in Scientific Notation – Methods & Examples

Most students confuse exponential numbers with numbers in scientific notations. Numbers in exponential form can be added or subtracted when they are having the same base and exponent. On the other hand, numbers in scientific notation usually contain a common base but our doubt is about their exponents.

To add or subtract quantities in scientific notation, numbers are manipulated so that they contain similar bases and exponents. This is done in order to ensure the corresponding integers in their coefficients are in the same place value.

Multiplication of numbers is equivalent to finding the product of their coefficients and adding their exponents. With addition of scientific notations, rewrite quantities that are not matching by expressing the powers of 10 as the product of two smaller powers.

Similarly, if we want to retain the exponent of the number with the largest power of 10, multiply simultaneously the exponents and divide the coefficients. Once the numbers have been put under the same base and exponents, we can then add or subtract their coefficients.

The following illustrations will help you understand better the operation of adding and subtracting numbers in scientific notation.

How to Add in Scientific Notation?

Let’s understand this concept using a few examples below.

Examples 1

Add (4.5 x 10 ⁴) + (1.75 x 10 ⁴)

Explanation

• The quantities have similar exponents, therefore by using distributive property of multiplication, the numbers are factored out;
• (4.5 x 10 ⁴) + (1.75 x 10 ⁴) = (4.5 + 1.75) x 10 ⁴
• Add the coefficients and multiply by the power of 10
• (4.5 + 1.75) x 10 ⁴= 25 x 10 ⁴
• Therefore, (4.5 x 10 ⁴) + (1.75 x 10 ⁴) = 6.25 x 10 ⁴

Example 2

Add (7.5 x 10 ³) + (5.25 x 10 ⁵)

Explanation

• In this case, the powers of the quantities are different, we need to manipulate the power with a larger exponent.
• Therefore, the property of exponents; b ^m x b ⁿ = b ^{m + n} is used to rewrite the exponent of 10 ⁵ = 10 ² x 10 ³
• Now group the quantities: (7.5 x 10 ³) + (5.25 x 10 ⁵) = (7.5 x 10 ³) + (5.25 x 10 ² x 10³)

= (7.5 x 10 ³) + [(5.25 x 10 ²) x 10³]

• Add the coefficients: [(7.5 + 525) x 10 ³

= 532.5 x 10 ³

• Convert the number to scientific notation

= (5.325 x 10 ²) x 10 ³

= 5.325 x (10 ² x 10 ³)

= 5. 325 x 10 ⁵

How to Subtract in Scientific Notation?

Let’s understand this concept using a few examples below.

Example 3

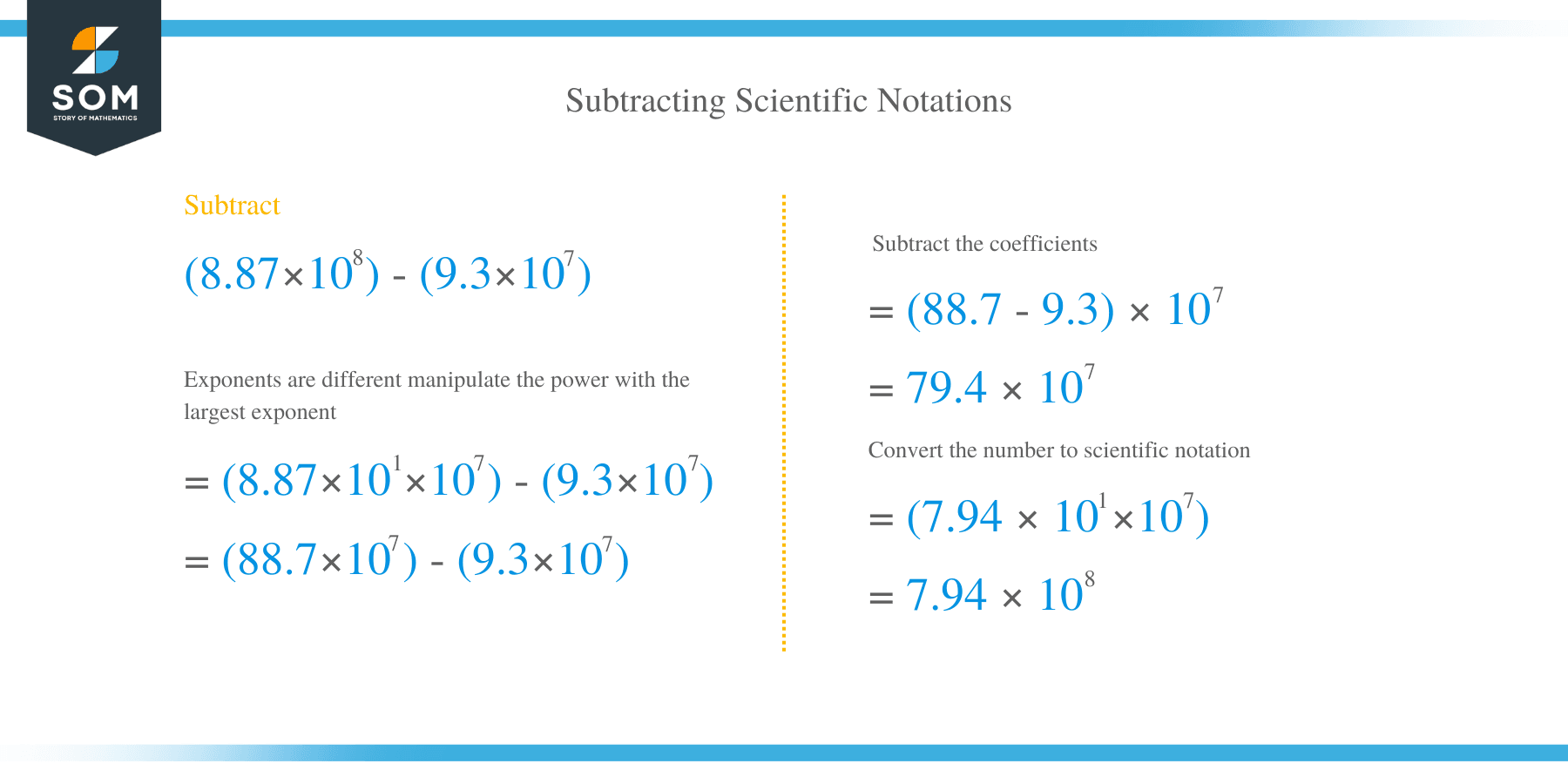
Subtract (8.87 × 10⁸) – (9.3 × 10⁷)

Explanation

• The quantities contain different exponents, manipulate the power with the largest exponent.

= (8.87 × 10¹ × 10⁷) – (9.3 × 10⁷)

= (88.7 × 10⁷) – (9.3 × 10⁷)

• Subtract the coefficients;

= (88.7 – 9.3) × 10⁷

= 79.4 × 10⁷

• Convert the number to scientific notation;

= 7.94 × 10¹ × 10⁷

• Therefore, (8.87 × 10⁸) – (9.3 × 10⁷) = 7.94 × 10⁸

Example 4

Subtract 0.0743 – 0.0022

Explanation

• First, convert the numbers to scientific notation

= (7.43 x 10 ^-3) – (92 .2 x 10 ^-3)

• Subtract the coefficients.
  = 7.43 – 0.22 = 7.21
• Join the new coefficient to the common power of 10.

= 7. 21 x 10 ^-2