Solve the Quadratic Equation 4x²-5x-12=0

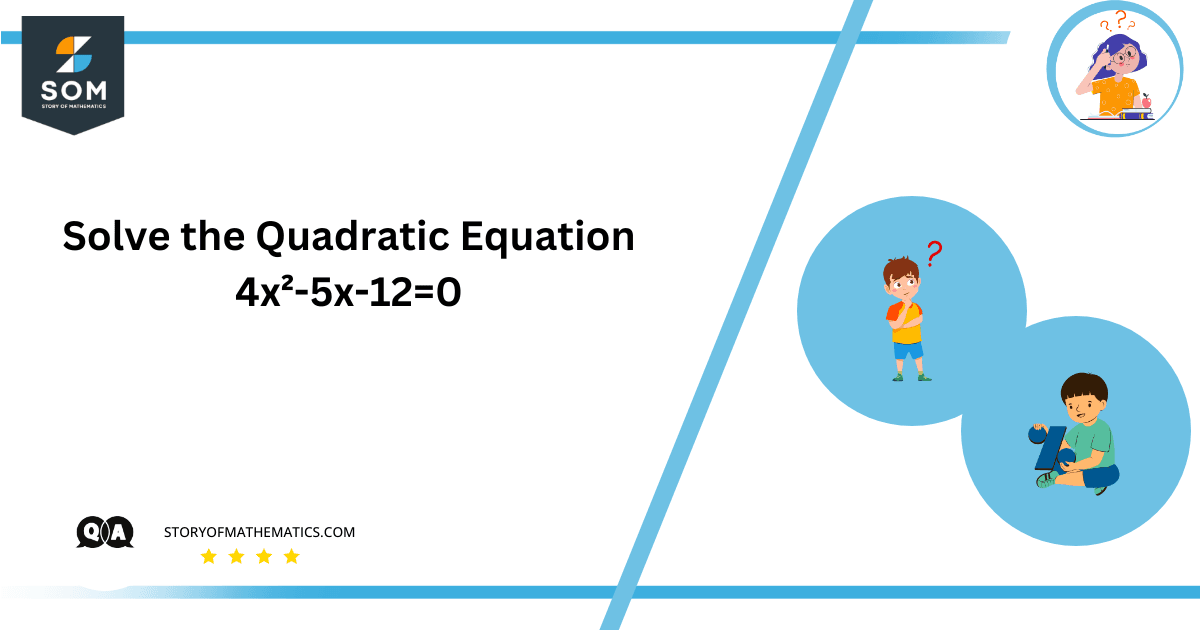
The roots for the given quadratic equation are x1 ≈ 2.46625 and x2 ≈ -1.21625. For solving the quadratic equation 4x² – 5x – 12 = 0 we apply the quadratic formula, this method is a steadfast solution strategy when facing any quadratic equation of the form ax² – bx – c = 0. Below we explain the detailed answer to solve the given quadratic equation.

Expert Answer

To solve the quadratic equation 4x² – 5x – 12 = 0, we can use the quadratic formula, which is:

x = (-b ± √(b² – 4ac)) / (2a)

Here, a, b, and c are coefficients from the equation, where a = 4, b = -5, and c = -12.

We start by calculating the discriminant (Δ):

Δ = b² – 4ac

Δ = (-5)² – 4×4×(-12)

Δ = 25 + 192

Δ = 217

The discriminant (Δ) is positive, indicating two distinct real solutions.

Using the discriminant, we apply the quadratic formula:

x = (5 ± √(217)) / 8

This gives us two solutions for x:

x₁ = (5 + √217) / 8

x₂ = (5 – √217) / 8

For a more precise calculation, we approximate √217:

√217 ≈ 14.73

Substituting this into the solutions, we get:

x1 ≈ (5 + 14.73) / 8

x1 ≈ 19.73 / 8

x1 ≈ 2.46625

and

x2 ≈ (5 – 14.73) / 8

x2 ≈ -9.73 / 8

x2 ≈ -1.21625

Numerical Result

So, the approximate solutions for the equation are:

x1 ≈ 2.46625 (or x1 ≈ 2.47 if rounded to two decimal places)

x2 ≈ -1.21625 (or x2 ≈ -1.22 if rounded to two decimal places)

Example

Solve the following quadratic equation: 3x² + 6x - 9 = 0

Solution

To solve the quadratic equation 3x² + 6x - 9 = 0, use the quadratic formula: x = (-b ± √(b² - 4ac)) / (2a). Here, the coefficients are a = 3, b = 6, and c = -9.

Calculate the discriminant (Δ):

Δ = b² - 4ac

Δ = 6² - 4*3*(-9)

Δ = 36 + 108

Δ = 144

Since the discriminant (Δ) is positive, there are two distinct real solutions.

Apply the quadratic formula:

x = (-6 ± √144) / (2*3)

This results in two solutions for x:

x₁ = (-6 + 12) / 6

x₁ = 1

and

x₂ = (-6 - 12) / 6

x₂ = -3

So, the solutions to the equation 3x² + 6x - 9 = 0 are x₁ = 1 and x₂ = -3. These are the x-values where the parabola represented by the equation would intersect the x-axis.