JUMP TO TOPIC
24 Times Table – Explanation & Examples
The 24 times table is the multiplication table of the number 24. The number 24 is important in our daily routine as we divide each day into 24 hours, so we may encounter many problems related to the multiples of 24 in daily life.
24 times table is a table that contains the multiples of the number 24.
Learning and understanding the 24 times table is essential for solving the multiplication, division, L.C.M, H.C.F, and factorization-related mathematical problems. In this topic, we will present some tips and patterns which will help you learn and memorize the 24 times table.
You should refresh the following concepts to understand the material discussed on this topic.
- Basics of addition and multiplication
- 4 times table
- 12 times table
- 20 times table
24 Multiplication Table
The table of 24 can be written as:
- $24\times 1 = 24$
- $24\times 2 = 48$
- $24\times 3 = 72$
- $24\times 4 =96$
- $24 \times 5 =120$
- $24 \times 6 =144$
- $24 \times 7 = 168$
- $24 \times 8 = 192$
- $24 \times 9 = 216$
- $24 \times 10 = 240$
Different Tips for the 24 Times Table:
Let us look at some simple tips which can help you memorize the 24 times table.
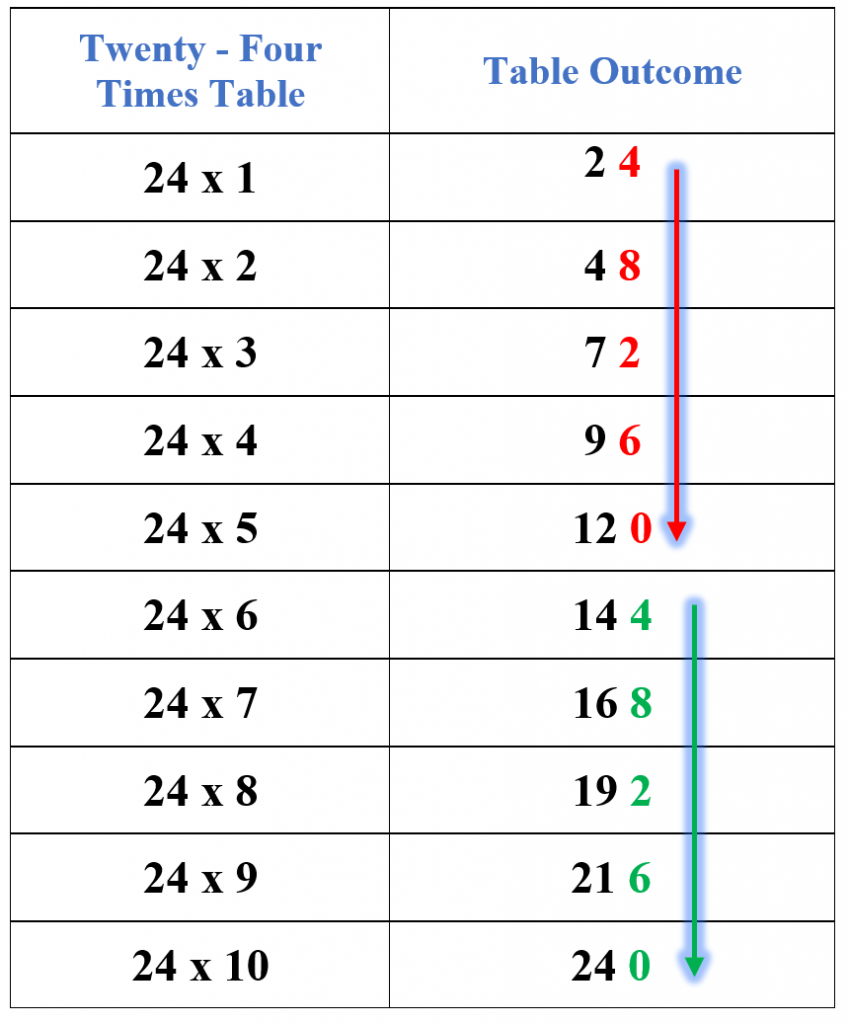
Digits Pattern: Similar to other multiples of 4, the 24 times table follows a digit pattern of five multiples, i.e., the digit pattern repeats itself after every five multiples. In this pattern, the ten’s digits of the first five multiples are 4, 8, 2, 6, and 0. The same sequence is repeated for the next five multiples and so on. The said pattern is shown in the table below.
Using the 20 and the 4 Times Tables: If you have learned and memorized the 20 and the 4 times tables, then this is one of the easiest methods to learn the 24 times tables. If we add the outcomes of the 20 times table to the outcomes of the 4 times table, the result is the 24 times table. For example, the third multiple of 20 is 60, and the third multiple of 4 is 12, and if we add 60 and 12, we get 72 which is the third multiple of 24. The detailed method is presented in the table below.
20 Times Table | 4 Times Table | Addition | Outcome |
| $20\times 1 = {\color{green}20}$ | $ 4 \times 1 = {\color{red}4}$ | ${\color{green}20}+ {\color{red}4}$ | $24$ |
| $20\times 2 = {\color{green}40}$ | $4 \times 2 = {\color{red}8}$ | ${\color{green}40}+ {\color{red}8}$ | $48$ |
| $20\times 3 = {\color{green}60}$ | $4 \times 3 = {\color{red}12}$ | ${\color{green}60} + {\color{red}12}$ | $72$ |
| $20\times 4 = {\color{green}80}$ | $4 \times 4 = {\color{red}16}$ | ${\color{green}80}+ {\color{red}16}$ | $96$ |
| $20\times 5 = {\color{green}100}$ | $4 \times 5 = {\color{red}20}$ | ${\color{green}100} +{\color{red}20}$ | $120$ |
| $20\times 6 = {\color{green}120}$ | $4 \times 6 = {\color{red}24}$ | ${\color{green}120} +{\color{red}24}$ | $144$ |
| $20\times 7 = {\color{green}140}$ | $4 \times 7 = {\color{red}28}$ | ${\color{green}140} +{\color{red}28}$ | $168$ |
| $20\times 8 = {\color{green}160}$ | $4 \times 8 = {\color{red}32}$ | ${\color{green}160} + {\color{red}32}$ | $192$ |
| $20\times 9 = {\color{green}180}$ | $4 \times 9 ={\color{red}36} $ | ${\color{green}180} + {\color{red}36}$ | $216$ |
$20\times 10 = {\color{green}200}$ | $4 \times 10 = {\color{red}40}$ | ${\color{green}200} + {\color{red}40}$ | $240$ |
Using the 12 Times Table: This method is quite simple, and it will help you revise the 12 times table as well. If you double the multiples of 12, you get the multiple of 24. For example, the sixth multiple of 12 is $12\times 6 = 72$, if we add the number to itself , we get $72 + 72 = 144$. We note that $144$ is the sixth multiple of 24. This method is presented in the table below.
12 Times Table | Double the Answer | 24 Times Table |
| $12\times 1 = {\color{green}12}$ | ${\color{green}12} + {\color{green}12} = {\color{red}24}$ | $24\times 1 = {\color{red}24}$ |
| $12\times 2 = {\color{green}24}$ | ${\color{green}24} + {\color{green}24} = {\color{red}48}$ | $24\times 2 = {\color{red}48}$ |
| $12\times 3 = {\color{green}36}$ | ${\color{green}36} + {\color{green}36} = {\color{red}72}$ | $24\times 3 = {\color{red}72}$ |
| $12\times 4 = {\color{green}48}$ | ${\color{green}48} +{\color{green}48} = {\color{red}96}$ | $24\times 4 = {\color{red}96}$ |
| $12\times 5 = {\color{green}60}$ | ${\color{green}60} + {\color{green}60} = {\color{red}120}$ | $24\times 5 = {\color{red}120}$ |
| $12\times 6 = {\color{green}72}$ | ${\color{green}72} + {\color{green}72} = {\color{red}144}$ | $24\times 6 = {\color{red}144}$ |
| $12\times 7 = {\color{green}84}$ | ${\color{green}84} + {\color{green}84} = {\color{red}168}$ | $24\times 7 = {\color{red}168}$ |
| $12\times 8 = {\color{green}96}$ | ${\color{green}96} + {\color{green}96} = {\color{red}192}$ | $24\times 8 = {\color{red}192}$ |
| $12\times 9 = {\color{green}108}$ | ${\color{green}54} + {\color{green}54} = {\color{red}216}$ | $24\times 9 = {\color{red}216}$ |
| $12\times 10 = {\color{green}120}$ | ${\color{green}60} + {\color{green}60} = {\color{red}240}$ | $24\times 10 = {\color{red}240}$ |
Table of 24 from 1 to 20:
A complete table of 24 from 1 to 20 can be written as:
Numerical Representation | Descriptive Representation | Product (Answer) |
$24 \times 1$ | Twenty-four times one | $24$ |
$24 \times 2$ | Twenty-four times two | $48$ |
$24 \times 3$ | Twenty-four times three | $72$ |
$24 \times 4$ | Twenty-four times four | $96$ |
$24 \times 5$ | Twenty-four times five | $120$ |
$24 \times 6$ | Twenty-four times six | $144$ |
$24 \times 7$ | Twenty-four times seven | $168$ |
$24 \times 8$ | Twenty-four times eight | $192$ |
$24 \times 9$ | Twenty-four times nine | $216$ |
$24 \times 10$ | Twenty-four times ten | $240$ |
$24 \times 11$ | Twenty-four times eleven | $264$ |
$24 \times 12$ | Twenty-four times twelve | $288$ |
$24 \times 13$ | Twenty-four times thirteen | $312$ |
$24 \times 14$ | Twenty-four times fourteen | $336$ |
$24 \times 15$ | Twenty-four times fifteen | $360$ |
$24 \times 16$ | Twenty-four times sixteen | $384$ |
$24 \times 17$ | Twenty-four times seventeen | $408$ |
$24 \times 18$ | Twenty-four times eighteen | $432$ |
| $24 \times 19$ | Twenty-four times nineteen | $456$ |
| $24 \times 20$ | Twenty-four times twenty | $480$ |
Example 1: If the price of an egg is $2. Calculate the price of 2 dozen eggs.
Solution:
We know that one dozen = 12, so 2 dozen eggs = 24 eggs.
The price of one egg is = $2
By using the 24 times table, we can calculate the price of 2 dozen eggs as $24 \times 2 = 48$ dollars.
Example 2: Calculate 24 times 7 minus 100 plus 10.
Solution:
24 times 7 minus 100 plus 10 can be written as:
$ = 24\times7 – 100 +10$
$ = 168 – 100+10$
$ = 68 +10$
$ = 78$
Example 3: What is the 7th multiple of 24?
Solution:
We know that the first 10 multiples of 24 are 24, 48, 72, 96, 120, 144, 168, 192, 216, and 240.
So, the 7th multiple is 168.
Example 4: Calculate 24 times 8 minus 300 plus 10 times 24.
Solution:
24 times 8 minus 300 plus 10 times 24 can be written as:
$ = (24\times8) – 300 +(10 \times 24)$
$ = 192 – 300+240$
$ = 192 – 60$
$ = 132$
Practice Questions:
1). Natallia has bought an electric bell. When she presses the button, the bell rings 24 times. Using the 24 times table, calculate how many times will the bell ring,
- If Natallia presses the button 4 times?
- If Natallia presses the button 14 times?
- If Natallia presses the button 11 times?
2). Find the value of “Y” if “$ Y \times 24 = 24 \times 12 – 576 + 24 \times 2 \times 10$.’’
3). From the given table select the numbers which are multiples of 24
| 24 | 38 | 18 | 21 | 51 | 269 | 192 |
| 41 | 34 | 72 | 96 | 19 | 240 | 111 |
| 48 | 172 | 98 | 94 | 99 | 104 | 211 |
| 120 | 131 | 49 | 121 | 118 | 113 | 47 |
| 159 | 260 | 216 | 52 | 114 | 112 | 61 |
| 09 | 141 | 168 | 79 | 86 | 89 | 134 |
| 91 | 173 | 171 | 179 | 65 | 215 | 360 |
| 99 | 156 | 154 | 456 | 151 | 336 | 138 |
| 221 | 84 | 77 | 51 | 227 | 196 | 266 |
| 464 | 480 | 55 | 59 | 160 | 220 | 270 |
Answer Key:
1). Bell rings 24 times if it is pressed once.
- By using the 24 times table we can calculate the number of times the bell will ring if it is pressed 4 times.
$24\times 4 = 96$ times.
- If the button is pressed 14 times then, $24\times 14 = 336$ times.
- If the button is pressed 11 times then, $24\times 11 = 264$ times.
2). $ Y \times 24 = 24 \times 12 – 576 + 24 \times 2 \times 10$
$ Y \times 24 = (24 \times 12) – 576 + (24 \times 2 \times 10) $.
$ Y \times 24 = 288 – 576 + ( 24\times 20) $.
$ Y \times 24 = 288 – 576 + 480$.
$ Y \times 24 = 288 – 96$.
$ Y \times 24 = 192$.
We know $24\times 8 = 192$, so
$ Y = 8 $.
3)
| 24 | 38 | 18 | 21 | 51 | 269 | 192 |
| 41 | 34 | 72 | 96 | 19 | 240 | 111 |
| 48 | 172 | 98 | 94 | 99 | 104 | 211 |
| 120 | 131 | 49 | 121 | 118 | 113 | 47 |
| 159 | 260 | 216 | 52 | 114 | 112 | 61 |
| 09 | 141 | 168 | 79 | 86 | 89 | 134 |
| 91 | 173 | 171 | 179 | 65 | 215 | 360 |
| 99 | 156 | 154 | 456 | 151 | 336 | 138 |
| 221 | 84 | 77 | 51 | 227 | 196 | 266 |
| 464 | 480 | 55 | 59 | 160 | 220 | 270 |