GEORG CANTOR – THE MAN WHO FOUNDED SET THEORY
Biography
 |
Georg Cantor (1845-1918) |
The German Georg Cantor was an outstanding violinist, but an even more outstanding mathematician. He was born in Saint Petersburg, Russia, where he lived until he was eleven. Thereafter, the family moved to Germany, and Cantor received his remaining education at Darmstradt, Zürich, Berlin and (almost inevitably) Göttingen before marrying and settling at the University of Halle, where he was to spend the rest of his career.
He was made full professor at Halle at the age of just 34, a notable accomplishment, but his ambitions to move to a more prestigious university, such as Berlin, were largely thwarted by Leopold Kronecker, a well-established figure within the mathematical community and Cantor’s former professor, who fundamentally disagreed with the thrust of Cantor’s work.
Meaning of Infinity
Cantor’s first ten papers were on number theory, after which he turned his attention to calculus (or analysis as it had become known by this time), solving a difficult open problem on the uniqueness of the representation of a function by trigonometric series. His main legacy, though, is as perhaps the first mathematician to really understand the meaning of infinity and to give it mathematical precision.
Back in the 17th Century, Galileo had tried to confront the idea of infinity and the apparent contradictions thrown up by comparisons of different infinities, but in the end shied away from the problem.
He had shown that a one-to-one correspondence could be drawn between all the natural numbers and the squares of all the natural numbers to infinity, suggesting that there were just as many square numbers as integers, even though it was intuitively obvious there were many integers that were were not squares, a concept which came to be known as Galileo’s Paradox.
He had also pointed out that two concentric circles must both be comprised of an infinite number of points, even though the larger circle would appear to contain more points. However, Galileo had essentially dodged the issue and reluctantly concluded that concepts like less, equals and greater could only be applied to finite sets of numbers, and not to infinite sets. Cantor, however, was not content with this compromise.
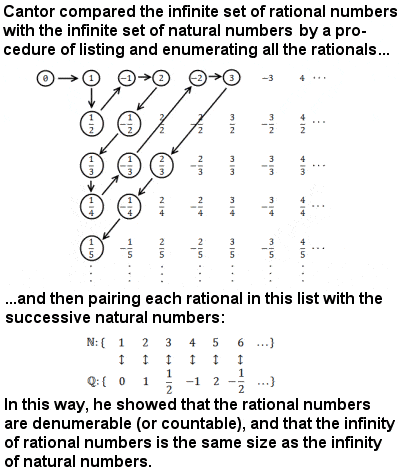 |
Cantor’s procedure of bijection or one-to-one correspondence to compare infinite sets |
Cantor’s starting point was to say that, if it was possible to add 1 and 1, or 25 and 25, etc, then it ought to be possible to add infinity and infinity. He realized that it was actually possible to add and subtract infinities, and that beyond what was normally thought of as infinity existed another, larger infinity, and then other infinities beyond that. In fact, he showed that there may be infinitely many sets of infinite numbers – an infinity of infinities – some bigger than others, a concept which clearly has philosophical, as well as just mathematical, significance. The sheer audacity of Cantor’s theory set off a quiet revolution in the mathematical community, and changed forever the way mathematics is approached.
His first intimations of all this came in the early 1870s when he considered an infinite series of natural numbers (1, 2, 3, 4, 5, …), and then an infinite series of multiples of ten (10, 20 , 30, 40, 50, …). He realized that, even though the multiples of ten were clearly a subset of the natural numbers, the two series could be paired up on a one-to-one basis (1 with 10, 2 with 20, 3 with 30, etc) – a process known as bijection – to show that they were the same “sizes” of infinite sets, in that they had the same number of elements.
This clearly also applies to other subsets of the natural numbers, such as the even numbers 2, 4, 6, 8, 10, etc, or the squares 1, 4, 9, 16, 25, etc, and even to the set of negative numbers and integers. In fact, Cantor realized that he could, in the same way, even pair up all the fractions (or rational numbers) with all the whole numbers, thus showing that rational numbers were also the same sort of infinity as the natural numbers, despite the intuitive feeling that there must be more fractions than whole numbers.
Cantor’s Diagonal Argument
 |
Cantor’s diagonal argument for the existence of uncountable sets |
However, when Cantor considered an infinite series of decimal numbers, which includes irrational numbers like π,eand √2, this method broke down. He used several clever arguments (one being the “diagonal argument” explained in the box on the right) to show how it was always possible to construct a new decimal number that was missing from the original list, and so proved that the infinity of decimal numbers (or, technically, real numbers) was in fact bigger than the infinity of natural numbers.
Aleph Null or Aleph Nought
He also showed that they were “non-denumerable” or “uncountable” (i.e. contained more elements than could ever be counted), as opposed to the set of rational numbers which he had shown were technically (even if not practically) “denumerable” or “countable”. In fact, it can be argued that there are an infinite number of irrational numbers in between each and every rational number. The patternless decimals of irrational numbers fill the “spaces” between the patterns of the rational numbers.
Cantor coined the new word “transfinite” in an attempt to distinguish these various levels of infinite numbers from an absolute infinity, which the religious Cantor effectively equated with God (he saw no contradiction between his mathematics and the traditional concept of God). Although the cardinality (or size) of a finite set is just a natural number indicating the number of elements in the set, he also needed a new notation to describe the sizes of infinite sets, and he used the Hebrew letter aleph (![]() ). He defined
). He defined ![]() 0 (aleph-null or aleph-nought) as the cardinality of the countably infinite set of natural numbers;
0 (aleph-null or aleph-nought) as the cardinality of the countably infinite set of natural numbers; ![]() 1 (aleph-one) as the next larger cardinality, that of the uncountable set of ordinal numbers; etc. Because of the unique properties of infinite sets, he showed that
1 (aleph-one) as the next larger cardinality, that of the uncountable set of ordinal numbers; etc. Because of the unique properties of infinite sets, he showed that ![]() 0 +
0 + ![]() 0 =
0 = ![]() 0, and also that
0, and also that ![]() 0 x
0 x ![]() 0 =
0 = ![]() 0.
0.
All of this represented a revolutionary step, and opened up new possibilities in mathematics. However, it also opened up the possibility of other infinities, for instance an infinity – or even many infinities – between the infinity of the whole numbers and the larger infinity of the decimal numbers. This idea is known as the continuum hypothesis, and Cantor believed (but could not actually prove) that there was NO such intermediate infinite set. The continuum hypothesis was one of the 23 important open problems identified by David Hilbert in his famous 1900 Paris lecture, and it remained unproved – and indeed appeared to be unprovable – for almost a century, until the work of Paul Cohen in the 1960s.
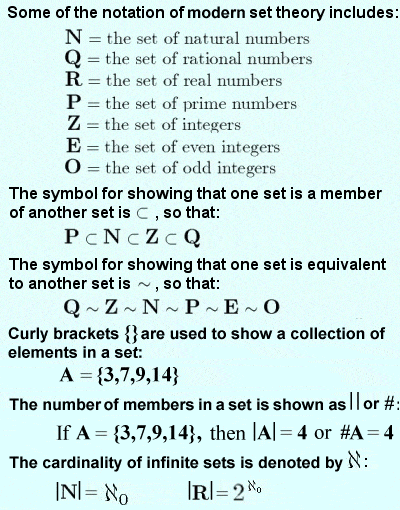 |
Modern set theory notation |
Just as importantly, though, this work of Cantor’s between 1874 and 1884 marks the real origin of set theory, which has since become a fundamental part of modern mathematics, and its basic concepts are used throughout all the various branches of mathematics. Although the concept of a set had been used implicitly since the beginnings of mathematics, dating back to the ideas of Aristotle, this was limited to everyday finite sets. In contradistinction, the “infinite” was kept quite separate, and was largely considered a topic for philosophical, rather than mathematical, discussion. Cantor, however, showed that, just as there were different finite sets, there could be infinite sets of different sizes, some of which are countable and some of which are uncountable.
Throughout the 1880s and 1890s, he refined his set theory, defining well-ordered sets and power sets and introducing the concepts of ordinality and cardinality and the arithmetic of infinite sets. What is now known as Cantor’s theorem states generally that, for any set A, the power set of A(i.e. the set of all subsets of A) has a strictly greater cardinality than Aitself. More specificially, the power set of a countably infinite set is uncountably infinite.
Despite the central position of set theory in modern mathematics, it was often deeply mistrusted and misunderstood by other mathematicians of the day. One quote, usually attributed to Henri Poincaré, claimed that “later generations will regard Mengenlehre (set theory) as a disease from which one has recovered”. Others, however, were quick to see the value and potential of the method, and David Hilbert declared in 1926 that “no one shall expel us from the Paradise that Cantor has created”.
Cantor had few other mathematicians with whom he could discuss his ground-breaking work, and most were distinctly unnerved by his contemplation of the infinite. During the 1880s, he encountered resistance, sometimes fierce resistance, from mathematical contemporaries such as his old professor Leopold Kronecker and Henri Poincaré, as well as from philosophers like Ludwig Wittgenstein and even from some Christian theologians, who saw Cantor’s work as a challenge to their view of the nature of God. Cantor himself, a deeply religious man, noted some annoying paradoxes thrown up by his own work, but some went further and saw it as the wilful destruction of the comprehensible and logical base on which the whole of mathematics was based.
As he aged, Cantor suffered from more and more recurrences of mental illness, which some have directly linked to his constant contemplation of such complex, abstract and paradoxical concepts. In the last decades of his life, he did no mathematical work at all, but wrote extensively on his two obsessions: that Shakespeare’s plays were actually written by the English philosopher Sir Francis Bacon, and that Christ was the natural son of Joseph of Arimathea. He spent long periods in the Halle sanatorium recovering from attacks of manic depression and paranoia, and it was there, alone in his room, that he finally died in 1918, his great project still unfinished.
<< Back to Boole | Forward to Poincaré >> |
