ANDRE WEIL: Founding Member of the Mathematical Bourbaki Group

Biography

André Weil (1906-1998)

André Weil was a very influential French mathematician around the middle of the 20th Century. Born into a prosperous Jewish family in Paris, he was brother to the well-known philosopher and writer Simone Weil, and both were child prodigies. He was passionately addicted to mathematics by the age of ten, but he also loved to travel and study languages (by the age of sixteen he had read the “Bhagavad Gita” in the original Sanskrit).

He studied (and later taught) in Paris, Rome, Göttingen and elsewhere, as well as at the Aligarh Muslim University in Uttar Pradesh, India, were he further explored what would become a life-long interest in Hinduism and Sanskrit literature.

Even as a young man, Weil made substantial contributions in many areas of mathematics, and was particularly animated by the idea of discovering profound connections between algebraic geometry and number theory. His fascination with Diophantine equations led to his first substantial piece of mathematical research on the theory of algebraic curves. During the 1930s, he introduced the adele ring, a topological ring in algebraic number theory and topological algebra, which is built on the field of rational numbers.

The early leader of the Bourbaki group

Weil was an early leader of the Bourbaki group who published many influential textbooks on modern mathematics

It was also at this time that he became a founding member, and the de facto early leader, of the so-called Bourbaki group of French mathematicians. This influential group published many textbooks on advanced 20th Century mathematics under the assumed name of Nicolas Bourbaki, in an attempt to give a unified description of all mathematics founded on set theory. Bourbaki has the distinction of having been refused membership of the American Mathematical Society for being non-existent (although he was a member of the Mathematical Society of France!)

When the Second World War broke out, Weil, a committed conscientious objector, fled to Finland, where he was mistakenly arrested as a possible spy. Having made his way back to France, he was again arrested and imprisoned as for refusing to report for military service. In his trial, he cited the Bhagavad Gita to justify his stand, arguing that his true dharma was the pursuit of mathematics, not assisting in the war effort, however just the cause. Given the choice of five more years in prison or joining a French combat unit, though, he chose the latter, an especially lucky decision given that the prison was blown up shortly afterwards.

But it was in 1940, in a prison near Rouen, that Weil did the work that really made his reputation (although his full proofs had to wait until 1948, and even more rigorous proofs were supplied by Pierre Deligne in 1973). Building on the prescient work of his countryman Évariste Galois in the previous century, Weil picked up the idea of using geometry to analyze equations, and developed algebraic geometry, a whole new language for understanding solutions to equations.

Weil Conjectures

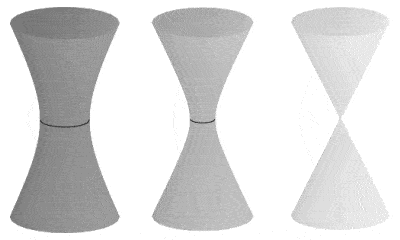An illustration of the “cycle évanescent” or “vanishing cycle” described in Deligne’s proof of the Weil conjectures

The Weil conjectures on local zeta-functions effectively proved the Riemann hypothesis for curves over finite fields, by counting the number of points on algebraic varieties over finite fields. In the process, he introduced for the first time the notion of an abstract algebraic variety and thereby laid the foundations for abstract algebraic geometry and the modern theory of abelian varieties, as well as the theory of modular forms, automorphic functions and automorphic representations. His work on algebraic curves has influenced a wide variety of areas, including some outside of mathematics, such as elementary particle physics and string theory.

In 1941, Weil and his wife took the opportunity to sail for the United States, where they spent the rest of the War and the rest of their lives. In the late 1950s, Weil formulated another important conjecture, this time on Tamagawa numbers, which remained resistant to proof until 1989. He was instrumental in the formulation of the so-called Shimura-Taniyama-Weil conjecture on elliptic curves which was used by Andrew Wiles as a link in the proof of Fermat’s Last Theorem. He also developed the Weil representation, an infinite-dimensional linear representation of theta functions which gave a contemporary framework for understanding the classical theory of quadratic forms.

Over his lifetime, Weil received many honorary memberships, including the London Mathematical Society, the Royal Society of London, the French Academy of Sciences and the American National Academy of Sciences. He remained active as professor emeritus at the Institute for Advanced Studies at Princeton until a few years before his death.