What Do You Learn in 10th Grade Math? Advancing Through Algebra and Geometry

What Do You Learn in 10th Grade Math? 

In 10th grade math, students typically expand their knowledge in geometry, exploring more complex theorems and proofs, and diving deeper into algebra with quadratic equations, functions, and inequalities. They may also be introduced to trigonometry, focusing on the relationships between the angles and sides of triangles.

Introduction

Tenth (10th) grade is a pivotal year in a student’s mathematical journey, where the foundations built in previous years are refined, and advanced concepts are introduced. In this comprehensive guide, we will explore the key concepts and skills that students typically learn in 10th–grade math.

From algebraic mastery to trigonometric functions, we’ll delve into the essential topics and provide practical numerical examples with detailed solutions to illustrate each concept. This in-depth exploration will help students, parents, and educators gain a deeper understanding of the challenging yet rewarding world of 10th–grade mathematics.

Key Concepts and Skills in 10th Grade Math

Algebraic Mastery

Tenth graders delve deep into algebra, honing their skills in equations, inequalities, and functions. Key concepts include:

Quadratic Equations

Solving quadratic equations through factoring, completing the square, and using the quadratic formula. For example, solving the equation 2x² – 5x + 3 = 0.

Systems of Equations

Solving systems of linear equations using various methods such as substitution and elimination. For instance, solving the system:

2x + 3y = 10
4x – y = 5

Polynomial Operations

Performing operations with polynomials, including addition, subtraction, multiplication, and division.

Geometry and Trigonometry

Trigonometric Functions

Introduction to trigonometric functions, including sine, cosine, and tangent. Students learn to apply trigonometry to solve right-triangle problems and explore periodic functions.

Below is an example of a trigonometric function.

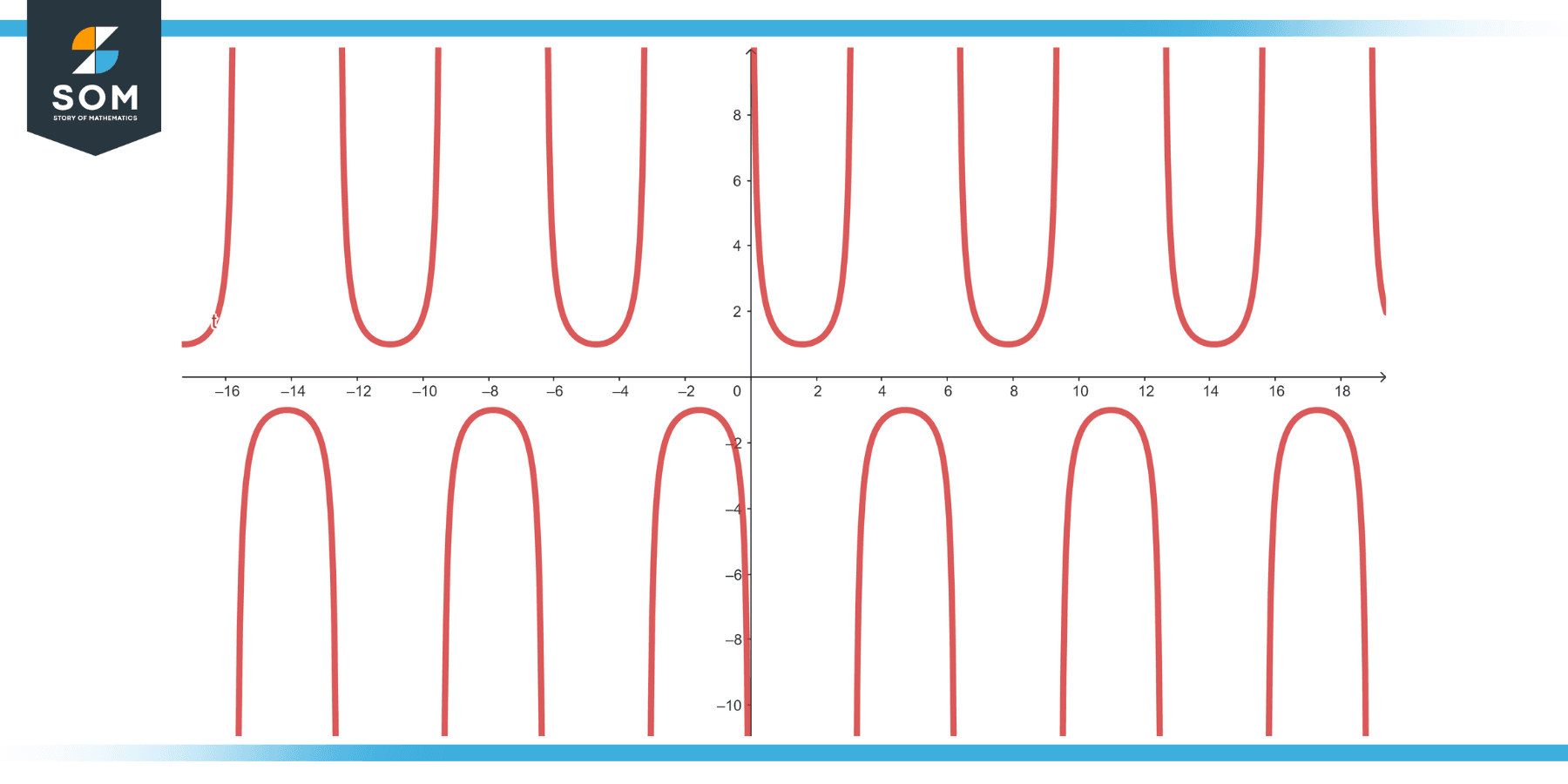
Figure-1: Generic Trigonometric Function

Geometry Proofs

Developing geometric proofs, using deductive reasoning to justify statements about geometric figures.

Advanced Functions and Graphing

Exponential and Logarithmic Functions

Understanding exponential growth and decay, as well as logarithmic functions. Students learn to solve exponential and logarithmic equations.

Graphing Functions

Mastering the graphing of linear, quadratic, exponential, and trigonometric functions, including transformations and inverse functions. Below is an example of a generic function plotted.

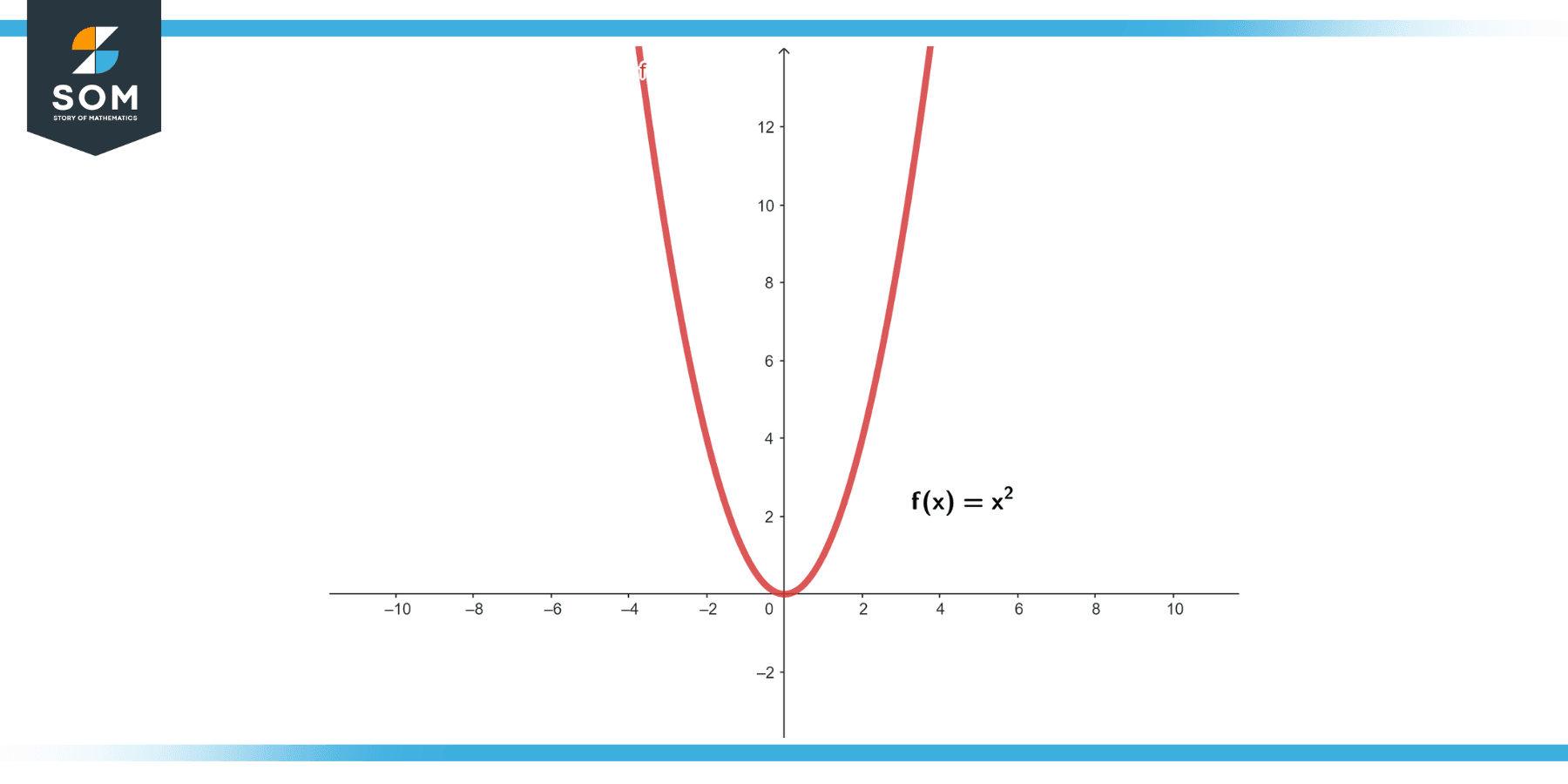
Figure-2: Generic Function

Numerical Examples

Let’s delve into numerical examples to gain a comprehensive understanding of 10th–grade math concepts:

Example 1

Quadratic Equations

Problem: Solve the quadratic equation 2x² – 5x + 3 = 0.

Solution

To solve the quadratic equation, we can use the quadratic formula:

x = (-b ± √(b² – 4ac)) / (2a)

In this case, a = 2, b = -5, and c = 3.

x = (5 ± √((-5)² – 4 * 2 * 3)) / (2 * 2)
x = (5 ± √(25 – 24)) / 4
x = (5 ± √1) / 4

There are two solutions:

x₁ = (5 + 1) / 4 = 6/4 = 3/2
x₂ = (5 – 1) / 4 = 4/4 = 1

So, the solutions are x = 3/2 and x = 1.

Example 2

Trigonometric Functions

Problem: Find the sine of an angle θ in a right triangle, where the opposite side is 4 units and the hypotenuse is 5 units.

Solution

Using the definition of sine: sin(θ) = opposite/hypotenuse

sin(θ) = 4/5

Example 3

Systems of Equations

Problem: Solve the following system of equations.

2x + 3y = 10
4x – y = 5

Solution

We can solve this system using the elimination method. Multiply the second equation by 3 to eliminate y:

Now, add the two equations to eliminate y:

(2x + 3y) + (12x – 3y) = 10 + 15
14x = 25
x = 25/14

Substitute the value of x into the second equation to find y:

4 (25/14) – y = 5
(100/14) – y = 5
– y = 5 – (100/14)
– y = (70/14) – (100/14)
– y = (-30/14)
– y = -15/7

So, the solution is x = 25/14 and y = -15/7.

Example 4

Exponential Function

Problem: Solve for x in the equation $2^{(x-1)}$ = 8.

Solution

Rewrite 8 as a power of 2: 8 = 2³.

Now, we have:

$2^{(x-1)}$ = 2³

Since the bases are the same, the exponents must be equal:

x – 1 = 3
x = 3 + 1
x = 4

So, the solution is x = 4.

Example 5

Polynomial Operations

Problem: Simplify the expression (3x² – 2x + 5) + (2x² + 4x – 1).

Solution

To simplify, combine like terms by adding the coefficients of the same degree terms:

(3x² – 2x + 5) + (2x² + 4x – 1) = (3x² + 2x²) + (-2x + 4x) + (5 – 1)
= 5x² + 2x + 4

Example 6

Geometry Proofs

Problem: Prove that the opposite angles of a parallelogram are congruent.

Solution

Let’s consider a parallelogram ABCD where AB || CD and AD || BC.

We want to prove that ∠A ≅ ∠C and ∠B ≅ ∠D.

In ΔABC and ΔADC:

AD = AD (Common side)

AB || CD and AD || BC (Opposite sides of a parallelogram)

Therefore, by the Alternate Interior Angles Theorem, ∠A ≅ ∠C.

In ΔBCD and ΔBAD:

AB = CD (Opposite sides of a parallelogram)

AD || BC and AB || CD (Opposite sides of a parallelogram)

Therefore, by the Alternate Interior Angles Theorem, ∠B ≅ ∠D.

Hence, we have proven that opposite angles of a parallelogram are congruent.

Example 7

Exponential Growth

Problem: A bacteria colony doubles in size every hour. If there are initially 100 bacteria, how many will there be after 5 hours?

Solution

We can use the formula for exponential growth: N(t) = N₀ * 2^(t/h), where N(t) is the final population, N₀ is the initial population, t is the time in hours, and h is the doubling time.

N(5) = 100 * $2^(5/1)$

N(5) = 100 * $2^5$

N(5) = 100 * 32

N(5) = 3200

After 5 hours, there will be 3200 bacteria in the colony.

Example 8

Trigonometric Identities

Problem: Prove the trigonometric identity: tan²(θ) + 1 = sec²(θ).

Solution

We start with the fundamental trigonometric identity:

sec(θ) = 1/cos(θ)

Square both sides:

sec²(θ) = (1/cos(θ))² = 1/cos²(θ)

Now, we use the Pythagorean identity:

1 + tan²(θ) = sec²(θ)

Substitute the value of sec²(θ):

1 + tan²(θ) = 1/cos²(θ)

Rearrange the equation:

1/cos²(θ) – 1 = tan²(θ)

Common denominator:

(1 – cos²(θ))/cos²(θ) = tan²(θ)

Now, use the Pythagorean identity:

sin²(θ) + cos²(θ) = 1

Solve for 1 – cos²(θ):

1 – cos²(θ) = sin²(θ)

Substitute into the equation: sin²(θ)/cos²(θ) = tan²(θ).

Simplify:

tan²(θ) = tan²(θ)

The identity is proven.

Conclusion

Tenth–grade mathematics is a bridge to more advanced mathematical concepts and problem-solving skills. It equips students with a deep understanding of algebra, geometry, trigonometry, and functions, setting the stage for success in higher-level math courses and future academic pursuits.

The numerical examples provided in this guide offer a glimpse into the types of problems and solutions that 10th graders encounter as they navigate the challenging yet intellectually stimulating world of advanced mathematics.

By mastering the intricacies of quadratic equations, systems of equations, trigonometric functions, and exponential functions, students develop critical thinking and analytical skills that extend far beyond the classroom. These skills empower them to tackle real-world problems, make informed decisions, and contribute to fields that rely on mathematical reasoning.

As 10th graders continue to explore and refine their mathematical abilities, they are well-prepared for the exciting mathematical journey that lies ahead in their academic careers.